MATHEMATICS CONTEST 2003 SOLUTIONS
PART I: 30 Minutes; NO CALCULATORS
Section A. Each correct answer is worth 1 point.
1. Find the sum of the first six prime numbers.
Solution: 2 + 3 + 5 + 7 + 11 + 13 = 41 (the number 1 is not a prime).
2. Give the official ( and more common ) name for a regular
quadrilateral.
Solution: “Regular” means equal sides and equal interior angles, so it’s
a square.
3. Express the repeating decimal
as a ratio of two positive integers in simplest form.
Solution 1: Let x =
;
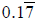then 10x =
,
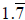and 10x − x = 1.777777 . . . − 0.177777 . . . = 1.6.
If 9x = 1.6, then x = 1.6/9 = 16/90 = 8/45 .
Solution 2: Recall that 7/9 =
,
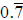so we want 7/9 − 3/5. (Or 1/10 + 7/90, or 1/6 + 1/90 .)
4. If 2x + 1 = 2003, find the value of 3x − 1000.
Solution: Solve for x: If 2x + 1 = 2003, then 2x = 2002, so x = 1001.
Then 3x − 1000 =
3(1001) − 1000 = 2003.
5. A non-isosceles triangle has integral sides of 4, 5,
and x. Find all possible values of x.
Solution: The triangle inequality theorem says that the sum of any two
sides must be greater than
the third side, so 5−4 < x < 4+5, or 1 < x < 9. But the sides are integral (they
are integers),
so x must belong to the set {2, 3, 4, 5, 6, 7, 8}. Additionally, the triangle is
non-isosceles (no
two sides can be equal). Therefore, x is in {2, 3, 6, 7, 8}.
6. Using some or all of the digits 0–9 (no digit more than
once ), construct the largest possible
six-digit odd number with a 9 in the tens place.
Solution:
9 must be in the tens place.
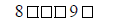Biggest possible number.
Biggest possible number.
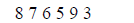Biggest possible number, number must be odd.
7. 7 + (−7) = 0. This is an example of what basic property
of addition ?
Solution: Additive inverse property or Opposites property.
Section B. Each correct answer is worth 2 points.
8. If (x5 + x4 + x − 5) is divided by (x + 1), find the
remainder.
Solution 1 (Long division):
Solution 2 (Synthetic division): Works when
dividing by x −c. Rewrite x +1 as x −(−1), then
write out the coefficients , multiply and add; the remainder is the last number
on the bottom.
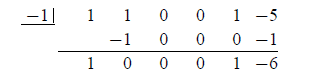
Solution 3 ( Math knowledge ): The remainder theorem
says you can just plug in −1 to get the
remainder: (−1)5 + (−1)4 + (−1) − 5 = −6
9. Find the 2003rd digit after the decimal point in the
decimal representation of 4/7.
Solution: Rational numbers (such as fractions) either stop or repeat. If
you do long division far
enough, the pattern is clear:
4 ÷ 7 = 0.57142857142857... or
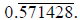
So it repeats every six numbers. Now divide 6 into 2003, and observe that the
remainder is 5,
so we choose the 5th repeated digit: The answer is 2.
10. In the figure on the right, the length of tangent
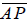is 12,
PD = 8, and chord
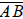bisects chord
.
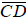If EB = 3, find
the length of
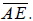
Solution: (AP)(AP) = (PD)(PC), so 122 = (8)(PC),
or PC = 18. Then CD = 10, and because
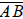bisects
,
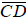CE = ED = 5. Now (AE)(EB) = (CE)(ED), so
(AE)(3) = (5)(5), and we conclude that AE = 25/3 .
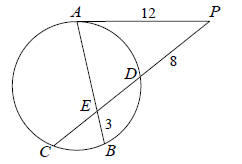
11. Express in simplest radical form (no radicals in the
denominator):
Solution: This is sometimes called “rationalizing the denominator”:
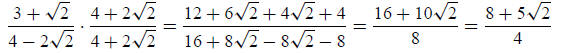
12. Write the exact numerical value of x if log8 128 = x.
Express in simplest form.
Solution 1: Transform to exponential form: 8x = 128. Now rewrite both 8 and 128
as powers of
2: 8 = 23 and 128 = 27, so 8x = (23)x = 23x = 27. Therefore, 3x = 7, or x = 7/3.
Solution 2: Use the change-of-base formula and properties of logarithms:
Section C. Each correct answer is worth 3 points.
13. The ellipse x 2 + 2y2 + 12y − 10x − 57 = 0 has a major axis with two
endpoints. Find the
coordinates of the endpoint that lies in quadrant IV. Express in ordered pair
form, (x, y).
Solution: Put in standard form by completing the squares:
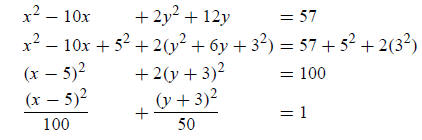
The ellipse is centered at (5,−3), and the major axis is therefore horizontal
with length 2a = 20;
the endpoint in quadrant IV is (5 + 10,−3) or (15,−3).
14. Softball player Berni Williams has 120 hits in 300 at-bats for a current
batting average of .400.
In today’s game, she will have 5 at-bats. What is the probability that she will
get exactly 2 hits?
Solution: The number of hits among her next five at-bats has a binomial
distribution (more on this
below), so P(exactly 2 hits) =
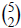(0.4)2(0.6)3, where
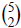is “5 choose 2” (sometimes written
as
),
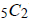which is equal to 10. This evaluates to
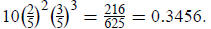
If you have never heard of the binomial distribution, here are two ways to think
about it:
1. Write out every possible sequence of two hits and three outs:
HHooo, HoHoo, HooHo, HoooH, oHHoo, oHoHo, oHooH, ooHHo, ooHoH, oooHH
There are 10 such sequences, and each has probability (0.4)2(0.6)3.
2. The binomial distribution gets its name from the terms in the expansion of
the binomial
(q + p)n, where p is the probability of success (a hit), q = 1 − p is the
probability of failure
(an out), and n is the number of attempts. Thus:
(0.6 + 0.4)5 = 0.65 + 5(0.640.41) + 10(0.630.42) + 10(0.620.43) + 5(0.610.44) + 0.45.
Each term in this sum corresponds to the probability of a certain number of
failures and successes;
we want the third term.
15. Solve the inequality
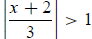and graph its solution on the given number line .
Solution: Either
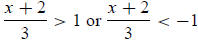, so that x > 1 or x < −5. This can be drawn either
of these ways:
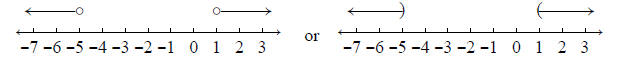
| Prev | Next |