GLOSSARY OF TERMS
| Algorithm. An established step-by-step procedure used | 1 |
| to achieve a desired result. For example, the | 55 |
| addition algorithm for the sum of two two -digit | + 27 |
| numbers where carrying is required: | 82 |
Arbitrary unit (of measure). A unit that is not part of the standardized metric or US Customary systems. For example, using one’s own shoe size to measure the length of a door opening or saying that the area of an exhibition hall floor is “about the size of two football fields.”
Associative property . When adding or multiplying three numbers, it doesn’t matter if the first two or the last two numbers are added or multiplied first. For example,

Attribute (measurable). An identifiable property of an object, set, or event that is subject to being measured. For example, some of the measurable attributes of a box are its length, weight, and capacity (how much it holds).
| Box plot.
A graphic method that shows the
distribution of a set of data by using the median, quartiles, and the extremes of the data set. The box shows the middle 50% of the data; the longer the box, the greater the spread of the data. spread of the data. |
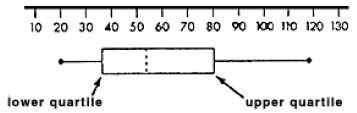 |
Central tendencies. A number which in some way conveys the “center” or “middle” of a set of data. The most frequently used measures are the mean and the median.
Combinations . Subsets chosen from a larger set of objects in which the order of the items in the subset does not matter. For example, determining how many different committees of four persons could be chosen from a set of nine persons. (See also, Permutations)
Commutative property. Numbers can be added or multiplied in either order. For example, 15 + 9 = 9 + 15; 3 x 8 = 8 x 3.
Congruence. The relationship between two objects that have exactly the same size and shape.
Correlation. The amount of positive or negative relationship existing between two measures. For example, if the height and weight of a set of individuals were measured, it could be said that there is a positive correlation between height and weight if the data showed that larger weights tended to be paired with larger heights and smaller weights tended to be paired with smaller heights. The stronger those tendencies, the larger the measure of correlation.
Deciles. The 10th, 20th, 30th, ...90th percentile points (See definition for Percentile.)
Direct measurement. A process of obtaining the measurement of some entity by reading a measuring tool, such as a ruler for length, a scale for weight, or a protractor for angle size.
Dispersion. The scattering of the values of a frequency distribution (of data) from an average.
Distributive property. Property indicating a special way in which multiplication is applied to addition of two (or more) numbers. For example, 5 x 23 = 5 x (20 + 3) = 5 x 20 + 5 x 3 = 100 + 15 = 115.
Expanded notation. Showing place value by multiplying each digit in a number by the appropriate power of 10. For example, 523 = 5 x 100 + 2 x 10 + 3 x 1 or 5 x 102+ 2 x 101+ 3 x 100.
Exponential function . A function that can be represented by an equation of the form y = abx+ c, where a, b, and c are arbitrary, but fixed, numbers and a ≠ 0 and b > 0 and b ≠ 1.
Exponential notation (exponent). A symbolic way of showing how many times a number or variable is used as a factor. In the notation 53, the exponent 3 shows that 5 is a factor used three times; that is 53= 5 x 5 x 5 =125.
Frequency distribution. An organized display of a set of data that shows how often each different piece of data occurs.
Function. A relationship between two sets of numbers or other mathematical objects where each member of the first set is paired with only one member of the second set. Functions can be used to understand how one quantity varies in relation to (is a function of) changes in the second quantity. For example, there is a functional relationship between the price per pound of a particular type of meat and the total amount paid for ten pounds of that type of meat.
Identity. For addition: The number 0; that is N + 0 = N for any number N. For multiplication: The number 1; that is, N x 1 = N for any number N.
Indirect measurement. A process where the measurement of some entity is not obtained by the direct reading of a measuring tool, or by counting of units superimposed alongside or on that entity. For example if the length and width of a rectangle are multiplied to find the area of that rectangle, then the area is an indirect measurement.
Integers. The set of numbers: {..., -6, -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, 6,...}
Intercept . The points where a line drawn on a rectangular-coordinate-system graph intersect the vertical and horizontal axes.
Inverse. For addition: For any number N, its inverse (also called opposite) is a number -N so that N + (-N) = 0 (e.g., the opposite of 5 is -5, the opposite of -3/4 is 3/4). For multiplication: For any number N, its inverse (also called reciprocal) is a number N* so that N x (N*) = 1 (e.g., the reciprocal of 5 is 1/5; the reciprocal of -3/4 is -4/3).
|
Line of best fit. A straight line used as a best approximation of a summary of all the points in a scatter-plot* (See definition below). The position and slope of the line are |
 |
|
Line plot.
|
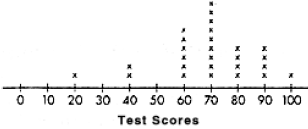 |
Linear equation . An equation of the form y = ax + b, where a and b can be any real number. When the ordered pairs (x, y) that make the equation true for specific assigned values of a and b are graphed, the result is a straight line.
Matrix (pl.: matrices). A rectangular array of numbers, letters, or other entities arranged in rows and columns.
Maximum/minimum ( of a graph ). The highest/lowest point on a graph. A relative maximum/minimum is higher/lower than any other point in its immediate vicinity.
Mean. The arithmetic average of a set of numerical data.
Median. The middle value of an ordered set of numerical data. For example, the median value of the set {5, 8, 9, 10, 11, 11,13} is 10.
Mode. The most frequently occurring value in a set of data. For example, the mode of the set {13, 5, 9, 11, 11, 8, 10} is 11.
Model (mathematical). A [verb] and a noun. [Generate] a mathematical representation (e.g., number, graph, matrix, equation(s), geometric figure) for real world or mathematical objects, properties, actions, or relationships.
(Non)-Linear functional relationship. (See definition of Function above.) Many functions can be represented by pairs of numbers. When the graph of those pairs results in points lying on a straight line, a function is said to be linear. When not on a line, the function is nonlinear.
Outlier. For a set of numerical data, any value that is markedly smaller or larger than other values. For example, in the data set {3, 5, 4, 4, 6, 2, 25, 5, 6, 2} the value of 25 is an outlier.
Patterns. Recognizable regularities in situations such as in nature, shapes, events, sets of numbers. For example, spirals on a pineapple, snowflakes, geometric designs on quilts or wallpaper, the number sequence {0, 4, 8, 12, 16,...}.
Percentile. A value on a scale that indicates the percent of a distribution that is equal to it or below it. For example, a score at the 95th percentile is equal to or better than 95 percent of the scores.
Permutations. Possible arrangements of a set of objects in which the order of the arrangement makes a difference. For example, determining all the different ways five books can be arranged in order on a shelf.
Prime number. A whole number greater than 1 that can be divided exactly (i.e., with no remainder) only by itself and 1. The first few primes are 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37.
Pythagorean theorem (relationship). In a right triangle, c2= a2+ b2, where c represents the length of the hypotenuse (the longest side of the triangle which is opposite the right (angle), and a and b represent the lengths of the other two, shorter sides of the triangle.
Quadratic function. A function that can be represented by an equation of the form y = ax2 (or ax^2) + bx + c, where a, b, and c are arbitrary, but fixed, numbers and a 0. The graph of this function is a parabola.
Quartiles. The 25th, 50th and 75th percentile points. (See definition of Percentile.)
Range (of a set of data). The numerical difference between the largest and smallest values in a set of data.
Rational number . A number that can be expressed as the ratio, or quotient, of two integers, a/b, provided b≠ 0. Rational numbers can be expressed as common fractions or decimals, such as 3/5 or 0.6. Finite decimals, repeating decimals, mixed numbers and whole numbers are all rational numbers. Nonrepeating decimals cannot be expressed in this way, and are said to be irrational.
Real numbers. All the numbers which can be expressed as decimals.
Real-world problems. Quantitative and spatial problems that arise from a wide variety of human experiences, applications to careers. These do not have to be highly complex ones and can include such things as making change, figuring sale prices, or comparing payment plans.
Rectangular coordinate system . This system uses two (for a plane) or three (for space) mutually perpendicular lines (called coordinate axes) and their point of intersection (called the origin) as the frame of reference. Specific locations are described by ordered pairs or triples (called coordinates) that indicate distance from the origin along lines that are parallel to the coordinate axes.
Scaling ( Scale drawing ). The process of drawing a figure either enlarged or reduced in size from its original size. Usually the scale is given, as on a map 1 inch equals 10 miles.
Scatter plot. Also
known as scattergram or scatter diagram. A two dimensional graph representing a
set of bi-variate data. That is, for each element being graphed, there are two
separate pieces of data. For example, the height and weight of a group of 10
teenagers would result in a scatter plot of 10 separate points on the graph.

Scientific notation. A short-hand way of writing very large or very small numbers. The notation consists of a decimal number between 1 and 10 multiplied by an integral power of 10. For example, 47,300 = 4.73 x4; 0.000000021 = 2.1 x 10
-8Similarity. The relationship between two objects that have exactly the same shape but not necessarily the same size.
Simulation. Carrying out extensive data collection with a simple, safe, inexpensive, easy-toduplicate event that has essentially the same characteristics as another event which is of actual interest to an investigator. For example, suppose one wanted to gather data about the actual order of birth of boys and girls in families with five children. (e.g., BBGBG is one possibility) Rather than wait for five children to be born to a single family, or identifying families that already have five children, one could simulate births by repeatedly tossing a coin five times. Heads vs. tails has about the same chance of happening as a boy vs. a girl being born.
Slope. A measure of the steepness or incline of a straight line drawn on a rectangularcoordinate- system graph. The measure is obtained by the quotient “rise/run” (vertical change divided by horizontal change) between any two points on that line.
|
Stem-and-leaf plot. A way of |
1|3699 2|268 3|344 |
| Key: 1|5 means 15 | |
Summary statistics. A single number representation of the characteristics of a set of data. Usually given by measures of central tendency and measures of dispersion (spread).
Symmetry. A figure has symmetry if it has parts that correspond with each other in terms of size, form, and arrangement. For example, a figure with line (or mirror) symmetry has two halves which match each other perfectly if the figure is folded along its line of symmetry.
Transformation. A change in the size, shape, location or orientation of a figure.
Transitive property. For equality: If a=b and b=c, then a=c; For inequality: If a>b and b>c, then a>c; or If a<b and b<c, then a<c.
|
Tree diagram. A schematic way of showing the number ofways a compound event may occur. For example, the tree diagram at the right shows the eight possible ways the tossing of three coins could happen. Unit fraction. A fraction with a numerator of 1, such as1/4 or 1/7. |
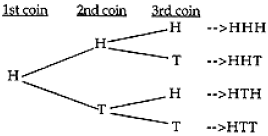 |
|
Variable. y = 2x + 7, both x and y are variables. |
 |
| Variance. The value of the standard deviation squared. | |
|
Vertical angles. The pair of angles that are
directly across from each
|
 |
| Whole numbers. The numbers: 0, 1, 2, 3, 4, 5, ... |
| Prev | Next |