Review Guide for Math 117 test 2
Unit 2 Test during lecture #11. Please seat yourself every
other seat. You will need your graphing
calculator. The test covers these functions:
| Polynomial | Exponential | Rational | Trig |
| Linear Quadratic Direct Variation |
Exponential | Inverse Variation | sine cosine |
The test also covers regression on the TI-83 (finding a
model to fit data), solving equations for x
or y , and other keystrokes we have discussed, such as the recursive feature of
the calculator.
The test will be given in 2 parts:
Part I: withOUT a calculator, identify functions from tables,
graphs, and equations; Determine linear and inverse functions from
information given; general questions about properties of functions studies;
distinguish inverse variations from inverses of functions; match equations to
their graphs without using a graphing calculator.
Part II: WITH graphing calculator, find specific functions in
word problems using stat regression, and use the function to find specific
answers for the problem.
You will complete and turn in Part I (no calculator) then get Part II.
You will receive last semester’s test for a practice test. Do not assume your
test will be a “clone” of
last semester’s test. Review lecture notes, lab activities, homework, and
assigned pages in the text.
SAMPLE TEST for practice
DIRECTIONS: Part I is to be done without a calculator. When you finish part I,
turn it in
and receive part II which requires a calculator. You have a maximum of 2 hours
to complete
both parts. (Each of the following problems worth 2 points)
For each of the following problems, select the best choice for the function described as:
a) linear b ) quadratic c) exponential d) inverse variation e) other

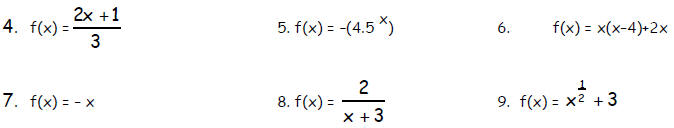
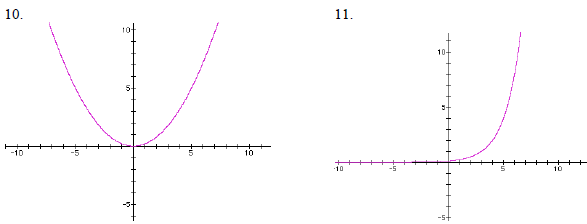
12. Write the point-slope equation of the line which contains the two points (1,5) & (3,9).
13. Write the vertex form of the quadratic equation where a=1 and vertex is (–3,4).
14. Gear ratios are used in industry. A gear ratio is the comparison of the
number of teeth
on two gears. When two gears are meshed, the revolutions per minute (rpm) are
inversely
proportional to the number of teeth . Suppose a gear revolves at 200 rpm and has
60 teeth.
Write a specific model for gear ratios.
15. Two scoops of ice cream sell for 90¢/scoop plus 25¢ for the cone. Write a
specific model
for buying x scoops of ice cream.
16. There are approximately 2 lb. of muscle for every 5 lb. of body weight. Name
and write a
specific model relating muscle to body weight.
17. What function is graphed? (circle your choice)
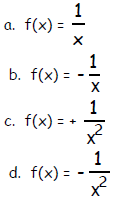
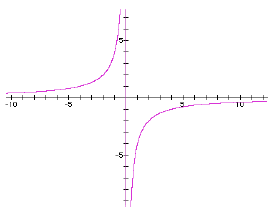
18. Given f(x) = x – 3, what is the inverse of f(x) or f-1(x)? (Show work)
Matching: Match the function on the left with its graph on the right.
19. f(x) = 2(x+1)+3
20. f(x) = -2(x+2)2+3
21. f(x) = 2(x–2)2–3
22. f(x) = 2x
23. f(x) = 2-x
24. f(x) = x-1
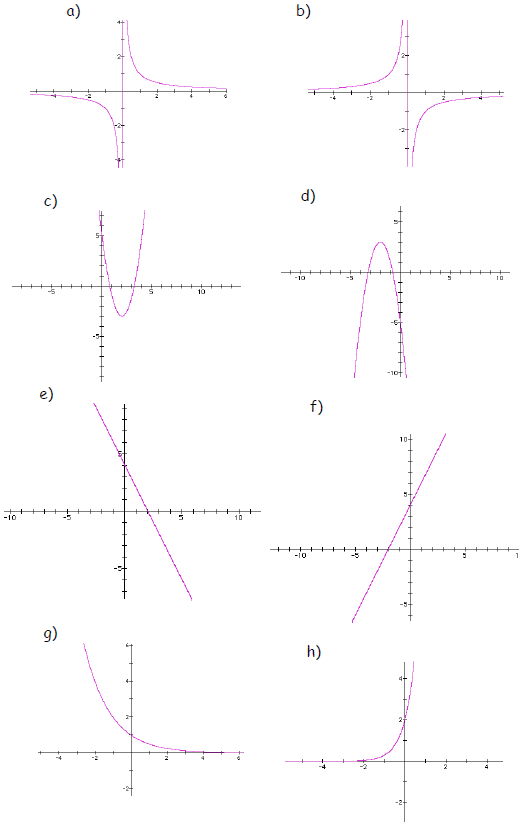
25. About what line(s) is the graph of f(x) = x2 symmetric?
DIRECTIONS: Part II requires a graphing calculator. If the problem requires
(asks for) an
explanation or showing work, you will earn only partial credit for an answer
without work.
(Each of the following problems worth 2 points unless stated otherwise.)
DCD Inc. is a mail order business that sells the latest CD's at rock bottom
prices. All of
their CD's are one price, $7.95 each, with a $4 shipping and handling fee for
each order.
26. Make a table showing the cost of 1 to 6 CD's.
Label the table columns using "#CD's" and "cost".
27. Write the function for the DCD problem.
The percentage of American students in grades 9-12 using a computer at home is
shown in the
following table, for different family incomes (recorded as median income
levels).
| Family income |
$7500 | $12500 | $17500 | $22500 | $27500 | $32500 | $7500 |
| % using computers |
4 | 7 | 14 | 14 | 17 | 20 | 25 |
28. Find a linear model for the data.
29. Find a quadratic model for the data.
30. Find an exponential model for the data.
31. Which model best fits the data? Briefly justify your choice.
32. Approximately what would be the family income if 40% of students in grades
9-12 use a
computer at home, according to the model you chose? (show how you got your
answer)
The intensity of light reaching you from a light bulb depends on how far from
the bulb you are
standing, i.e., the intensity (I) varies inversely as the square of the distance
(d). Suppose that at 3
meters, the intensity is 120 units, and at 6 meters it is 30 units.
(note: this was the "Light From a Distance lab activity)
33. State the specific equation for this problem.
34. Predict the intensity at 10 meters.
35. Predict the distance at which the intensity will be 4 units.
A radioactive atom "decays" by shooting out something from its nucleus. Once the
"thing" is shot
out, the atom is no longer radioactive. Radioactive iodine, used to examine
people's thyroid glands,
decays with a "half-life" of 8.1 days. (This means that when 8.1 days is added
to the time , the
amount of iodine remaining is multiplied by 1/2).
36. What kind of function would be an appropriate mathematical model relating
the amount of
iodine remaining to the time that has passed? Briefly explain your choice.
37. A hospital receives a shipment of 23.7 millicuries of radioactive iodine
(consider this
day 0). Write the specific equation expressing the amount of iodine remaining as
a function of
the number of days since the hospital received the iodine.
38. Assume that the hospital uses none of the radioactive iodine on patients.
Use your equation to
predict the amount of iodine remaining after 1 week.
39. Assuming again that the hospital uses none of the radioactive iodine on
patients. Use your
equation to find the number of days until the amount of iodine has dropped to
0.8 millicuries.
In science lab, Wanda measures the following values of x and y .
| x | 11 | 18 | 23 |
| y | 394 | 807 | 1162 |
40. Wanda wants to know whether the function could be linear. Tell her the
answer, and how you
decided.
41. If it is linear, state the specific equation. If it is not linear, assume it
is quadratic and
find the specific equation.
Write the general equation for the function that has the following property.
42. Doubling x doubles y.____________
43. Doubling x makes y half as big._____________
44. When a constant is added to x, the value of y is multiplied by a constant._________________
45. The product of x and y is constant.____________
46. When a constant is added to x, a constant is added to y._____________
47. The value of y is obtained by raising each value of x to the second power .____________
Give the calculator strokes for: (worth 3 points each)
48. Creating an arithmetic sequence 1, 3, 5, 7… using the recursive feature of
the calculator.
49. Evaluating the function (other than direct substitution ) for more than one
given value of x
(such as x = 14 & 15) after you enter a function (for example, y1 = 2x + 7).
| Prev | Next |