COLLEGEWIDE COURSE SYLLABUS FOR PRECALCULUS
|
Prerequisites: |
Required math score on College Placement Test, MAT 075, or appropriate transfer credit course |
| Corequisites: | None |
| Course Hours and Credits: | 4:0:4 |
| . | |
| Course Description: |
This course is designed to integrate intermediate algebra, analytic geometry, and trigonometry with other college algebra topics through a functional approach as a preparation for calculus |
|
Required Text: |
Beecher, J., Penna,J., & Bittinger, M. (2005) Precalculus, (2nd ed.) Boston: Pearson, Addison Wesley |
| Materials: |
Graphing calculator is required for this course: TI-83/84 TI-85/86 TI-89/92 |
| Method of Instruction : | Lecture |
| Manuals: | None |
| Disclaimer: | None |
CORE COURSE PERFORMANCE OBJECTIVES
The student will be able to:
1. Perform basic operations of intermediate algebra. (CCC 2, 7)
2. Use functional definitions and the algebra of functions to solve
applications
of linear and quadratic functions (CCC 2, 7)
3. Solve problems involving polynomial , exponential and logarithmic
functions. (CCC
2, 7)
4. Solve problems of applications involving circular and trigonometric
functions.
5. Solve trigonometric application problems using right triangle definitions
and oblique triangle principles. (CCC 2, 7)
6. Solve problems of applications involving vectors. (CCC 2, 7)
7. Perform basic operations on complex numbers (CCC 2,7)
8. Demonstrate principles of analytic geometry working with conic sections.
(CCC
2, 7)
9. Solve linear and nonlinear systems (CCC 6,7)
10. Perform operations on matrices (CCC6,7)
MEASURABLE PERFORMANCE OBJECTIVES
1. Perform basic operations of intermediate algebra. (CCC 2, 7)
1.1 Perform basic operations on the set of real numbers, including order
of operations, absolute value , and fractions.
1.2 Perform basic operations on polynomials, including addition,
subtraction,
multiplication and evaluation, and division by a
monomial .
1.3 Perform basic operations with integer exponents.
1.4 Demonstrate your ability to factor polynomials.
1.5 Demonstrate your ability to work with rational expressions by adding,
subtracting, multiplying, and dividing the given expressions.
1.6 Simplify complex fractions .
1.7 Write radicals in simplest radical form.
1.8 Demonstrate your ability to rationalize the numerator or the
denominator of
a radical expression.
1.9 Solve first degree equations in one unknown .
1.10 Solve first degree equations involving absolute
value.
1.11 Solve second degree equations by factoring, quadratic formula, and
completing the square.
1.12 Solve radical equations.
1.13 Demonstrate your ability to solve applications of equations.
1.14 Solve inequalities.
1.15 Solve inequalities involving absolute values.
2. Use functional definitions and the algebra of functions to solve
applications
of linear and quadratic functions (CCC 2, 7)
2.1 Define a function and a relation.
2.2 Evaluate a function.
2.3 Find the domain and range of a function.
2.4 Use the vertical line rule to classify relations as functions.
2.5 Solve applications of function problems.
2.6 Find the slope of a nonvertical line
2.7 Write the equation of a line using point-slope or slope- intercept form
of a
line.
2.8 Draw the graph of a linear equation.
2.9 Write the equation of a line perpendicular or parallel to a given line or
segment.
2.10 Solve applications of linear functions.
2.11 Put a quadratic equation of a parabola in standard form.
2.12 Sketch the graph of a parabola.
2.13 Graph and identify functions in the form: .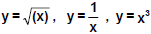
2.14 Solve applications of quadratic functions.
2.15 Use additional graphing techniques to graph quadratic
functions
2.16 Given functions f(x) and g(x) find f + g, f - g, fg, f o g.
2.17 Find the domain of the composition of functions.
2.18 Find the inverse of a function.
2.19 Solve variation problems.
3. Solve problems involving polynomial, exponential and logarithmic
functions.
(CCC 2, 7)
3.1 Given two polynomials, divide them using long division.
3.2 Given two polynomials, divide them using synthetic division.
3.3 Use the Remainder and Factor Theorem.
3.4 Use Descartes’ Rule of Signs.
3.5 Graph a function of the form 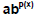
3.6 Change an expression in the form 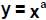 to the form
to the form 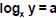
3.7 Solve logarithmic equations .
3.8 Solve exponential equations.
3.9 Solve applications of exponential and logarithmic functions.
4. Solve problems of applications involving circular and trigonometric
functions
(CCC 2, 7)
4.1 Change an angle in decimal form to degrees minutes and seconds.
4.2 Change an angle in degrees minutes and seconds to decimal
degrees.
4.3 Convert an angle in degrees to radian.
4.4 Convert an angle in radian to degrees.
4.5 Graph an angle in standard position.
4.6 Use the formula 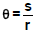 to solve for the indicated parts.
to solve for the indicated parts.
4.7 Define the six circular functions in terms of a unit
circle and a right
triangle.
4.8 Sketch the curves of six circular functions, and identify the
amplitude,
period, and phase shift.
5. Solve trigonometric application problems using right triangle
definitions and
oblique triangle principles. (CCC 2, 7)
5.1 Use notational conventions in trigonometry.
5.2 Memorize basic right-triangle identities.
5.3 Simplify trigonometric expressions.
5.4 Verify that a given trigonometric expression is an identity.
5.5 Solve trigonometric equations.
5.6 Solve applications of trigonometric equations.
5.4 Solve for the indicated parts of a right triangle.
5.5 Solve application problems involving right triangles.
5.6 Develop the laws of sines and cosines and use them to solve
applications
problems involving oblique triangles.
5.7 Solve non-right triangles for the indicated part, using the Law of
Sines.
5.8 Solve non-right triangles for the indicated parts, using the Law of
Cosines.
6. Solve problems of applications involving vectors. (CCC 2, 7)
6.1 Sketch the graphs of sine and cosine functions considering their
period,
amplitude and phase shift.
6.2 Solve application problems involving simple harmonic motion.
6.3 Graph the tangent and reciprocal functions.
6.4 Define and illustrate vectors in a plane using a geometric approach.
6.5 Define and use vectors in the plane using an algebraic approach.
6.6 Define a vector and be able to find its i, j components.
6.7 Solve vector problems.
7. Perform basic operations on complex numbers (CCC 2,7)
7.1 Define complex numbers and the imaginary unit, real and imaginary
parts.
7.2 Determine equality of complex number and find the complex
conjugate of
complex numbers.
7.3 Perform the basic operations of addition, subtraction, multiplication,
and
division of complex numbers.
7.4 Change a given complex number to trigonometric form.
7.5 Use trigonometric or polar forms of complex numbers and
DeMoivre’s Theorem
to multiply and divide, and find powers and
roots of complex numbers.
7.6 Use DeMoivre's Theorem to raise a complex number to a power.
7.7 Use DeMoivre's Theorem to find the roots of a complex number.
7.8 Explore the roots of unity.
8. Demonstrate principles of analytic geometry working with conic
sections. (CCC
2, 7)
8.1 State the definition of the four conics.
8.2 Sketch the graph of a given equation of a circle.
8.3 Given information about a circle write the equation.
8.4 Sketch the graph of a given equation of a parabola.
8.5 Given information about a parabola write the equation.
8.6 Sketch the graph of a given ellipse.
8.7 Given information about an ellipse write the equation.
8.8 Sketch the graph of a hyperbola.
8.9 Given information about a hyperbola write the equation.
8.10 Solve application problems involving conics.
9. Solve linear and nonlinear systems (CCC 6,7)
9.1 Solve linear systems of equations using the augmented matrix and
elementary
row operations.
9.2 Use Cramer’s Rule to solve a linear system of equations.
9.3 Solve nonlinear systems of equations.
9.4 Solve linear programming problems using systems of linear
inequalities.
10. Perform operations on matrices (CCC6,7)
10.1 Define equality of matrices.
10.2 Perform matrix operations of addition, subtraction, multiplication, and
the
multiplication of two matrices.
10.3 Find the inverse of a square matrix.
10.4 Use the inverse matrix and a graphing calculator to solve a linear
systems
of equations.
10.5 Find the determinant of a square matrix.
EVALUATION CRITERIA
Students will demonstrate proficiency on all Measurable Performance Objectives
at least to the 75% level. The grade will be determined using the College
Grading
System:
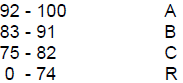
Students should refer to the Student Handbook for
information on
Academic Standing Policy, Academic Honesty Policy, Students
Rights and
Responsibilities and other policies relevant to their academic
progress.
| Prev | Next |