ALGEBRA STRAND
| GRADE | GPS | Comments |
| 3RD | M3A1 Students will use
mathematical expressions to represent relationships between quantities and interpret given expressions. a. Describe and extend numeric and geometric patterns. b. Describe and explain a quantitative relationship represented by a formula (such as the perimeter of a geometric figure). c. Use a symbol, such as  and and
 , to represent an unknown and find
the , to represent an unknown and find
thevalue of the unknown in a number sentence. |
|
| 4TH | M4A1 Students will represent and
interpret mathematical relationships in quantitative expressions. a. Understand and apply patterns and rules to describe relationships and solve problems. b. Represent unknowns using symbols, such as  and and
 c. Write and evaluate mathematical expressions using symbols and different values. |
|
| 5TH | M5A1 Students will represent and
interpret the relationships between quantities algebraically. a. Use variables, such as n or x, for unknown quantities in algebraic expressions. b. Investigate simple algebraic expressions by substituting numbers for the unknown. c. Determine that a formula will be reliable regardless of the type of number (whole numbers or decimal fractions) substituted for the variable . |
|
| 6TH | M6A1 Students will understand the
concept of ratio and use it to represent quantitative relationships. M6A2 Students will consider relationships between varying quantities. a. Analyze and describe patterns arising from mathematical rules, tables, and graphs. b. Use manipulatives or draw pictures to solve problems involving proportional relationships . c. Use proportions (a/b = c/d) to describe relationships and solve problems, including percent problems. d. Describe proportional relationships mathematically using y = kx, where k is the constant of proportionality. e. Graph proportional relationships in the form y = kx and describe characteristics of the graphs. f. In a proportional relationship expressed as y = kx, solve for one quantity given values of the other two. Given quantities may be whole numbers, decimals, or fractions. Solve problems using the relationship y = kx. g. Use proportional reasoning (a/b = c/d and y = kx) to solve problems. M6A3 Students will evaluate algebraic expressions, including those with exponents, and solve simple one-step equations using each of the four basic operations. |
|
| 7TH | M7A1 Students will represent and
evaluate quantities using algebraic expressions. a. Translate verbal phrases to algebraic expressions. b. Simplify and evaluate algebraic expressions, using commutative, associative, and distributive properties as appropriate . c. Add and subtract linear expressions . M7A2 Students will understand and apply linear equations in one variable. a. Given a problem, define a variable, write an equation, solve the equation, and interpret the solution. b. Use the addition and multiplication properties of equality to solve one- and two-step linear equations. M7A3 Students will understand relationships between two variables. a. Plot points on a coordinate plane. b. Represent, describe, and analyze relations from tables, graphs, and formulas. c. Describe how change in one variable affects the other variable. d. Describe patterns in the graphs of proportional relationships, both direct (y = kx) and inverse (y = k/x). |
|
| 8TH | M8A1 Students will use algebra to
represent, analyze, and solve problems. a. Represent a given situation using algebraic expressions or equations in one variable. b. Simplify and evaluate algebraic expressions. c. Solve algebraic equations in one variable, including equations involving absolute values. d. Solve equations involving several variables for one variable in terms of the others. e. Interpret solutions in problem contexts. M8A2 Students will understand and graph inequalities in one variable . a. Represent a given situation using an inequality in one variable. b. Use the properties of inequality to solve inequalities. c. Graph the solution of an inequality on a number line. d. Interpret solutions in problem contexts. M8A3 Students will understand relations and linear functions. a. Recognize a relation as a correspondence between varying quantities. b. Recognize a function as a correspondence between inputs and outputs where the output for each input must be unique. c. Distinguish between relations that are functions and those that are not functions. d. Recognize functions in a variety of representations and a variety of contexts. e. Use tables to describe sequences recursively and with a formula in closed form. f. Understand and recognize arithmetic sequences as linear functions with whole-number input values. h. Interpret the constant difference in an arithmetic sequence as the slope of the associated linear function. i. Identify relations and functions as linear or nonlinear. j. Translate among verbal, tabular, graphic, and algebraic representations of functions. M8A4 Students will graph and analyze graphs of linear equations and inequalities. a. Interpret slope as a rate of change. b. Determine the meaning of the slope and y- intercept in a given situation. c. Graph equations of the form y = mx + b. d. Graph equations of the form ax + by = c. e. Graph the solution set of a linear inequality, identifying whether the solution set is an open or a closed half-plane. f. Determine the equation of a line given a graph, numerical information that defines the line, or a context involving a linear relationship. g. Solve problems involving linear relationships. M8A5 Students will understand systems of linear equations and inequalities and use them to solve problems. a. Given a problem context, write an appropriate system of linear equations or inequalities. b. Solve systems of equations graphically and algebraically, using technology as appropriate. c. Graph the solution set of a system of linear inequalities in two variables. d. Interpret solutions in problem contexts. |
|
| 9TH | MM1A1. Students will explore and
interpret the characteristics of functions, using graphs, tables, and simple algebraic techniques. a. Represent functions using function notation. b. Graph the basic functions  , where
n = 1 to 3, , where
n = 1 to 3, 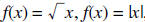 , ,and f(x) = 1/x. c. Graph transformations of basic functions including vertical shifts, stretches, and shrinks, as well as reflections across the x- and y-axes. d. Investigate and explain the characteristics of a function: domain, range, zeros , intercepts, intervals of increase and decrease, maximum and minimum values, and end behavior. e. Relate to a given context the characteristics of a function, and use graphs and tables to investigate its behavior. f. Recognize sequences as functions with domains that are whole numbers. g. Explore rates of change, comparing constant rates of change (i.e., slope) versus variable rates of change. Compare rates of change of linear, quadratic, square root, and other function families. h. Determine graphically and algebraically whether a function has symmetry and whether it is even, odd, or neither. i. Understand that any equation in x can be interpreted as the equation f(x) = g(x), and interpret the solutions of the equation as the x-value(s) of the intersection point(s) of the graphs of y = f(x) and y = g(x). MM1A2. Students will simplify and operate with radical expressions , polynomials, and rational expressions. a. Simplify algebraic and numeric expressions involving square root. b. Perform operations with square roots. c. Add, subtract, multiply, and divide polynomials . d. Expand binomials using the Binomial Theorem. e. Add, subtract, multiply, and divide rational expressions. f. Factor expressions by greatest common factor, grouping , trial and error, and special products limited to the formulas below.  g. Use area and volume models for polynomial arithmetic. MM1A3. Students will solve simple equations. a. Solve quadratic equations in the form 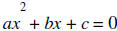 , where a = 1, , where a = 1,by using factorization and finding square roots where applicable. b. Solve equations involving radicals such as  , using , usingalgebraic techniques. c. Use a variety of techniques, including technology, tables, and graphs to solve equations resulting from the investigation of 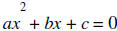 . .d. Solve simple rational equations that result in linear equations or quadratic equations with leading coefficient of 1. |
|
| 10TH | MM2A1. Students will investigate
step and piecewise functions, including greatest integer and absolute value functions. a. Write absolute value functions as piecewise functions. b. Investigate and explain characteristics of a variety of piecewise functions including domain, range, vertex, axis of symmetry, zeros, intercepts, extrema, points of discontinuity, intervals over which the function is constant, intervals of increase and decrease, and rates of change. c. Solve absolute value equations and inequalities analytically, graphically, and by using appropriate technology. MM2A2. Students will explore exponential functions. a. Extend properties of exponents to include all integer exponents. b. Investigate and explain characteristics of exponential functions, including domain and range, asymptotes, zeros, intercepts, intervals of increase and decrease, rates of change, and end behavior. c. Graph functions as transformations of 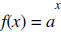 d. Solve simple exponential equations and inequalities analytically, graphically, and by using appropriate technology. e. Understand and use basic exponential functions as models of real phenomena. f. Understand and recognize geometric sequences as exponential functions with domains that are whole numbers. g. Interpret the constant ratio in a geometric sequence as the base of the associated exponential function. MM2A3. Students will analyze quadratic functions in the forms 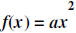 + bx + c and 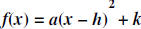 . .a. Convert between standard and vertex form . b. Graph quadratic functions as transformations of the function f(x) = 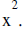 c. Investigate and explain characteristics of quadratic functions, including domain, range, vertex, axis of symmetry, zeros, intercepts, extrema, intervals of increase and decrease, and rates of change. d. Explore arithmetic series and various ways of computing their sums. e. Explore sequences of partial sums of arithmetic series as examples of quadratic functions. MM2A4. Students will solve quadratic equations and inequalities in one variable. a. Solve equations graphically using appropriate technology. b. Find real and complex solutions of equations by factoring, taking square roots, and applying the quadratic formula. c. Analyze the nature of roots using technology and using the discriminant. d. Solve quadratic inequalities both graphically and algebraically, and describe the solutions using linear inequalities MM2A5. Students will explore inverses of functions. a. Discuss the characteristics of functions and their inverses, including one-to-oneness, domain, and range. b. Determine inverses of linear, quadratic, and power functions and functions of the form f(x) = a/x, including the use of restricted domains. c. Explore the graphs of functions and their inverses. d. Use composition to verify that functions are inverses of each other. |
|
| Prev | Next |