Basic Algebra Midterm - Practice Questions
Note: These problems are only intended to help you study. Questions
based an any material covered in
class may appear on the actual test.
1. True or false?
(a) The set of all positive real numbers is a group under multiplication?
(b) For all a, b ∈G, where G is a group, (ab)3 = a3b3.
(c) If α,β are disjoint
cycles in S7 then
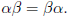
(d) Every transposition is an even permutation.
(e) Every subgroup of Sn has order dividing n!.
(f) Let G be a group and H ≤G, K≤G cyclic subgroups. Then the intersection H
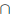K
is a cyclic
subgroup.
(g) Any two groups of order 17 are isomorphic .
(h) Any two groups of order 24 are isomorphic .
2. Let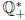 
be the set of
strictly positive rational numbers . Show that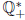 
forms a group with the binary
law given by multiplication . Is this group cyclic?
3. Find the signature sgn (α) and α-1, where:
4. What is th order of each of the following permutations?
5. Let:
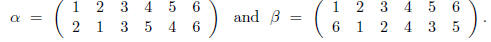
Compute each of the following:
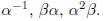
6. Suppose that G is a finite commutative group and that G
has no element of order two . Show that the
map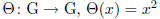 
is a group isomorphism of G to itself.
Will this statement still hold if G is
infinite?
7. Let G be a group. Prove that the function
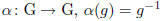is a group isomorphism if and only
if G is commutative.
8. Let G be a group for which x^2 = e for any x ∈G. Prove that G must be commutative.
9. Let G be a finite group. Show that the number of elements x ∈G such that x^3 = e is odd
10. For each of the groups in the following list, describe all the proper subgroups.
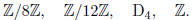
11. Let G be a group and x ∈G. If
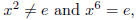what is the order of x?
12. List all the elements of Z/40Z that have order 10.
13. Let G be the set of all polynomials of the form ax^2 +
bx + c, with coefficients from the set {0, 1, 2}.
One can make G a group under addition , by adding polynomials in the usual way,
except that one uses
remainders modulo 3 to combine the coefficients . What is the order of this
group? Is G cyclic?
15. Let H≤S3, given by H = {e, (12)}. Is H a normal subgroup of S3?
16. Prove that An (the set of even permutations) is a normal subgroup of Sn.
| Prev | Next |