Linear Equations and Absolute Value Equations

Review: Solve

1. 9x +13 = -14

2. 3(2x + 7) - 4x = 5x - 6

3. 3x -2(4x - 5) = 2x - 11

4. -7x - 10 = 2(3x-7) - 9x

5. 5x - 7 = 6(x + 2) - x

6. (2x + 1) - (5 - 3x) = 2(3x - 2) - x

Absolute Value Equations
Solve .

1. |x| = 7

2. |3x + 8| = 5

3. |2x - 3| = 7

4. |x +2| - 5 = -1

5. |x - 3| + 7 = 15

6. -2|x +1| = -8

7. 4|2x - 5| - 9 = 7

8. |7x-1| = -8 [Be Careful!]

9. |2x + 3| + 8 = 2

Homework: pg. 502 #11, 17, 19, 23, 27, 29, 31, 33, 45, 47, 49, 53, 59, 6

Review: Solve , Graph, and Write in Interval Notation

1. x > 5

2. 2x + 5 - 9

3. 5 - 2x > -5

4. -3 < 2x - 1 < 5

5. -10 < 4 - 2x - 16

6. x + 3 < -3 OR 2x + 5 > 14

7. 2x + 5 - 9 OR x - 7 > 1

Absolute Value Inequalities : Solve, Graph, and Write in Interval Notation
Less Than:

1. |x| - 3

2. |x| < 5

3. |x + 3| - 1

4. |2x - 1| + 4 < 9

5. |x + 1| - 3 < 8

6. 2|x - 1| - 7 - 15

7. |x+7| + 9 - 3 [Be Careful!]

Greater Than:

1. |x| ≥ 5

2. |x| > 9

3. |x - 2| ≥ 4

4. |2x + 7| + 5 > 10

5. 3|x - 2| + 1 ≥ 10

6. 2|7x-13| +7 > 1 [Be Careful!]

Homework: pg. 512 #7, 9, 11, 15, 19, 23, 27, 29, 33, 35, 37, 39, 41, 45, 49, 53

Graphing Linear Equations and Linear Functions; Graphing Absolute Value Functions

Examples:

1. Find the intercepts and graph .
y = -2x + 6

2. Graph the line using the slope and the y-intercept.

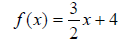
3. Graph y = 4x - 2

Absolute Value Functions :

1. Graph f (x) = |x|

What shape are absolute value functions ?
Where is the turning point (bottom of the “v”)?
State the Domain and the Range of the function.

2. Graph f(x) = |x + 1| + 3. What is the turning point? State the domain and range.

3. Graph f(x) = -|x|. What is the turning point? State the domain and range.
Dooley

4. Graph f(x) = -|x - 3| - 2. What is the turning point? State the domain and range.

Homework: pg. 525 #5, 13, 17, 19, 35, 37, 39, 41, 45

Review of Factoring ; Quadratic Equations and Rational Equations

Factor .

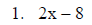
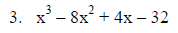
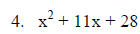
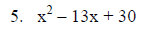
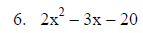
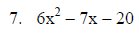
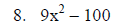
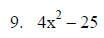
Solving Quadratic Equations :
Solve.

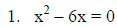
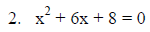
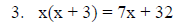
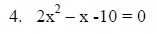
Solving Rational Expressions :
Solve.

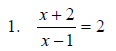
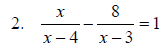
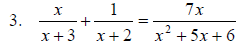
Homework: pg. 538 #9, 11, 13, 15, 17, 19, 25, 27, 29, 41, 43, 47, 51, 55, 75, 79, 81