Two Methods for Solving Quadratic Equations

Taking Square Roots and Completing the Square

You MUST use the method you are told to use, even when it is not the best method for that situation.
Each method is important for future topics.

If no method is specified, how do you decide which method to use? Here are suggestions:

1. When set equal to zero , can it be easily factored ?
If so, factoring will usually be the fastest and easiest way to solve it. If not, try another method.

2. Can the equation be written in the form "perfect square = constant?
If so, taking square roots will usually be the fastest and easiest way to solve it . If not, try another method.

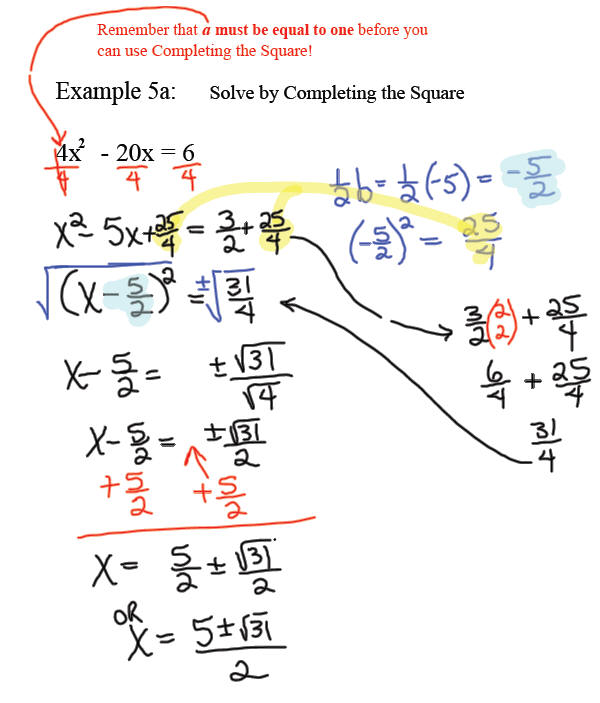
3. When set equal to zero is a = 1 and is b an even number ?
If so, it is a good candidate for completing the square. If a is not 1 and/or b is not even, another method will be faster
and easier.

4. If the equation is not in the ideal form for any of the first three methods
Use the quadratic formula .

Remember: When solving an equation, it is "legal" to:
Add the same quantity (positive or negative) to both sides of an equation.
Multiply /divide both sides of an equation by the same quantity.
Take both sides of an equation to the same power (matches the index )
NEW in Chapter 9:
Take the same root of both sides of an equation ( matches the power .)

Solve Quadratic Equations by Taking Square Roots. Form of equation: square = constant

Example 1: square = constant so take square roots	Example 2a: need square = constant	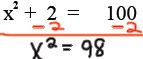Example 2b: need square = constant so isolate the square first
	square = constant so take square roots	square = constant so take square roots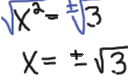
Example 2c: isolate the square first	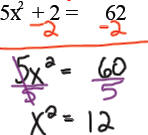Example 3a: square = constant so take square roots	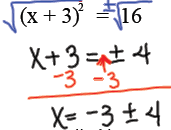Example 3b: square = constant so take square roots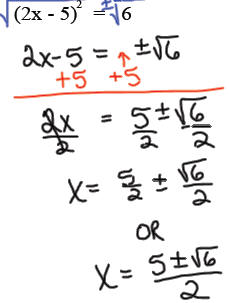
square = constant so take square roots	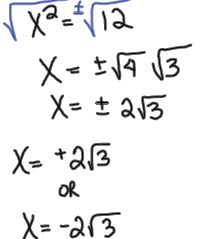no radical in answer , separate and do arithmetic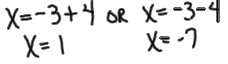

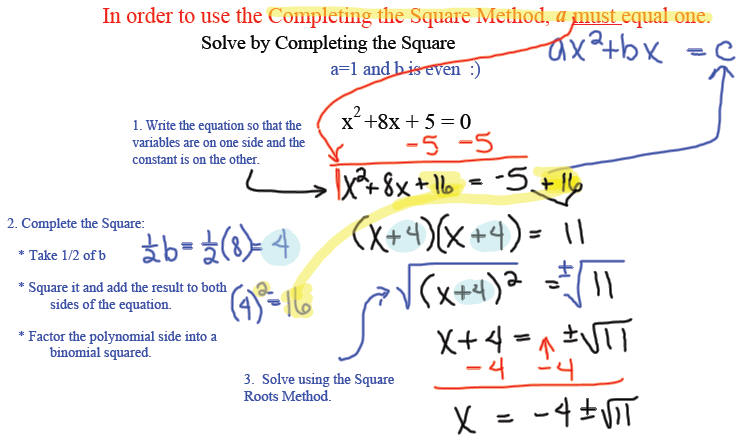
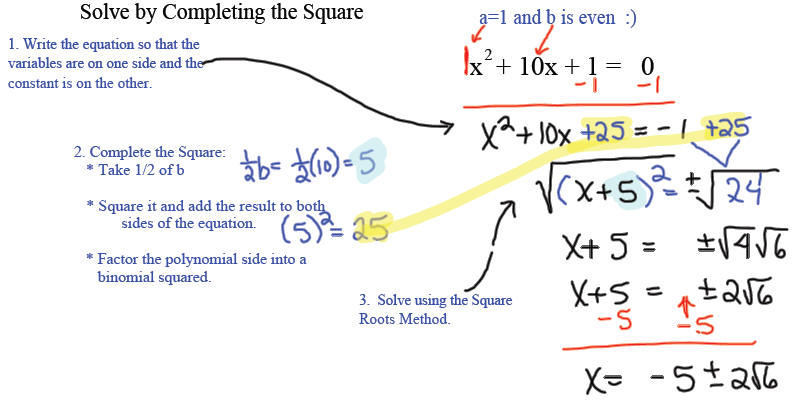
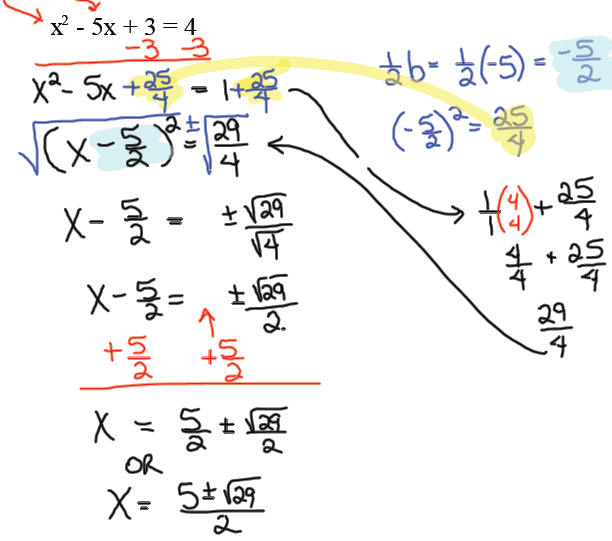
Example 4b: Solve by Completing the Square
DO NO USE DECIMALS IN PLACE OF FRACTIONS!!!!!!!!!!!!!!!!!!!!
a=1 but b is odd, so our job is slightly more difficult. :(