Factoring_Trinomials30
Factoring Trinomials
Trinomials of the Form x2 +b x +c
If a polynomial of the form x2 + b x + c is factorable
then it can be written as (x + u) (x + v).
Note: (x + u) (x + v) = x2 + v x + u x + u ٠ v = x2 + (u + v) x + u ٠ v
Looking at this carefully we see that if a trinomial of the form x2 + b x + c
is factorable then b = u + v and c = u٠v, for some
numbers u and v . So to factor this form of a polynomial we need to find two
numbers that have product c and that have sum b .
Examples
Example 1: Factor x2 + 7 x + 12
Solution : We need to find two numbers that multiply to be
12, but add to be 7. These two numbers are 3 and 4. This then gives
the factorization
x2 + 7 x + 12 = (x + 3) (x + 4)
Example 2: Factor x2 - 12 x + 35
Solution: We need to find two numbers that multiply to be
35, but add to be -12. These two numbers are -7 and - 5. This then
gives the factorization
x2 - 12 x + 35 = (x - 7) (x - 5)
Example 3: Factor x2 + 5 x - 24
Solution: We need to find two numbers that multiply to be
-24, but add to be 5. These two numbers are -3 and 8. This then
gives the factorization
x2+ 5 x - 24 = (x - 3) (x + 8)
Trinomials of the Form ax2 +b x +c
If a polynomial of the form a x2 + b x + c is factorable
then it can be written as (m x + u) (n x + v).
Note:
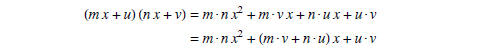
Looking at this carefully we see that if a trinomial of
the form a x 2 + b x + c is factorable then b = m٠ v + n ٠ u and
a٠ c = m٠ n ٠u ٠v = (m٠v)(n ٠u), for some numbers m, n, u and v. So to
factor this form of a polynomial we need to find two
numbers that have product a ÿ c and that have sum b. We can then rewrite the
polynomial as four terms (similar to the first step
above) and then factor by grouping .
Examples
Example 1: Factor 6 x2 + 7 x + 2
Solution: First we find the product of a and c . In this
case it's 6 * 2 = 12. So we need two numbers that multiply to be 12, but add
to be 7. These numbers are 3 and 4. We use these numbers to rewrite the middle
term as 3 x + 4 x and then factor by grouping as
follows :
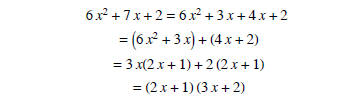
Example 2: Factor 10 x2 - 23 x + 12
Solution: First we find the product 10 * 12 = 120. So we
need two numbers that multiply to be 120 and add to be -23. These two
numbers are -15 and - 8. So the middle term can be rewritten as -15 x - 8 x. Now
factor by grouping.
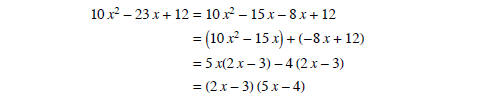
Example 3: Factor 24 x2 - 14 x - 3
Solution: First we find the product 24 *-3 = -72. So we
need two numbers that multiply to be -72 and add to be -14. These
two numbers are -18 and 4. So the middle term can be rewritten as -18 x + 4 x.
Now factor by grouping.
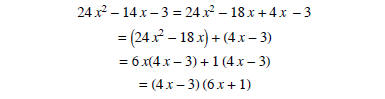
| Prev | Next |