Distance and Midipoint Formula; Circle

Objectives:
1. To find the distance between two points
2. To find the midpoint of a line segment
3. To write the standard form of a circle’s equation
4. To give the center and radius of a circle whose
equation is in standard form
5. To convert the general form of a circle’s equation to
standard form
Objective 1: To find the distance between two points
The distance Formula
Given two points P₁(x₁,y₁) and P₂(x₂,y₂)

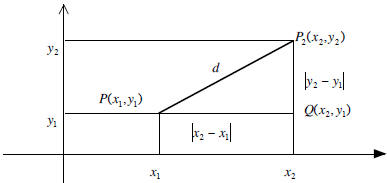
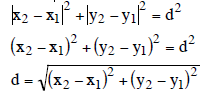
Example: Find the distance between (-4, -1) and (2, - 3).

Objective 2: To find the midpoint of a line segment
Finding the midpoint
Formula :

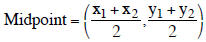
Find the midpoint of the line segment with endpoints
(-4,-1) and (2, - 3)

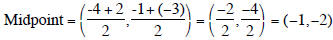
Objective 3: To write the standard form of a circle’s
equation

Circle:
A circle is the set of all points in a plane that are
equidistant from a fixed point called the center. The fixed
distance from the circle’s center is called the radius.

Find the formula of a circle whose center is (h, k) and
radius is r.

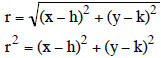
The equation of a circle whose center is at the origin and
radius r is x² + y² = r².

Write the standard form of the equation of the circle with
center at (0,0) and the radius is 4. Graph the circle on the
board.
Equation: x² + y² = 16.

Write the standard form of the equation of a circle with
center (-2, 1) and radius 2. Graph circle on the board.
Equation:

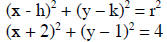
Objective 4: To give the center and radius of a circle
whose equation is in standard form

Give the center and radius of a circle whose equation is
(x + 4)² + (y + 5)² = 36.
The center is (-4,-5) and the radius is 6.

Objective 5:
To convert the general form of a circle’s equation to
standard form
The general form of the equation of a circle is
x² + y² Dx + Ey + F= 0

Standard Form: (x - h)² + (y – k)² = r²
Express x² + y² +8x + 4y + 16= 0 in standard form and
find the center and the radius.

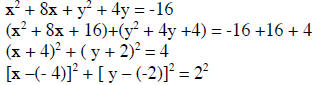
Center: (-4,-2) and radius = 2

Express x² + y² - 6y - 7= 0 in standard form and find the
center and the radius.

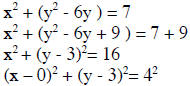
Center: (0, 3) and radius is 4