Introduction to Factoring

Greatest Common Factor (GCF): the largest whole number that divides evenly into the numbers

Examples:
1. Find the GCF of 12 and 20.
2. Find the GCF of 48, 120, and 156.

3. Find the GCF of 12x^5 and 28x^3.

4. Find the GCF of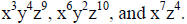

Factoring out the GCF :
1. Factor 6x^4 – 42x^3 + 90x^2

2. Factor 12x^3 + 6x – 3

3. Factor 5x(x – 9) + 14(x – 9)

4. Factor 7x(x – 8) – 6(x – 8)

Factoring by Grouping – 4 Terms
1. x^3 + 4x^2 + 7x + 28

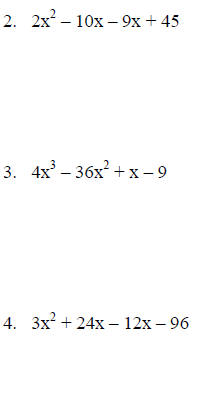
Homework: pg. 362 #5,9,13,17,19,21,25,27,31,37,39,41,43,45,51,53,59

Factoring Trinomials of the Form 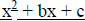when the coefficient of 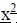is 1.

Examples: Factor

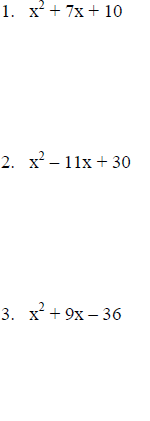
4. 5x^2 + 40x + 60 (look for GCF)

 

 

 

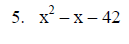
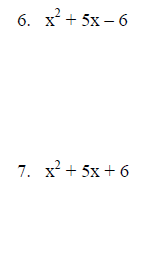
8. –x^2 + 14x – 48 (look for GCF)

 

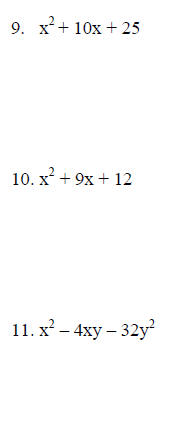
Homework: pg. 369 #5-53 ever other odd (5,9,13,…)

Factoring Trinomials of the Form

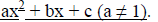
Examples: Factor

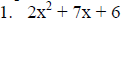
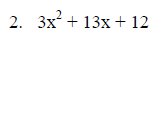
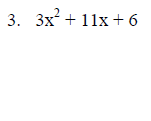
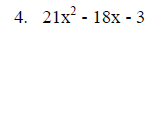
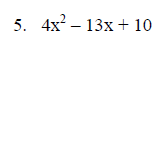
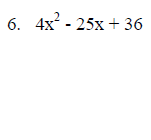
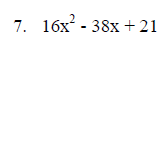
Homework: pg. 377 #3-29 (odd), 31-65 (every other odd)

Factoring Special Binomials

Difference of Squares : a^2 – b^2 = (a + b)(a – b)

Examples:
Factor.

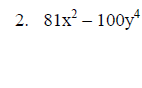
3. 7y^2 – 252 (look for GCF)

 

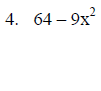
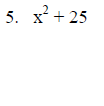
Difference of Cubes : a^3 - b^3 = (a – b)(a^2 + ab + b^2)
Sum of Cubes : a^3 + b^3 = (a + b)(a^2 - ab + b^2)

Examples:
Factor.

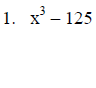
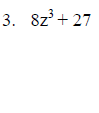
Homework: pg. 384 #7-51 (every other odd), 53-87 (odd)

Factoring a Polynomial:

1. Factor out any common terms .

2. Determine the number of terms in the polynomial .
a. If there are only two terms, check to see if the binomial is one of the special binomials discussed in Section 6.4.
 • Difference of Squares: a^2 – b^2 = (a+b)(a-b)
 • Sum of Squares: a^2 + b^2 is not factorable
 • Difference of Cubes: a^3 – b^3 = (a-b)(a^2+ab+b^2)
 • Sum of Cubes: a^3 + b^3 = (a+b)(a^2-ab+b^2)

b. If there are three terms, try to factor the trinomial using the techniques of Sections 6.2 and 6.3.
 • x^2 + bx + c = (x+m)(x+n): find two integers m and n whose product is c and whose sum is b.
 • ax^2 + bx + c (a≠1): factor using grouping, refer to object 1 in Section 6.3

c. If there are four terms, try factoring by grouping, discussed in Section 6.1.

3. After the polynomial has been factored, be sure that any factor with two or more terms does not have any common factors other than 1. If there are common factors, factor them out.

4. Check your factoring through multiplication .

Examples.
Factor.

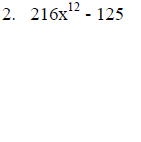
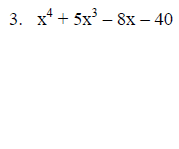
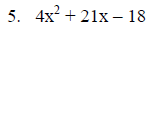
Homework: pg. 390 # 7-61 (every other odd)

Solving Quadratic Equations by Factoring:

If a*b=0, then either a = 0 or b = 0.

Examples:
Solve.

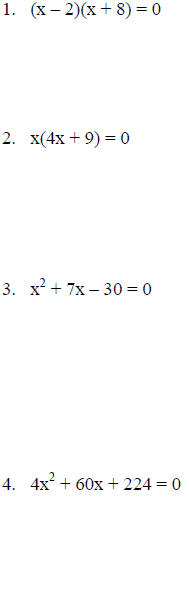
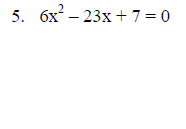
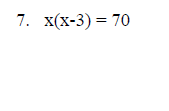
8. Find a quadratic equation that has the solutions -6 and 8.

 

9. Find a quadratic equation that has the solutions -2 and -5.

 

Quadratic Functions:

Examples:
1. For f(x) = x^2 – 9x + 12, find f(-6).

 

2. Let f(x) = x^2 – 17x + 72. Find all values for x so that f(x) = 0.

 

3. Let f(x) = x^2 – 6x + 20. Find all values for x so that f(x) = 92.

 

Homework: pg. 404 #3,5,7,9,21,23,25,27

Applications of Quadratic Equations and Quadratic Functions

1. The product of two consecutive positive integers in 132. Find the two integers

 

2. The product of two consecutive even negative integers is 80. Find the two integers.

 

3. One positive number is 9 more than a second number, and their product is 112. Find the two numbers.

 

4. One positive number is 3 more than twice a second number, and their product is 189. Find the two numbers.

 

5. The area of a rectangle is 105 square feet . If the length of the rectangle is 8 feet more than its width, find the dimensions of the rectangle.

 

6. A homeowner poured a rectangular concrete slab in her backyard to use as a barbecue area. The length is 3 feet more than its width. There is a 2-foot wide flower bed around the barbecue area. If the area covered by the barbecue area and the flower bed is 270 square feet, find the dimensions of the barbecue area.
Dooley