Math 20A Final Review Outline
Chapter 4: Applications of the Derivative
Section 4.1: Linear Approximation and Applications
• Let Δ f = f(a+ Δx)−f(a). The linear approximation is the estimate Δf ≈
f'(a)Δx for small
Δx.
• Know the formula for the tangent line approximation to f(x)
![]() f(x) L(x) = f'(a)(x −
a) + f(a).
f(x) L(x) = f'(a)(x −
a) + f(a).
- Note: This is just the point- slope formula . To avoid confusion, if you are
asked to find
the equation of a tangent line, follow the methods that we did previously .
1. Find the slope by finding the derivative and plugging in the point
2. Find a point on the curve (if not already given) by plugging x into the
function
3. Use point-slope to find the equation of the line
- Note: Local approximation or tangent line approximation may also be referred
to as
(local) linearization. We have too many terms for the same concept. Be careful.
- See the chapter for examples for finding tangent lines. Some questions may read:
"Find
the linear approximation to the function f(x) = tan(x) at 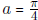 ." or "Find the lineariza-
." or "Find the lineariza-
tion of f(x) = sin(2x) at x = 0."
- Suppose g(1) = 2 and g'(1) = 3. Find the equation of the tangent line at (1,
2).
- Let f(x) = (3x−x2)e2x. (i) Find the linear approximation to f(x) near x = 5.
(ii) Using
the result from part (a), estimate by how much f(x) changes if x changes from 5
to 4.75.
• Know that the error in the linear approximation is given by
Error = l Δ f − f'(a) Δx l
• Know how to compute the percentage error (in addition to the error ):
Percentage 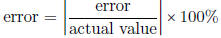
Section 4.2: Extreme Values
• Know what is meant by an extreme value of a function on an interval [a, b]
• Know that the extreme value theorem says that if you have a continuous
function on a closed
interval, then it will have a global maximum and a global minimum.
• Know what it means for f(c) to be a local minimum or a local maximum of a
function f(x)
• Know what it means for x = c to be a critical point of
f(x)
• Know Fermat's Theorem which says that if f(c) is a local minimum or a local
maximum,
then c is a critical point
• Know that f(x) has a critical point wherever the first derivative is equal to 0.
- Note: This is not the same as f(x) having a max or a min at this point. It is
possible
that f'(p) = 0 and p is not a local max or min!
• Know that f(x) has a global minimum at a point p if f(p) ≤ f(x) for all x.
• Know that f(x) has a global maximum at a point p if f(p) ≥ f(x) for all x.
• Know how to find the global max/min of a function on a closed interval:
1. First, find the critical points on the interval and their corresponding
y-values.
2. Find the y-values of the end points of the interval
3. The largest y-value corresponds with the global max on the interval and the
smallest
y-value corresponds with the global min on the interval.
- Find the absolute maximum and minimum values of f(x) = x3 − 9x2 + 15x + 3 on
the
interval [0, 2].
- Find the absolute maximum and minimum values of f(x) = 3x4 + 4x3 − 12x2 + 7 on
the
interval [−1, 2].
• Know Rolle's Theorem
![]() If f(x) is continuous on [a, b] and
differentiable on (a, b), and if f(a) = f(b),
then there
If f(x) is continuous on [a, b] and
differentiable on (a, b), and if f(a) = f(b),
then there
exists c between a and b such that f'(c) = 0
Section 4.3: The Mean Value Theorem and Monotonicity
• Know the Mean Value Theorem
![]() If f(x) is continuous on [a, b] and
differentiable on (a, b), then there exists
a number c
If f(x) is continuous on [a, b] and
differentiable on (a, b), then there exists
a number c
between a and b such that 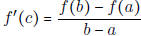 .
.
• Know the Constant Function Theorem
![]() If f(x) is continuous on [a, b] and
differentiable on (a, b) and if f'(x) = 0
on (a, b), then
If f(x) is continuous on [a, b] and
differentiable on (a, b) and if f'(x) = 0
on (a, b), then
f(x) is constant on [a, b].
• Know what it means for a function to be monotonic on the
interval (a, b)
• Know the Increasing Function Theorem
![]() If f(x) is continuous on [a, b] and
differentiable on (a, b), then
If f(x) is continuous on [a, b] and
differentiable on (a, b), then
(i) If f'(x) > 0 on (a, b), then f(x) is increasing on [a, b] and
(ii) If f'(x) ≥ 0 on (a, b), then (x) is nondecreasing on [a, b].
- (i) Show that the equation 4x5 + x3 + 2x + 1 = 0 has a root in the interval
[−1, 0]. (ii)
Show that the equation 4x5 +x3 +2x+1 = 0 has only one real root. In other words,
show
that it cannot have two roots .
• Know the Racetrack Principle
![]() If g(x) and h(x) are continuous on [a, b] and
differentiable on (a, b), and
g'(x) ≤ h'(x) on
If g(x) and h(x) are continuous on [a, b] and
differentiable on (a, b), and
g'(x) ≤ h'(x) on
(a, b), then
(i) If g(a) = h(a), then g(x) ≤ h(x) on [a, b]
(ii) If g(b) = h(b), then g(x) ≥ h(x) on [a, b]
• Know the first-derivative test for local max/min
- If p is a critical point and the derivative changes from neg to pos, p is a
local min
- If p is a critical point and the derivative changes from pos to neg, p is a
local max
- If p is a critical point and the derivative does not change, p is neither
• To make this a bit simpler , draw a table

- The vertical lines represent where the function has
critical points
- In each area, circle whether the derivative is positive or negative (and the
shape below).
- If f'(x) > 0, f(x) is increasing and if f'(x) < 0, f(x) is decreasing.
- The arrows will give you a rough shape of the graph. From that, you can see if
the
critical points are local maxs, mins, or neither.
- Let f(x) = 5 ln(x2 + 4) − 2x, then 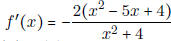
(i) Find the critical points of f(x). (ii) Determine the interval(s) on which
f(x) is
increasing and the interval(s) on which f(x) is decreasing. (iii) For each
critical point
found in part (a), indicate whether it is a local minimum, local maximum, or
neither
without computing f''(x). Be sure to state how you arrived at your conclusion(s).
Section 4.4: The Shape of a Graph
• Know what it means for a function to be concave up or concave down
• Know whether or not a function is concave up or concave down (the sign of
f ''(x))
• Know that f(x) may have point of inflection wherever the second derivative is
equal to 0.
- Make another table like above , but for f''(x) and f(x)
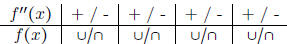
The vertical lines represent where the function has points
of inflection
- In each area, circle whether the second derivative is positive or negative
(and the shape
below).
- If f''(x) > 0, f(x) is concave up and if f''(x) < 0, f(x) is concave down.
- The curves will give you a rough shape of the graph.
• Know how to use the second-derivative test to classify the critical points.
- If p is a critical point and f''(p) > 0, then p is a local min
- If p is a critical point and f''(p) < 0, then p is a local max
- If p is a critical point and f''(p) = 0, then no information is known from
this test
• Given a graph of f '(x), know how to determine where f(x)
is
increasing/decreasing and concave up/concave down.
| - Given the graph of f'(x) (right),
find the
interval(s) where f(x) is increasing, decreasing, concave up, concave down, as well as list the x- coordinate (s) of all local max/min and inflection points of f(x). |
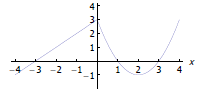 |
• Given an equation of f(x), know how to determine where
f(x) is increasing/decreasing,
concave up/concave down, as well as local/global max/mins.
- Let f(x) = x3 − 9x2 + 24x. On what intervals is f(x) increasing? Decreasing?
Concave
up? Concave down? Find all local max/min. Find all global max/min on the
interval
[0, 7].
| Prev | Next |