Solving Equations & Transforming Formulas
Solving Equations
To solve an equation, it is necessary to isolate the variable on one side of
the equal sign (usually, but not necessarily, to the left.) The properties noted
below will assist you as you do this work.
Distributive property = a(b + c) = ab + ac
Addition property = If a = b, then c + a = c + b
Subtraction property = If a = b, then c - a = c - b
Division property = If a = b, then c/a = c/b
Multiplication property = If a = b, then c x (a) = c x (b)
Follow these steps:
1. If there are parentheses , perform the appropriate operations in order to
remove them.
2. If there are fractions, multiply both sides of the equation by the common
denominator, in order to cancel all denominators.
3. Combine any like terms appearing on each side of the equation.
4. Rewrite the equation with all terms containing the variable on one side of
the equation and all terms not containing the variable on the other side of the
equation by adding and/or subtracting terms as needed (see hint below.)
5. Complete the solution by multiplying on both sides by the reciprocal of the
coefficient (or by dividing both sides of the equation by the coefficient) so
that the variable is finally alone.
HINT: First decide which term must be moved. After noting what operation is being performed on the term you want to eliminate, you must perform the opposite operation on that term. For example, if you want to eliminate a term that is being subtracted, then add it to both sides of the equation. Another way to look at this is to ask yourself, “What do I have to combine with this m (the one you want to eliminate) to make zero?” Remember to do it to both sides.
Some examples
| 25 = S - 5 | |
| 25 + 5 = S - 5 + 5 | Add 5 to both sides to isolate S. |
| 30 = S | The coefficient of S is positive one, so the formula is solved . |
| S = 30 | S is rewritten on left for convenience. |
| 10 = 2R | |
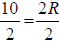 |
Divide both sides by the coefficient of the variable R . |
| 5 = R | |
| R = 5 | Rewrite with R on the left. |
Solving Equations & Transforming Formulas
| -5 = 5(3 - 2x) | |
| -5 = 15 - 10x | Use the distributive property to remove parentheses . |
| -5 +10x = 15 - 10x +10x | Add 10x to both sides to get the variable on the left. |
| -5 + 10x = 15 | |
| -5 + 5 + 10x = 15 + 5 | Add 5 to both sides to get the constants on the right. |
| 10x = 20 | |
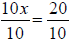 |
Divide both sides by the numerical coefficient. |
| x = 2 | |
| 7 + c2 = 107 | |
| 7 – 7 + c2 = 107-7 | Isolate c2 by subtracting 7 from both sides. |
| c2 = 100 | |
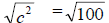 |
Take the square root of both sides. |
| C = 10 | Rewrite. |
The ability to clear fractions in a linear equation is a very important skill. Study this example and use it as a model for simplifying these fearsome equations; you will find lots of them on this worksheet.
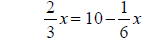 |
Find the common denominator , in this case 6. |
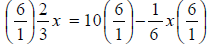 |
Multiple each term by 6/1 |
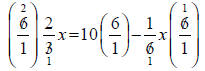 |
Simplify (cancel). |
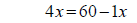 |
Clean up the equation by rewriting it. |
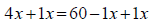 |
Add 1x to both sides to get the variable on one
side and the constant on the other. |
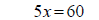 |
Clean up the equation again by rewriting it. |
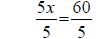 |
Divide both sides by the numerical coefficient. |
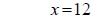 |
Solving Practice
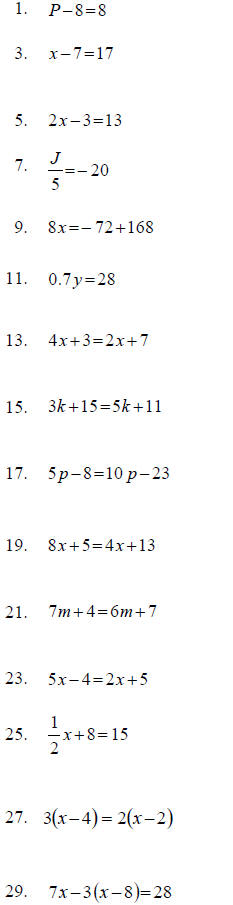
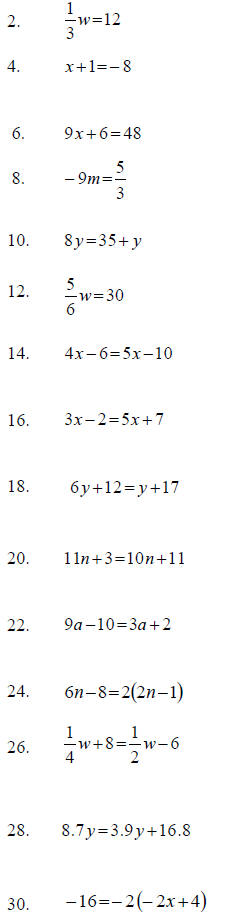
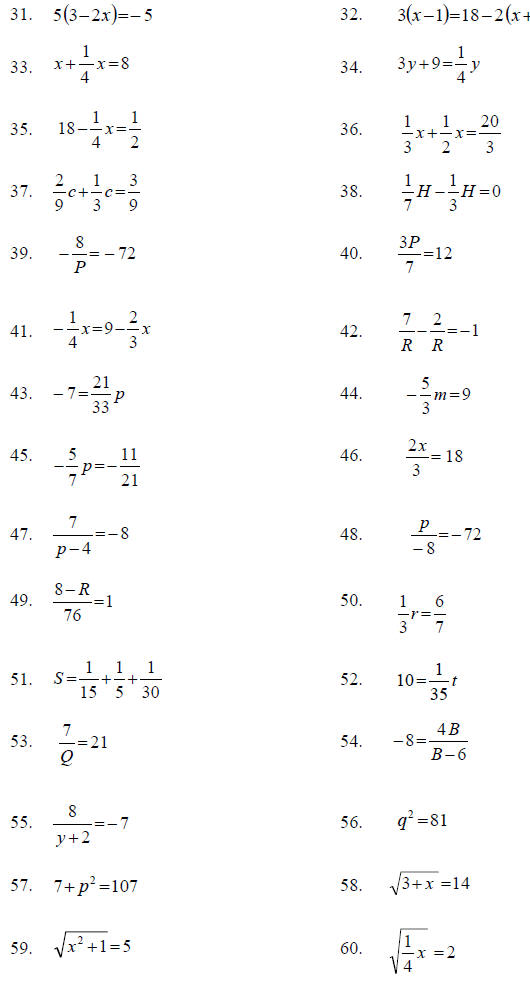

| Prev | Next |