Multiplying and Dividing Rational Expressions
I. Rational Expressions and Functions
A. A rational expression is a polynomial divided by a
nonzero polynomial.
B. A rational function is a function defined by a formula that is a rational
expression.
C. Fractions are rational expressions.
Examples:

What value(s) of x would make the denominators of the
above examples equal to 0?
1)
2)
3)
Why do these values matter ?
C. The domain of a rational function is the set of all real numbers except those
Examples: Find the domain of the following functions. (Easiest to use set-builder notation.)

II. Simplifying Rational Expressions
A. To simplify, reduce , or write in lowest terms means the same thing: the
numerator and denominator
don’t contain any common factors (other than 1 or -1).
B. To simplify:
1) Factor completely the numerator and denominator .
2) Divide both the numerator and denominator by the common factors; that is,
cancel out any
common factors that the numerator and denominator share. NEVER cancel TERMS ;
only FACTORS.
Examples: Simplify.
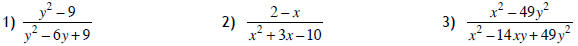
III. Multiplying Rational Expressions
A. Multiplying rational expressions is the same as multiplying fractions:

B. To multiply rational expressions:
1) Factor completely all numerators and denominators.
2) Divide numerators and denominators by common factors.
3) Multiply the remaining numerators; multiply the remaining denominators.
Examples: Multiply.

IV. Dividing Rational Expressions
A. Dividing rational expressions is the same as dividing fractions:
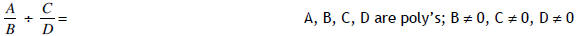
C. To divide rational expressions:
1) Leave the first rational expression alone; change division to multiplication;
“flip” the divisor.
2) Now it is a multiplication problem. Proceed with multiplication.
Examples: Divide.

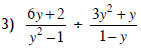
| Prev | Next |