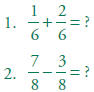The California Mathematics Content Standards
Grade Three Mathematics Content Standards
By the end of grade three, students deepen their
understanding of place value and their
understanding of and skill with addition, subtraction, multiplication, and
division of whole
numbers. Students estimate, measure, and describe objects in space. They use
patterns to
help solve problems. They represent number relationships and conduct simple
probability
experiments.
|
Number Sense 1.0 Students understand the place value of whole numbers: 1.1 Count, read, and write whole numbers to 10,000. What is the smallest whole number you can make
using the digits 4, 3, 9, 1.2 Compare and order whole numbers to 10,000.
1.4 Round off numbers to 10,000 to the nearest ten, hundred, and thousand.
True or false? 2.0 Students calculate and solve problems
involving addition, subtraction,
1. 591 + 87 = ? To prepare for recycling on Monday, Michael
collected all the bottles in
2.5 Solve division problems in which a multidigit
number is evenly divided by 2.6 Understand the special properties of 0 and 1 in multiplication and division. True or false? 2.7 Determine the unit cost when given the total
cost and number of units. 3.0 Students understand the relationship
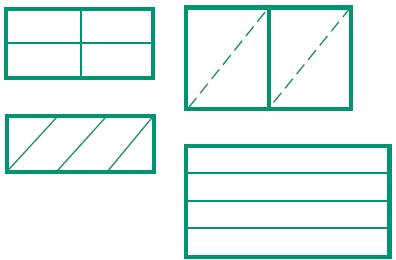
between whole numbers, simple fractions, 3.1 Compare fractions represented by drawings or
concrete materials to show Which is longer: 1/3 of a foot or 5 inches? 2/3
of a foot or 9 inches?
Find the values:
Pedro bought 5 pens, 2 erasers and 2 boxes of
crayons. The pens cost 3.4 Know and understand that fractions and
decimals are two different representations |
Note: The sample problems illustrate the standards and are written to help clarify them. Some problems are written in a form that can be used directly with students; others will need to be modified, particularly in the primary grades, before they are used with students. The symbol
|
|
|
Algebra and Functions 1.0 Students
select appropriate symbols, operations, and properties to represent,
1.2 Solve problems involving numeric equations or
inequalities . 2.0 Students represent simple functional relationships:
John wants to buy a dozen pencils. One store
offers pencils at 6 for $1. 2.2 Extend and recognize a linear pattern by its
rules (e.g., the number of legs on Here is the beginning of a pattern of tiles.
Assuming that the pattern
|
||
|
Measurement and Geometry
1.0 Students choose and use appropriate units and
measurement tools to quantify 1.1 Choose the appropriate tools and units (metric
and U.S.) and estimate and
1.4 Carry out simple unit conversions within a
system of measurement 2.0 Students describe and compare the
attributes of plane and solid geometric
2.4 Identify right angles in geometric figures or
in appropriate objects and
2.5 Identify, describe, and classify common
three-dimensional geometric objects |
||
|
Statistics, Data Analysis, and Probability
1.0 Students conduct simple probability experiments
by determining the number 1.1 Identify whether common events are certain,
likely, unlikely , or improbable.
1.4 Use the results of probability experiments to
predict future events (e.g., use a |
||
|
Mathematical Reasoning 1.0 Students
make decisions about how to approach problems: 2.2 Apply strategies and results from simpler
problems to more complex |
| Prev | Next |
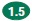 Use expanded
notation to represent numbers (e.g., 3,206 = 3,000 + 200 + 6).
Use expanded
notation to represent numbers (e.g., 3,206 = 3,000 + 200 + 6).