Fifth Grade Vocabulary
| Categorical Data: Data with no
established arrangement or numerical order . Data that can be put into categories. |
Example: For M&Ms, each M&M is either red, green, blue, yellow, orange or brown. Thus, we can classify each M&M by putting it in one of each of these categories. 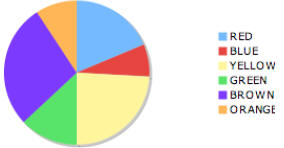 |
| Compose/Decompose: To put
shapes together (compose) or to take shapes apart (decompose). |
 The hexagon is
composed of six triangles. The hexagon is
composed of six triangles.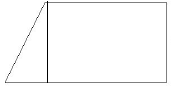 The trapezoid can be decomposed into a triangle and a rectangle, making it easier to find its area. |
| Convex Polygon: A polygon in
which all vertices are “pushed outward.” If you connect two non-consecutive vertices, the segment would lie entirely inside the polygon. |
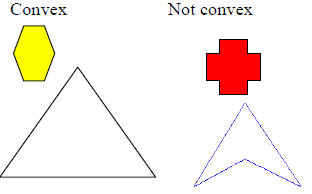 |
| Divisibility Rules: Rules that
determine whether a number is divisible by a certain number. |
*All even numbers are evenly divisible by two. *If the sum of the digits of a number is divisible by 3, the number is divisible by 3. *Numbers ending in 5 or 0 are divisible by 5. |
| Edges/Faces: A line segment
where two faces of a polyhedron meet is an edge. A flat surface on a 3-dimensional shape is a face. |
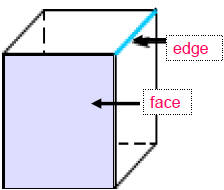 |
| Equation (Modeling): A
mathematical sentence that shows that two quantities are equal To solve an equation, find a value for the variable that makes the sentence true. |
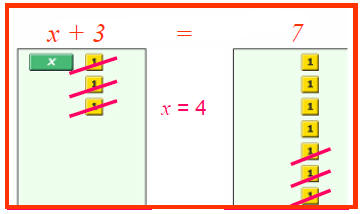 |
| Exponential Notation: A way to
show repeated multiplication by the same factor. A number written with a base and an exponent. |
 |
| Greatest Common Factor/Least
Common Multiple: The largest number that can be divided evenly into each number in a set is called the greatest common factor .
|
|
| Inverse Operation: An
“operation” that reverses another operation. It undoes the effect of another operation such as addition, subtraction, multiplication or division. |
Addition and subtraction are inverse operations (undo adding 3 by subtracting 3) Multiplication and division are inverse operations (undo multiplying by 2 by dividing by 2) |
| Linear Equations/Inequalities
(Solve): An equation or inequality containing variables of degree one or constants; (no squares, cubes, etc.) such as x + 8 = 12. To solve an equation, find a value for the variable that makes the sentence true. |
x + 8 <12 is a linear inequality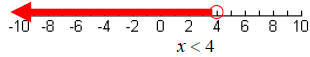 |
| Mean: The average value of a
set of data. The sum of a set of numbers divided by the number of numbers in the set. It is one of the measures of central tendency. |
In the data set 95, 85, 92, 84, the mean is 89. |
| Millions/Millionths: The
seventh place value position is the millions. The sixth place value position after the decimal point is the millionths. |
|
| Mixed number: A number that is
written using both a whole number and a fraction. |
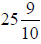 is a mixed
number. is a mixed
number.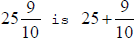 |
| Net: Two-dimensional
representation for constructing 3-dimensional shapes. |
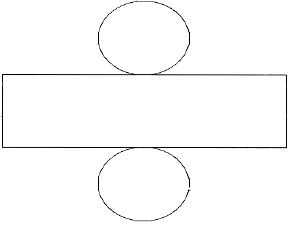 A net for a cylinder. The length of the side of the rectangle is equal to the circumference of the circle. |
| Order of Operations : Rules
that tell in what order to perform operations in arithmetic and algebra : Parentheses, exponents, multiplication and/or division from left to right, addition and/or subtraction from left to right. |
PEMDAS: Parentheses , Exponents, Multiply or Divide from left to right, Add or Subtract from left to right. (The mnemonic to remember this is “Please excuse my dear Aunt Sally”). |
| Orthogonal/Projective View: Orthogonal views of an object are from the top, front and sides. Projective views are picture views. |
Orthogonal views of a rectangular prism: Projective view: 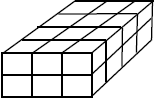 |
| Outliers: A number which is
far removed from the other numbers in a data set. Technically, they are values that lie more than one and a half times the length of the box in a box-andwhiskers plot from either end of the box. |
Upper quartile = 93, lower quartile = 64.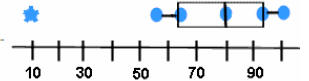 The length of the box or interquartile range (IQR) is 93 – 64 = 28 So, an outlier is any point that is more than (1.5)(28) = 42 away from the upper or lower quartiles. This means any point greater than 93 + 42=135 or less than 64 − 42 = 22 . Since there is a data point at 10, (a number less than 22), it is an outlier. |
| Polygon (Regular/Irregular): A
closed 2- dimensional figure that is made up of line segments joined end to end. A regular polygon is one whose sides are all the same length and whose angles are all equal. |
Regular polygons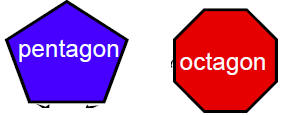 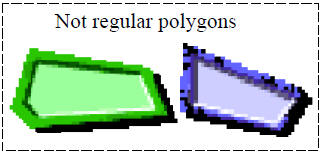 |
| Polyhedral (Regular/Platonic)
Solids: A geometric solid with polygons as faces. The faces intersect at edges and the edges come together at the vertices. The five special solids having faces which are all congruent regular polygons and the same number of polygons at each vertex are called Platonic solids. |
The five Platonic Solids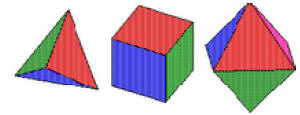 Tetrahedron Cube Octahedron 4 faces 6 faces 8 faces  Dodecahedron Icosahedron 12 faces 20 faces |
| Precision of Measurement: The
level of detail of a measurement, determined by the unit of measure. Precision depends on the smallest u of measurement being used. The number of significant digits in a measurement is an indication of the precision with which the measurement was taken. |
A ruler with 1/16 "markings would have greater precision than a ruler with only1/4" markings. |
| Prism: A solid with two
congruent, parallel faces; its other faces are all parallelograms formed by joining the vertices of the two bases. |
Prism Triangular 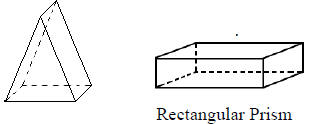 |
| Proper/Improper Fraction: A
fraction whose numerator is less than the denominator is proper . A fraction whose numerator is greater than or equal to its denominator is improper. |
 |
| Significant Digits: Digits
that express a quantity to a specified degree of accuracy. Non- zero digits are always significant. Zeros at the end of a decimal and zeros between two nonzero digits are significant . Zeros at the end of whole number and zeros immediately following a decimal point in front non-zero digits are not significant. |
7.957 has 4 significant digits. 0.07957 has 4 significant digits 0.79570 has 5 significant digits. 7,957 has 4 significant digits. 79,570 has 4 significant digits. 79,057 has 5 significant digits. 70,905,007 has 8 significant digits. |
| Substitution Property : A
mathematical rule what is the value of the expression: that states that if two quantities are equal one o the quantities can be substituted for the other in any expression. |
if x=51,what is the value of the expression: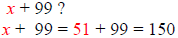 |
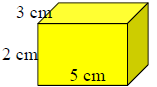
| Surface Area: The sums of the
areas of the faces of a solid figure. |
The surface area of |
| Terminating/Repeating Decimals:
A decimal number that contains a finite number of digits is called terminating. A decimal number in which one or more of its digits repeat infinitely is called repeating. |
0.25 is a terminating decimal Repeating decimals: 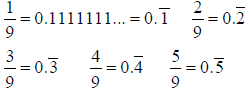 |
| Variable: A letter or other
symbol that is used to represent a number. Numbers are called constants since their values do not change . |
In the equation x + 15 = 60, x is the variable, 15 and 60 are constants. |
| Prev | Next |
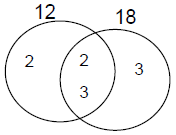 Use a Venn Diagram
Use a Venn Diagram One million is written
1,000,000.
One million is written
1,000,000.