rational exponents

Math D : 7.2

We de ne rational exponents to have the same properties as integer expo-
nents. Suppose

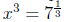
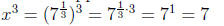
so x³ = 7 so x is the number whose cube is 7 so
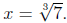
But we started with

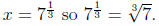
De nition 0.1 If 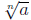represents a real number and n 2 is an integer,
then

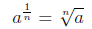
If a is negative , n must be odd.

Example 0.1 Converting from

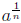
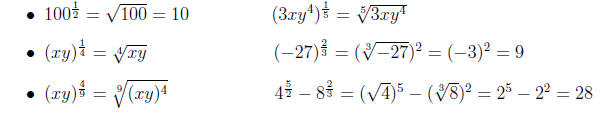
De nition 0.2 If 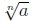represents a real number , m/n is a positive rational
number reduced to lowest terms, and n ≥ 2 is an integer, then

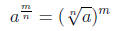
and

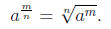
Example 0.2 Converting to

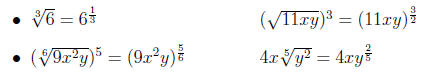
De nition 0.3 If 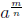is a nonzero real number , then

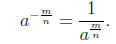
Example 0.3 Negative exponents

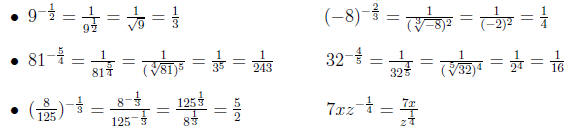
Property 0.1 Properties of Rational Exponents
If m and n are rational exponents , and a and b are real numbers for which
the following expressions are de ned, then

Example 0.4 Properties of Rational Exponents and Reducing

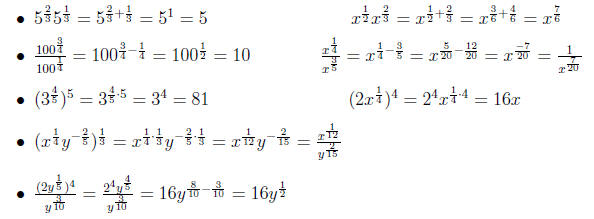
Property 0.2 Simplifying Rational Expressions

•Rewrite each radical expression with a rational exponent.
• Simplify using properties of rational exponents.
•Rewrite in radical notation .

Example 0.5 Simplify