The Story of a Number
Chapter 1
John Napier (1550-1617)
Seeing there is nothing that is so troublesome to mathematical practice, nor
that doth more molest and hinder
calculators , than the multiplications, divisions, square and cubical extractions
of great numbers. . . .
I began therefore to consider in my mind by what certain and ready art I might
remove those hindrances.
- JOHN NAPIER, Mirifici logarithmorum canonis description (1614)
Rarely in the history of science has an abstract mathematical idea been received
more enthusiastically by the entire
scientific community than the invention of logarithms. And one can hardly
imagine a less likely person to have made
that invention. His name was John Napier.
The son of Sir Archibald Napier and his first wife, Janet Bothwell, John was
born in 1550 (the exact date is unknown)
at his family's estate, Merchiston Castle, near Edinburgh, Scotland. Details of
his early life are sketchy. At the age of
thirteen he was sent to the University of St. Andrews, where he studied
religion. After a sojourn abroad he returned to
his homeland in 1571 and married Elizabeth Stirling, with whom he had two
children. Following his wife's death in
1579, he married Agnes Chisholm, and they had ten more children. The second son
from this marriage, Robert, would
later be his father's literary executor. After the death of Sir Archibald in
1608, John returned to Merchiston, where, as
the eighth laird of the castle, he spent the rest of his life.
Napier's early pursuits hardly hinted at future mathematical creativity. His
main interests were in religion, or rather in
religious activism. A fervent Protestant and staunch opponent of the papacy, he
published his views in A Plaine
Discovery of the whole Revelation of Saint John (1593), a book in which he
bitterly attacked the Catholic church,
claiming that the pope was the Antichrist and urging the Scottish king James VI
(later to become King James I of
England) to purge his house and court of all "Papists, Atheists, and Newtrals."
He also predicted that the Day of
Judgment would fall between 1688 and 1700. The book was translated into several
languages and ran through twenty-
one editions (ten of which appeared during his lifetime), making Napier
confident that his name in history-or what little
of it might be left-was secured.
Napier's interests, however, were not confined to religion. As a landowner
concerned to improve his crops and cattle,
he experimented with various manures and salts to fertilize the soil. In 1579 he
invented a hydraulic screw for
controlling the water level in coal pits. He also showed a keen interest in
military affairs, no doubt being caught up in
the general fear that King Philip II of Spain was about to invade England. He
devised plans for building huge mirrors
that could set enemy ships ablaze, reminiscent of Archimedes' plans for the
defense of Syracuse eighteen hundred
years earlier. He envisioned an artillery piece that could "clear a field of
four miles circumference of all living creatures
exceeding a foot of height," a chariot with "a moving mouth of mettle" that
would "scatter destruction on all sides," and
even a device for "sayling under water, with divers and other stratagems for
harming of the enemyes" - all forerunners
of modem military technology. It is not known whether any of these machines was
actually built.
As often happens with men of such diverse interests, Napier became the subject
of many stories. He seems to have
been a quarrelsome type, often becoming involved in disputes with his neighbors
and tenants. According to one story,
Napier became irritated by a neighbor's pigeons, which descended on his property
and ate his grain. Warned by
Napier that if he would not stop the pigeons they would be caught, the neighbor
contemptuously ignored the advice,
saying that Napier was free to catch the pigeons if he wanted. The next day/the
neighbor found his pigeons lying half-
dead on Napier's lawn. Napier had simply soaked his grain with a strong spirit
so that the birds became drunk and
could barely move. According to another story, Napier believed that one of his
servants was stealing some of his
belongings. He announced that his black rooster would identify the transgressor.
The servants were ordered into a
dark room, where each was asked to pat the rooster on its back. Unknown to the
servants , Napier had coated the bird
with a layer of lampblack. On leaving the room, each servant was asked to
show his hands; the guilty servant, fearing
to touch the rooster, turned out to have clean hands, thus betraying his guilt.
All these activities, including Napier's fervent religious
campaigns, have long since been forgotten. If Napier's name is
secure in history, it is not because of his best-selling book or his mechanical
ingenuity but because of an abstract
mathematical idea that took him twenty years to develop: logarithms.
The sixteenth and early seventeenth centuries saw an enormous expansion of
scientific knowledge in every field.
Geography, physics, and astronomy, freed at last from ancient dogmas, rapidly
changed man's perception of the
universe. Copernicus's heliocentric system, after struggling for nearly a
century against the dictums of the Church,
finally began to find acceptance. Magellan's circumnavigation of the globe in
1521 heralded a new era of marine
exploration that left hardly a corner of the world unvisited. In 1569 Gerhard
Mercator published his celebrated new
world map, an event that had a decisive impact on the art of navigation. In
Italy Galileo Galilei was laying the
foundations of the science of mechanics, and in Germany Johannes Kepler
formulated his three laws of planetary
motion, freeing astronomy once and for all from the geocentric universe of the
Greeks. These developments involved
an ever increasing amount of numerical data , forcing scientists to spend much of
their time doing tedious numerical
computations. The times called for an invention that would free scientists once
and for all from this burden. Napier took
up the challenge.
We have no account of how Napier first stumbled upon the idea that would
ultimately result in his invention. He was
well versed in trigonometry and no doubt was familiar with the formula
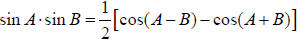
This formula, and similar ones for
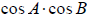and 
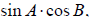were
known as the prosthaphaeretic rules, from the
Greek word meaning " addition and subtraction ." Their importance lay in the fact
that the product of two trigonometric
expressions such as sin A .sin B could be computed by finding the sum or
difference of other trigonometric
expressions, in this case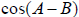 
and
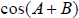. Since it is easier to add and subtract than
to multiply and
divide , these formulas provide a primitive system of reduction from one
arithmetic operation to another, simpler one. It
was probably this idea that put Napier on the right track.
A second, more straightforward idea involved the terms of a geometric
progression, a sequence of numbers with a
fixed ratio between successive terms. For example, the sequence 1, 2, 4, 8, 16,
… is a geometric progression with the
common ratio 2. If we denote the common ratio by q, then, starting with 1, the
terms of the progression are
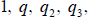and so on (note that the nth term is
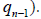Long before Napier's time, it had been
noticed that there
exists a simple relation between the terms of a geometric progression and the
corresponding exponents, or indices, of
the common ratio. The German mathematician Michael Stifel (1487-1567), in his
book Arithmetica integra (1544),
formulated this relation as follows: if we multiply any two terms of the
progression 
the result would be
the same as if we had added the corresponding exponents. For example,
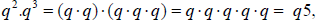a result that could have been obtained by
adding the exponents 2 and
3. Similarly, dividing one term of a geometric progression by another term is
equivalent to subtracting their exponents:
.We thus have the simple rules
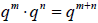and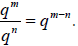 
A problem arises, however, if the exponent of the denominator is greater than
that of the numerator, as in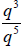 
;our rule
would give us 
an expression that we have not
defined. To get around this difficulty, we simply define
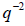to be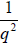 
,so
that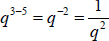 
in agreement with the result obtained
by dividing 
by
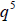directly. (Note
that in order to be consistent with the rule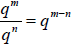
when
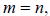, we must also define
) With these
definitions in mind, we can now extend a geometric progression indefinitely in
both directions:
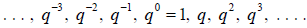We see that each term is a power of the
common ratio q, and that the
exponents. . . , -3, -2, -1, 0, 1, 2, 3, . . . form an arithmetic progression
(in an arithmetic progression the difference
between successive terms is constant, in this case 1). This relation is the key
idea behind logarithms; but whereas
Stifel had in mind only integral values of the exponent, Napier's idea was to
extend it to a continuous range of values.
His line of thought was this: If we could write any
positive number as a power of some given, fixed number (later to be
called a base), then multiplication and division of numbers would be equivalent
to addition and subtraction of their
exponents. Furthermore, raising a number to the nth power (that is, multiplying
it by itself n times) would be equivalent
to adding the exponent n times to itself - that is, to multiplying it by n - and
finding the nth root of a number would be
equivalent to n repeated subtractions - that is, to division by n. In short,
each arithmetic operation would be reduced to
the one below it in the hierarchy of operations, thereby greatly reducing the
drudgery of numerical computations.
Let us illustrate how this idea works by choosing as our base the number 2.
Table 1.1 shows the successive powers of
2, beginning with 
and ending with
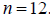Suppose we wish to multiply 32 by 128. We
look in the table for the
exponents corresponding to 32 and 128 and find them to be 5 and 7, respectively.
Adding these exponents gives us
12. We now reverse the process, looking for the number whose corresponding
exponent is 12; this number is 4,096,
the desired answer. As a second example, suppose we want to find
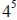. We find the exponent corresponding to 4,
namely 2, and this time multiply it by 5 to get 10. We then look for the number
whose exponent is 10 and find it to be
1,024. And, indeed,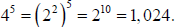 
| Prev | Next |