Solving Quadratic Inequalities

EXAMPLE: Solve 3x^2 + 3x – 6 > 0

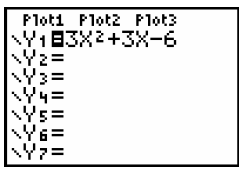
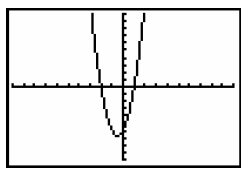
	Step 1: Press Y= Under Y₁ enter the polynomial 3x^2 + 3x – 6 Then press GRAPH We want to find where the polynomial is greater than 0.
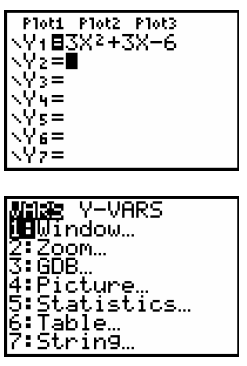	Step 2: Go back to Y= We want to know where Y₁ > 0, so let’s enter this under Y₂ Place the cursor beside Y₂= Press the VARS key
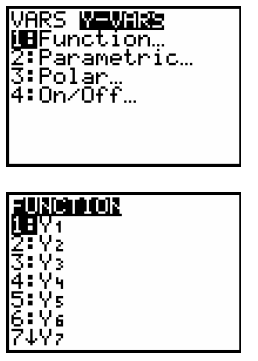	Step 3: Arrow over to Y-VARS We want to call up Y₁ which is under 1:Function so press ENTER To select Y₁ press ENTER again
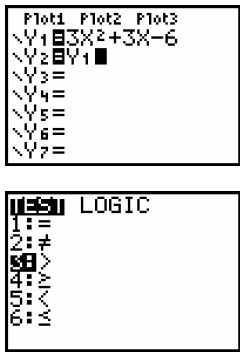	Step 4: To insert the inequality symbol , go to the TEST menu by pressing 2^nd MATH Arrow down to 3:> and press ENTER
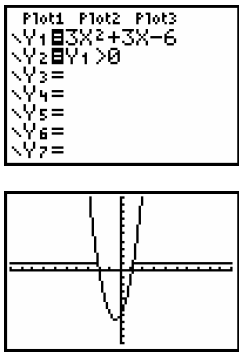	Step 5: Now enter 0 and press GRAPH The horizontal lines show which x- values have positive y-values, which is what we are looking for since 3x^2 + 3x – 6 > 0 The x- values are to the left and right of the x-intercepts.
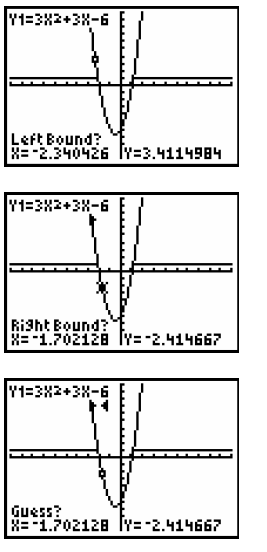
	Step 6: To find the x-intercepts, go to the CALCULATE menu by pressing 2nd TRACE Arrow down to 2: zero and press ENTER
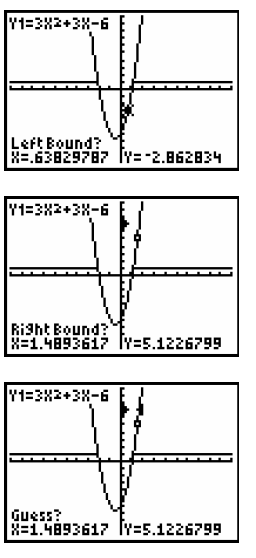	Step 7: To find the intercept on the right , use the arrow keys to move the cursor to the left of the intercept (left bound) and press ENTER Then move the cursor to the right of the intercept (right bound) and press ENTER Now press ENTER one more time (for guess)
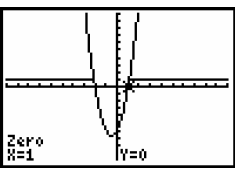	The x-value for the intercept is 1
	Step 8: To find the intercept on the left, we repeat the process. Go to the CALCULATE menu by pressing 2^nd TRACE arrow down to 2: zero and press ENTER Use the arrow keys to move the cursor to the left of the intercept (left bound) and press ENTER Then move the cursor to the right of the intercept (right bound) and press ENTER Now press ENTER one more time (for guess)
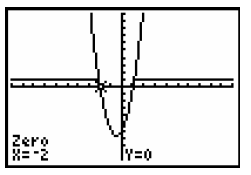	The x-value for the intercept is -2

So the solution for the inequality is