Polynomial Division, The Remainder Theorem, and The Factor Theorem
Polynomial Division , The
Remainder Theorem, and The
Factor Theorem
Long Division

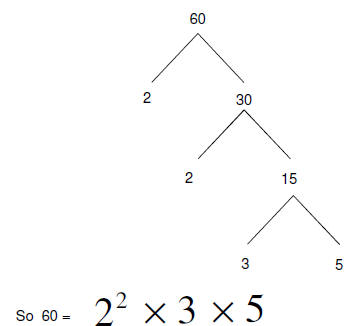
Notice: If we get a remainder of zero the quotient is a factor of the dividend
We have known how to find factors of integers using division for years
We can use long division on polynomials in a like manner
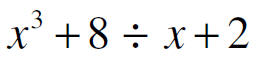
| Set-up Put the divisor outside |
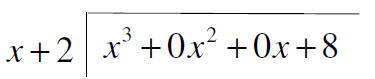 |
Put the dividend inside with
every degree of the variable shown

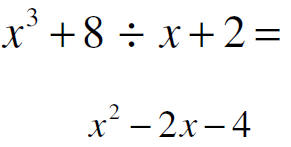
Warning: This book may represent the remainder as
some function
R(x) and say R(x) = (some constant.)
ONLY if we write the QUOTIENT as the sum of two separate
functions
would this be acceptable.
Otherwise the answer is as shown as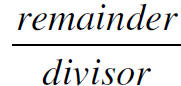
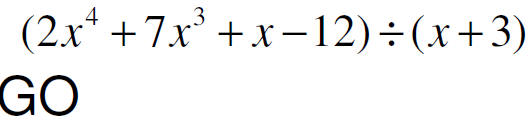
Synthetic Division
• Can only use when the divisor has a degree of 1.
Otherwise a different method is needed .
• Uses the coefficients
• Must backfill for all powers of the variable
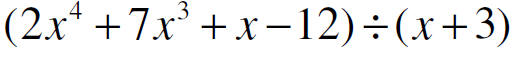
| Set-up Put the solution outside |
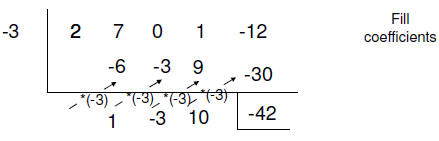 |
| The 1st coefficient moves down |
|
| Multiply by solution and move |
Add |
The numbers below the line are the coefficients to a term that is lower by 1 degree

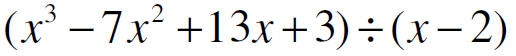
GO
The remainder Theorem
• If a number c is substituted for x in the polynomial p(x)
• then the result p(c) is the remainder that would result from dividing p(x) by
(x-c)

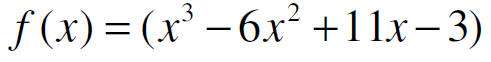
Find the remainder for: f(1) f(3) f(-2)
Check with synthetic division
The Factor Theorem
• For a polynomial p(x)
• if p(c) = 0
• then (x-c) is a factor of p(x)
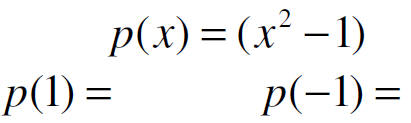
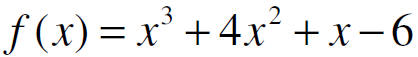
Test the following numbers to see if they are zeros using
the table feature in the calculator
1, -2, 3
If they are zeros, give the factors
| Prev | Next |