Review of Factoring Trinomials

There are many types of factoring. Most trinomial factoring that needs to be done in this
course can be done by trial and error. That is, you just play with the numbers until you
find a factorization that works . This can be very hard, however, and there is a systematic
(albeit long) way to factor a trinomial .

We will use 6x² + 39x + 54 as an example.

To factor ax ² + bx + c

Step #1: Multiply a *c.
Example: 6*54 = 324

Step #2: This is the long step , but this is the key to the method . List the ways to multiply
two numbers together to obtain a*c. You can stop when the sum of the two numbers
equals b. These two numbers will be used in the next step.

Example: We are looking for two numbers that multiply to (a*c =) 324 but add to
(b=) 39. So we just start listing the ways to multiply to numbers together to get
324. We can stop when their sum is 39.

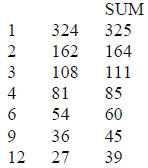
We can stop because the last one adds to39 (our b).

Step #3: We split the middle term . Instead of bx we write (number 1)x +(number 2)x
Example: 6x² + 39x + 54 = 6x² + 12x + 27x + 54

Step #4: Factor by grouping . We will review this in the second section of the course.
Example: 6x² + 12x + 27x + 54 = 6x(x + 2) + 27(x + 2) = (x + 2)(6x + 27)

Example #2

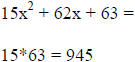
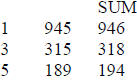
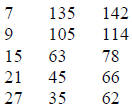
We can stop since the sum of the two numbers adds up to 62. Now we can split the
middle term .