Systems of Equations
Topic 2‐3 Solving Systems : Elimination Method
Elimination Method for Solving Systems of
Equations
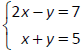 |
1. As necessary, multiply one or both coefficients by a positive or negative whole number so that a column of coefficients have the same absolute value but different signs . 2. Add the equations to eliminate a variable and create a new equation. 3. Plug the solution found in step 2 into any equation involving two variables & solve. 4. Write your solution as an ordered pair . |
Solve the system of equations.
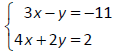
Solve the system of equations.
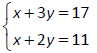
Solve the system of equations.
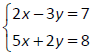
Solve the system of equations.
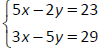
Solve the system of equations.
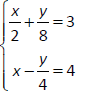
Solve the system of equations. If the system has no
solutions or infinitely‐many solutions, state that.

Solve the system of equations. If the system has no
solutions or infinitely‐many solutions, state that.
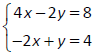
Topic 2‐4 Solving Applications of Systems, Part 1
A very important use of systems of equations is in
solving real‐world scenarios. When the values of
two things are unknown, the only way to get a
definitive solution to a situation is by having two
equations that provide information about a system.
Your responsibility will be to create systems of
equations out of statements and then appropriately
solve them and verbally state the solution.
Solving Applied Systems Problems
1. Read the question carefully.
2. Make notes, charts , or images—as necessary—to
better understand the question.
3. Translate the unknowns —as appropriate and
necessary—into mathematical variables . Clearly
define your variables with words .
4. Translate the problem into mathematical statements.
If your variables are appropriately defined, your
equations should be readable as complete sentences.
5. Solve
6. Check your solutions and interpret the solutions
appropriately using the definitions of the variables
and the mathematical statements you form.
In my pocket are fourteen coins, all of them quarters or
dimes. If the total amount of change is $2.75, how many
quarters and how many dimes do I have?
The Gold Star Baseball Team sold 311 tickets for an
afternoon game. Regular admission tickets cost $6 while
youth tickets cost $2. If the total receipts were $1542,
how many tickets of each type were sold?
In 2000, the top two destinations for conventions were
Washington, D.C. and Orlando, Florida, which between
them, hosted 353 conventions. If Washington D.C. had
27 more conventions than Orlando, find the number of
conventions held in each location.
A company rents trucks to help groups move their
equipment to and from a presentation site . On two
occasions the manager rented a truck at the same daily
rental fee and the same cost per mile. On the first date
the manager drove 50 miles and the bill was $75. On the
second date the manager drove 200 miles and the bill
was $120. How much was the daily rental fee and what
was the cost per mile?
Topic 2‐5 Solving Applications of Systems, Part 2
Focus on rates & blends
Blends ‐ you have so much of one object and so
much of a second object, how much will you have
when you mix it together?
Remember:
simple interest = principle x rate x time
distance = rate x time
Many problems involving interest are a rate and a
blend at the same time.
A Candy Barrell shop manager mixes M&M's worth $10
per pound with trail mix worth $6 per pound. Find how
many pounds of each she should use to get 40 pounds of
party mix worth $7.50 per pound.
A store is selling a mix of wildflower seeds. In the mix
is a
purple flower that costs $3 per ounce and a blue flower
that costs $7 per ounce. If the store wants to create a 12
ounce mix that will sell for $4 per ounce, how much of
each type of flower seed should be included in the mix?
A pharmacist needs 500 milliliters of a 20% saline
solution but only had 5% and 25% saline solutions
available. Find how many milliliters of each he should
mix to get the desired solution.
The pharmacist is knocked out by an evil chemist who
has decided to replace all the bottles of saline solution
with hydrochloric acid solutions. Two bottles of solution
contain 3% HCl acid in solution and 15% HCl acid in
solution. How much of each solution should be mixed
together to make 1.5 L of 7% hydrochloric acid solution.
The company Juiced‐Up wants to market a drink which is
10% real fruit juice. They receive a base containing 4%
fruit juice and mix it with pure fruit juice. How much of
the base and how much of the pure fruit juice should be
mixed together for each 64‐ounce bottle?
Sam Abney invested $9000 one year ago. Part of the
money was invested at 6%, the rest at 10%. If the total
interest earned in one year was $652.80, find how much
was invested at each rate.
Two cyclists start at the same point and travel in
opposite directions. One travels 4 mph faster than the
other. In four hours they are 112 miles apart. Find how
fast each is traveling.
| Prev | Next |