Addition and Subtraction of Fractional Numbers

TOPIC: ADDITION OF FRACTIONS
• Materials: GEOBOARDS

9. As a group, explore how to use a Geoboard to model [1/2] + [1/3] = ?

Draw a clear , well-labeled picture of your work here. (Instead of using a “This is One” circle, use a
separate Geoboard (the “This is 1 board” to keep a model of 1 for reference)
• As a group decide how many Geoboard pictures to draw for this problem.

The value of each
square is: _____

10. As a group write a brief summary of the steps that you need to show to clearly model fraction
addition with a Geoboard. Use your previous work as a guide and check to make sure you have all of
the steps that a student would need to follow your procedure. Use the terms addends and sum.
Fraction Addition Guide (Geoboards)

11. Practice Using Your Guide:
As a group, use a Geoboard to model the following. Draw a clear, well-labeled picture of your work.
(Don’t forget to use your “This is One” board for reference)
[3/(16)] + [2/8] = _____ ?

The value of each
square is: _____

12. How does your Fraction Addition Guide for Wooden Cubes compare to your Fraction Addition
Guide for Geoboards? As a group discuss which components are the same and which components
are different and summarize your discussion here:

TOPIC: FRACTION ADDITION AND SUBTRACTION
• Materials: CUISENAIRE RODS

13. As a group, use Cuisenaire Rods and work through the following to model [1/6] + [1/8].
a. We wish to model 1/6 (sixths) and we wish to model 1/8 (eighths). We know the LCM (6, 8) = 24
and so we know the LCD (1/6, 1/8) = _____.
b. We wish to use a train that is _____ whites long to model sixths and a second train (still _____
whites long) to model eighths.
These two trains look like this (use Cuisenaire Rods to model and fill in the colors here)

c. We now wish to use this model to rewrite 1/6 and 1/8 as like fractions ..

In our model this rod represents 1/6 (fill in the color):

Since 1 is a train that is 24 whites long and this rod is _____ whites long, we can rewrite 1/6 as: ______
In our model this rod represents 1/8 (fill in the color):

Since 1 is a train that is 24 whites long and this rod is _____ whites long, we can rewrite 1/8 as: ______

d. We now wish to add 1/6 and 1/8. We leave our previous model intact for reference, thus:
• FIRST we make a NEW (2^nd) model for 1
• SECOND we line up the rods for 1/6 and 1/8 to model this (fill in the like fractional names for 1/6
and 1/8):

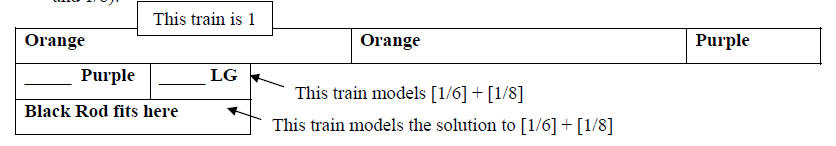
The value of the
white rod is: _____

Since 1 is a train that is 24 whites long and the black rod is _____ whites long we now know that
[1/6] + [1/8] = ______/24

14. As a group:
• Write a brief summary of the steps that you need to show to clearly model fraction addition with
Cuisenaire Rods. Use your previous work as a guide. Use the terms addends and sum.
• You may wish to combine some steps, but be very clear. You must include finding the LCD of two
unlike fractions, finding the model for 1, modeling the fractions with one train set and modeling the
fraction addition with a different train set.
• Check to make sure you have all of the steps that a student would need to follow your procedure.
Fraction Addition Guide (Cuisenaire Rods)

15. How does your Fraction Addition Guide for Cuisenaire Rods compare to your Fraction Addition
Guides for Wooden Cubes and Geoboards ? As a group discuss which components are the same and
which components are different and summarize your discussion here:

16. Practice Using Your Guide:
As a group, use Cuisenaire Rods to model the following. Draw a clear, well-labeled picture of your
work. Don’t forget to always use your model (train) for 1 in both parts (find the fractions, show the
addition) of your problem.
a. [2/3] + [1/6]

The value of the
white rod is: _____

b. [2/5] + [1/3]

The value of the
white rod is: _____

c. [5/6] + [2/9]

The value of the
white rod is: _____

17. As a group, explore how to use a Cuisenaire Rods to model [5/6] - [1/4] = ?

Draw a clear, well-labeled picture of your work. Don’t forget to always use your model (train) for 1
in both parts (find the fractions, show the subtraction) of your problem. Be sure to include finding
the LCD in your work.

The value of the
white rod is: _____

18. As a group write a brief summary of the steps that you need to show to clearly model fraction
subtraction with Cuisenaire Rods. Use your previous work as a guide and check to make sure you
have all of the steps that a student would need to follow your procedure. Use the terms minuend,
subtrahend and difference .
Fraction Subtraction Guide (Cuisenaire Rods)

19. How does your Fraction Subtraction Guide for Cuisenaire Rods compare to your Fraction
Subtraction Guide for Wooden Cubes? As a group discuss which components are the same and
which components are different and summarize your discussion here:

20. Practice Using Your Guide:
As a group, use Cuisenaire Rods to model the following. Draw a clear, well-labeled picture of your
work. Don’t forget to always use your model (train) for 1 in both parts (find the fractions, show the
subtraction) of your problem.

a. [2/3] - [1/4]

The value of the
white rod is: _____

b. [2/5] - [1/10]

The value of the
white rod is: _____

c. [5/6] - [2/9]

The value of the
white rod is: _____

LAB SEVEN DISCUSSION QUESTIONS
As a group, discuss and fill in the blank arrows. Feel free to also discuss/talk with the other groups.
What manipulatives could be used for ADDING AND SUBTRACTING FRACTIONS?
• Blocks
• Cuisenaire Rods
• Geoboards
•
•
•
•

What mathematical knowledge would you want your students to KNOW prior to introducing ADDING
AND SUBTRACTING FRACTIONS?
•
•
•
•

What mathematical knowledge would you want to EMPHASIZE while introducing ADDING AND
SUBTRACTING FRACTIONS?
•
•
•
•