Grade 8 Math

Idaho Department of Education Content Standards	Objective	Sub Objectives	Task Analysis	Essential Vocabulary	Sample Assessment
Standard 2: Concepts and Principles of Measurement
Goal 2.1: Understand and use U.S. customary and metric measurements.	8.M.2.1.1 Select and use appropriate units and tools to make formal measurements in both systems. CL: C Calc: YES Content Limit: Select appropriate units and tools only. Units for length are inches, feet, yards, miles, millimeters, centimeters, and meters. Units for time are seconds, minutes, hours, days, and years. Units for weight are ounces, pounds, tons, grams, and kilograms. Units for volume (capacity) are cups, quarts, gallons, milliliters, and liters. ‘use … tools to make formal measurements’ to be assessed in the classroom, not on the ISAT.	• Select and use appropriate units and tools to make formal measurements in both systems	• determine appropriate customary and metric units for given objects • use appropriate tools for measurement in customary and metric system	• capacity • volume • liter • meter • gram • milli- • centi- • kilo-	• Measure the length of your pencil to the nearest centimeter. • What unit of measure would you use to find the capacity of a cereal box?

	8.M.2.1.2 Apply estimation of measurement to real -world and content problems using standard measuring devices. CL: Calc: Content Limit: Assessed in the classroom, not on the ISAT.	• Apply estimation of measurement to real-world and content problems using standard measuring devices	• select an appropriate unit of measure for the problem • demonstrate magnitude of measurements	• magnitude	• About how many meters long is a classroom? • Which is more- a kilometer or a mile?
	8.M.2.1.3 Compare the differences and relationships among measures of perimeter, area, and volume (capacity) within both systems. CL: Calc: Content Limit: Assessed in the classroom, not on the ISAT	• Compare the differences and relationships among measures of perimeter, area, and volume (capacity) within both systems	• identify linear units for perimeter, square units for area, and cubic units for volume	• perimeter • area • volume • capacity • square units • cubic units • dimensions	• Compare the perimeter and area of a square with 3½" sides. What is the volume of a cube with the same dimensions?
	8.M.2.1.4 Given the formulas, find the circumference, perimeter, or area of triangles, circles, and quadrilaterals, and the volume and surface area of rectangular prisms. CL: C Calc: YES Content Limit: Items assess finding linear measure , capacity, perimeter, circumference, area, surface area, and/or volume. Items may include composite figures. Graphics are used in most of these items. Items requiring three-dimensional graphics must be realistic and include verbal descriptions. Items should be set in a real-world context. Answer options may be left in terms of π.	• Calculate the circumference and area of circles given formulas • Calculate perimeter and area of triangles and quadrilaterals given formulas • Calculate the volume and surface area of rectangular prisms given formulas	• select and apply formulas to determine circumference or area of circles given either diameter or radius • produce answers in terms of pi and using approximations of pi • select and apply formulas for area and perimeter of triangles and quadrilaterals • combine formulas to solve composite area problems • select and apply formulas for surface area and volume of rectangular prisms	• radius • diameter • circumference • in terms of pi • quadrilateral • base • height (altitude) • rhombus • trapezoid • parallelogram • prism • lateral area • surface area	• Use the formula C=πd or C=2πr to find the circumference of a circle with a radius of 3.2 cm. Leave your answer in terms of pi. • Use the formula A=½bh to find the area of a triangle with a height of 7 inches and a base of 12 inches. • Use the formula V=lwh to find the volume of a rectangular prism that is 2 cm x 3 cm x 5cm.
	8.M.2.1.5 Convert units of measurement within each system in problem solving situations. CL: C Calc: YES Content Limit: All conversions must be within the same system of measurement. Customary units may include inches, feet, yards, miles, ounces, pounds, tons, fluid ounces, cups, pints, quarts, and gallons. Metric prefixes may include milli-, centi-, and kilo- with base units of grams, liters, and meters. Time units may include years, months, weeks, days, hours, minutes, and seconds. Items should be set in a real-world context.	• Convert units of measurement within each system in problem solving situations	• examine units of measure to determine agreement • determine appropriate unit to use • convert measurments	• see 8.M.2.1.1	• Sal has a garden that is 5 yards by 8½ feet. He wants to put a fence around it. How many feet of fence does he need to buy?
	8.M.2.1.6 Solve problems involving area of circles and the perimeter and area of rectangles and triangles. CL: C Calc: YES Content Limit: Graphics should be used in most of these items, as appropriate. Items should be set in a real-world context. Measurements may be in either metric or customary units. Problems may include shapes that are formed by a combination of two shapes.	• Solve problems involving area of circles and the perimeter and area of rectangles and triangles.	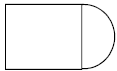• apply concepts of area and perimeter to solve real world problems		• Kendall needs to paint this basketball key which is made up of a rectangle and a semicircle. The length of the rectangle is 18 feet and the width is 12 feet. What is the area of the space that needs to be painted
	8.M.2.1.7 Use appropriate vocabulary and notations. CL: Calc: Content Limit: Assessed in the classroom, not on the ISAT.	• Use appropriate vocabulary and notations	• determine appropriate labeling of perimeter, area and volume • communicate using correct mathematical terminology
Goal 2.2: Apply the concepts of rates, ratios, and proportions.	8.M.2.2.1 Use rates, proportions, ratios, and map scales in problem-solving situations. CL: C Calc: YES Content Limit: Items involving rate should not be limited to time/distance problems, but should include other rated measures (e.g., rates of change for temperature as it changes throughout the day or speed as the rate of change in distance over time, and other derived measures). Items may require students to demonstrate knowledge of proportional relationships in scale drawings or solve real-world problems, including distance, using a scale drawing. There should be no more than two conversions within one dimension per item (e.g., years to seconds is considered four conversions: year-day-hour-minute-second). Measurements may be in either metric or customary units. Items should involve interpreting and applying various scales including those based on number lines , graphs, models, and maps. Scales should use only rational numbers. Items should be set in a real-world context. Graphics are used in most of these items.	• Use rates, proportions, ratios, and map scales in problem-solving situations.	• relate data as rates, ratios, and proportions • use proportions to solve problems involving scale • convert units of measurement as necessary	• proportion • ratio • scale drawing • unit rate	• During a 7.5 hour drive, a car's odometer (milage indicator) starts at 18,560 miles and ends at 18,980 miles. What is the car's rate of travel?
				• proportion • ratio • scale drawing • unit rate
	8.M.2.2.2 Determine unit rates in real-world situations. CL: C Calc: YES Content Limit: Situations must be real-world applications such as gas mileage, speed, growth, etc. Rates given in the problem should be equivalent unit rates equal to a whole number or a terminating decimal .	• Determine unit rates in real-world situations	• write and use unit rates to solve problems	• unit rate	• A 32 ounce container of yogurt costs $2.69. An 8 ounce container of yogurt cost $0.75. Find the unit costs of each container. Which is the better buy?
Goal 2.3: Apply dimensional analysis.	8.M.2.3.1 Illustrate the interrelationship of measurement units through dimensional analysis conversions. CL: C, D Calc: YES Content Limit: Customary units may include inches, feet, yards, miles, ounces, pounds, tons, fluid ounces, cups, pints, quarts, and gallons. Metric prefixes may include milli-, centi-, and kilo- with base units of grams, liters, and meters. Time units may include years, months, weeks, days, hours, minutes, and seconds. No more than two conversion ratios should be used in any item	• Demonstrate the relationship between measurement units through dimensional analysis conversions	• identify common unit conversions • use appropriate conversion ratios to relate equivalent measurement units	• conversion ratio (factor)	• Use dimensional analysis to convert 9 kilometers to meters. • Use the correct conversion factor to convert 70 ounces to pounds.
				• conversion ratio (factor)