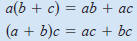Properties of Real Numbers
P.2 Properties of Real Numbers
What you should learn:
• How to identify and use the
properties of real numbers
• How to develop additional
properties of real numbers
Why you should learn it:
Understanding properties of real
numbers will help you to understand
and use the properties of
algebra .
Mathematical Systems
In this section, you will review the properties of real numbers. These
properties
make up the third component of what is called a mathematical system. These
three components are a set of numbers, operations with the set of numbers, and
properties of the numbers (and operations).
Figure P.8 is a diagram that represents different
mathematical systems. Note
that the set of numbers for the system can vary. The set can consist of whole
numbers, integers, rational numbers , real numbers, or algebraic expressions.
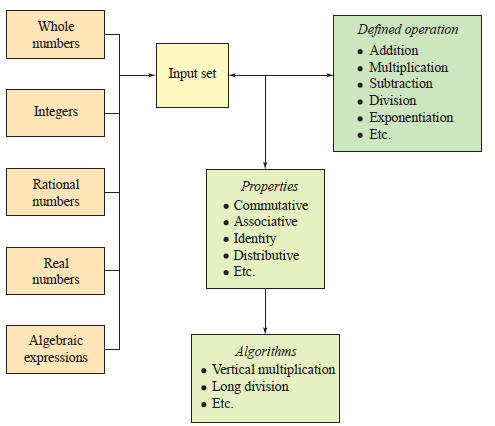
Figure P.8
Basic Properties of Real Numbers
For the mathematical system that consists of the set of real numbers
together with
the operations of addition, subtraction, multiplication, and division, the
resulting
properties are called the properties of real numbers. In the list on page 17, a
verbal description of each property is given, as well as one or two examples .
Properties of Real Numbers
Let a, b, and c represent real numbers.
| Property | Verbal Description |
| Closure Property of Addition a + b is a real number. Closure Property of Multiplication ab is a real number. Commutative Property of Addition a + b = b + a Commutative Property of Multiplication a٠b =b٠a Associative Property of Addition (a + b) + c = a + (b + c) (ab)c = a(bc) |
The sum of two real numbers is a real number. Example: 1 + 5 = 6 and 6 is a real number. The product of two real numbers is a real number. Example: 7٠ 3 = 21 and 21 is a real number. Two real numbers can be added in either order . Example:2 + 6 = 6 + 2 Two real numbers can be multiplied in either order. Example:3٠(-5) = -5٠3 When three real numbers are added, it makes no difference which two are added first. Example:(1 + 7) + 4 = 1 + (7 + 4) When three real numbers are multiplied, it makes no difference which two are multiplied first. Example:(4٠3)٠9 = 4٠(3٠9) Multiplication distributes over addition. Examples:  The sum of zero and a real number equals the number itself. Example:4 + 0 = 0 + 4 = 4 The product of 1 and a real number equals the number itself. Example:5٠1 = 1٠5 = 5 The sum of a real number and its opposite is zero. Example:5 + (-5) = 0 The product of a nonzero real number and its reciprocal is 1. Example:  |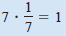
The operations of subtraction and division are not listed
above because they fail
to possess many of the properties described in the list. For instance,
subtraction and division are not commutative. To see this, consider 4 - 3 ≠ 3 -
4
and 15 ÷ 5 ≠ 5 ÷15
Similarly, the examples 8 - (6 - 2) ≠ (8 - 6) - 2
and 20 ÷ (4 ÷ 2) ≠ (20
÷ 4) ÷ 2 illustrate the fact that
subtraction
and division are not associative.
Example 1 Identifying Properties of Real Numbers
Name the property of real numbers that justifies each statement. (Note: a
and b
are real numbers.)
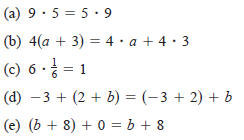
Solution
(a) This statement is justified by the Commutative Property of Multiplication.
(b) This statement is justified by the Distributive Property.
(c) This statement is justified by the Multiplicative Inverse Property.
(d) This statement is justified by the Associative Property of Addition.
(e) This statement is justified by the Additive Identity Property.
Example 2 Identifying Properties of Real Numbers
The area of the rectangle in Figure P.9 can be represented in two ways: as
the area
of a single rectangle, or as the sum of the areas of the two rectangles.
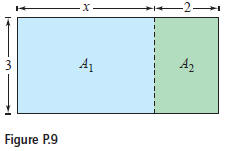
(a) Find this area in both ways.
(b) What property of real numbers does this demonstrate?
Solution
(a) The area of the single rectangle with width 3 and length x + 2 is
A = 3(x + 2)
The areas of the two rectangles are
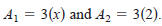
The sum of these two areas represents the area of the
single rectangle. That is,
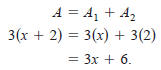
(b) Because the area of the single rectangle and the sum
of the areas of the two
rectangles are equal, you can write 3(x + 2) = 3x + 6 This demonstrates
the Distributive Property.
To help you understand each property of real numbers, try
stating the properties
in your own words.
Example 3 Using the Properties of Real Numbers
Complete each statement using the specified property of real numbers.
(a) Multiplicative Identity Property
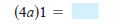
(b) Associative Property of Addition
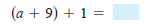
(c) Additive Inverse Property
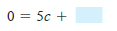
(d) Distributive Property
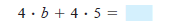
Solution
(a) By the Multiplicative Identity Property, you can write
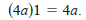
(b) By the Associative Property of Addition, you can write
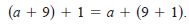
(c) By the Additive Inverse Property, you can write
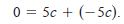
(d) By the Distributive Property, you can write
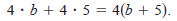
Additional Properties of Real Numbers
Once you have determined the basic properties of a mathematical system
(called
the axioms of the system), you can go on to develop other properties of the
system. These additional properties are often called theorems, and the formal
arguments that justify the theorems are called proofs. The list on page 20
summarizes several additional properties of real numbers.
Example 4 Proof of a Property of Equality
Prove that if a + c = b + c, then a = b. (Use the Addition
Property of
Equality.)
Study Tip
Notice in Example 4
how each step is justified from
the previous step by means of a
property of real numbers.
Solution
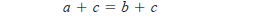Write original equation.
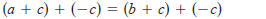Addition Property of Equality
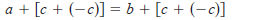Associative Property of Addition
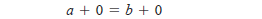Additive Inverse Property
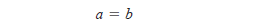Additive Identity Property
Additional Properties of Real Numbers
Let a, b, and c be real numbers.
| Properties of Equality | Verbal Description |
| Addition Property of Equality If a = b then a + c = b + c Multiplication Property of Equality If a = b then ac = bc, c ≠ 0 Cancellation Property of Addition If a + c = b + c then a = b Cancellation Property of Multiplication If ac = bc and c ≠ 0, then a = b Reflexive Property of Equality a = a Symmetric Property of Equality If a = b then b = a Transitive Property of Equality If a = b and b = c then a = c |
Adding a real number to each side of a true
equation produces another true equation. Multiplying each side of a true equation by a nonzero real number produces another true equation. Subtracting a real number from each side of a true equation produces another true equation. Dividing each side of a true equation by a nonzero real number produces another true equation. A real number always equals itself. If a real number equals a second real number, then the second real number equals the first. If a real number equals a second real number and the second real number equals a third real number, then the first real number equals the third real number. |
| Properties of Zero | Verbal Description |
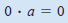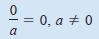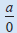Multiplication Property of Zero  Division Property of Zero  Division by Zero Is Undefined  is undefined. |
The product of zero and any real number is zero. If zero is divided by any nonzero real number, the result is zero We do not define division by zero. |
| Properties of Negation | Verbal Description |
Multiplication by -1 Placement of Negative Signs 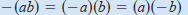 Product of Two Opposites  |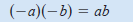
The opposite of a real number a can be obtained
by multiplying the real number by -1 The opposite of the product of two numbers is equal to the product of one of the numbers and the opposite of the other. The product of the opposites of two real numbers is equal to the product of the two real numbers. |
| Prev | Next |