Quadratic Functions
define quadratic function. A quadratic function is a function defined by a
quadratic polynomial ,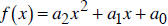 
where
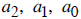constants
with 
or ( more commonly )
where a, b, c
constants with a ≠0. E.g.,
y = -2x2+ 3x -1.
define quadratic- like functions . Some functions have a similar form to quadratic
functions, e.g.,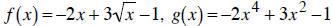 
, and
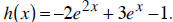Each of these functions can be transformed
into a
quadratic function by using an appropriate substitution, e.g., in g we can
substitute y = x2 to get
g(y) =-2y2+ 3y -1. We can now find the zeros
of 
by first finding the zeros of
g (y) =-2y2+ 3y -1
and we find y =1, y =1/2 .We back substitute for y to get
.x2 =1, x2 = 1/2.
Solving for x , we get x = ±1,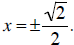
graphs of quadratic functions, parabola, vertex, axis of symmetry. Graphs of
quadratic functions are curves called parabolas. Every parabola has two
important features: an axis of symmetry and a vertex. The vertex is either
a global maximum or minimum point and the axis of symmetry is a
vertical line that passes through the vertex. The graph of every quadratic
function also has a y- intercept and either zero, one or two x -intercepts.
Find the equation of the parabola with a vertical axis of symmetry that
passes through the points (1,0), (2,9), and (-3,4).
translations of 
vertex quadratic form. Draw graph of
We have
sketched the graph of 
previously by
converting the
equation to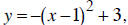 
where the vertex is (1,3). This
suggests
another quadratic form (called the vertex form), i.e.,
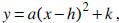
where (h, k) is the vertex and x = h is the equation of the axis of
symmetry. If a > 0, the parabola opens upward; if
a < 0, it opens
downward. The vertex form is useful for finding the vertex and the axis of
symmetry easily.
vertex form of quadratic functions. We can find general formulas for calculating
the vertex and axis of symmetry for
by completing its
square, i.e., =
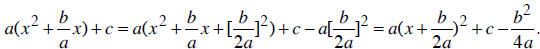
This is now in vertex form and the vertex is the point
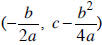and
the axis of symmetry is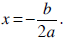 
Find the equation whose
graph is a parabola with vertex
(4,-3), a vertical axis of symmetry, and
passes through the point
(5,-6) .
factored form of quadratic functions, discriminant. The quadratic formula,
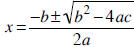, calculates zeroes of the function
These zeroes (or roots of the equation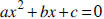 
) may be real or
complex . Let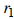 
and
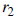represent the roots of the equation, then we can
rewrite f(x) as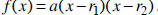 
Early we stated that the roots,
and 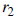, could be two distinct real roots, one repeated real root, or two
complex roots. The discriminant,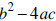 
, will determine what type of
roots we have. If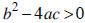 
, then we have two distinct real roots. If
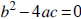, one repeated real root. If
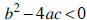, two complex roots.
Why? Notice that complex roots always occur in conjugate pairs, i.e.,
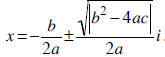. Find the quadratic function whose graph has
zeros at
x = -5 and x = 3 and a y-intercept at (0, 30). Using the general
form, substitute the points
(-5,0), (3,0), and
(0,30)
for
x and y. We obtain three functions:
25a - 5b + c = 0
9a + 3b + c = 0
c = 30
Solving for A, B, and C, A = -2, B = -4, and C = 30. So,
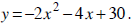
Using the vertex form. We know that h lies on the axis of symmetry,
which is the midpoint of the two zeros, so
h = -1. So,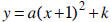 
Again, after substituting our three points, we obtain 3 functions:
16a + k = 0
16a + k = 0
a + k = 30
Solving for a and k, we obtain
a = -2 and k = 32. So,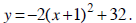 
Using the factored form,
. We know
and
.
Therefore,
y = a(x +5)(x - 3) . Substituting the point (0, 30), we obtain
-15a = 30, so a = -2 and
y = -2(x +5)(x - 3) . Show that
compute vertex and axis of symmetry. The axis of symmetry is
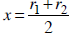(the
average of the two roots). The y- value of the vertex can be found by
substituting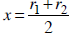
into
f (x) and calculating its value,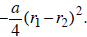 
Therefore, the vertex is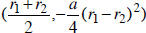 
. Compute the vertex and axis
of symmetry of
y = -2(x +5)(x - 3) .
compute intercepts. To find the x-intercepts of the graph of any function f (if
they
exist), we solve the equation
f (x) = 0. To find the y-intercept, we
calculate the value
f (0) . Find the intercepts of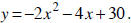 
application. Find the equation of the tangent lines to the circle
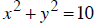from
the point
(1,-7) .
| Prev | Next |