Using the History of Calculus to Enrich our Teaching

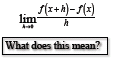
Easier question:

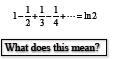
More basic:

What do we mean when we say that two irrational
numbers are equal ?

Euclid answers this question (ca. 300 BC), building on
earlier work of Theaetetus of Athens (417–369 BC) and
Eudoxus of Cnidus (408–355 BC).

Even more basic: What do we mean by an irrational
number?

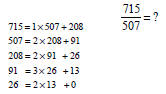
This process must end after a finite
number of steps because the remainders
are strictly decreasing.

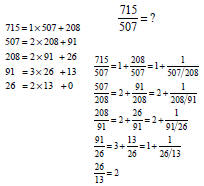
The quotients will
be the same
regardless of which
equivalent fraction
we use.

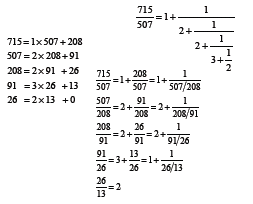
Every ratio of integers corresponds to a
unique finite sequence of integers.

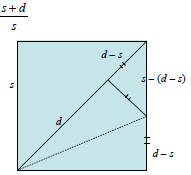
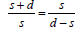
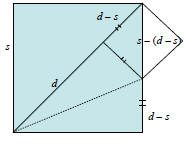
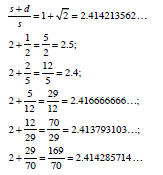
Eudoxus:

α = β if given any rational number m/n:
m < nα implies m < nβ, m/n < α 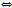m/n < β
m = nα implies m = nβ, m/n = α 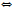m/n = β
m > nα implies m > nβ, m/n > α 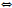m/n > β

Equivalent to a Dedekind cut: the set of rational
numbers less than α is the same as the set of
rational numbers less than β.

Eudoxus is also credited with being the first to use the
method of exhaustion for finding areas and volumes,
which would be used to great effect by Archimedes
(287–212 BC).

There is no such thing as an infinite summation . When
we write an infinite series, it is simply shorthand for an
infinite sequence of finite sums :

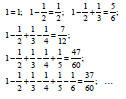
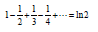
This means: Pick any rational number m/n less than
ln 2.
From some point on, all of the finite sums are > m/n.
Pick any rational number m/n larger than ln 2.
From some point on, all of the finite sums are < m/n.

Pick any number b larger than f ´(x); for all h sufficiently
close to (but not equal to) 0, [f(x+h) – f(x)]/h < b.

Pick any number a smaller than f ´(x); for all h sufficiently
close to (but not equal to) 0, [f(x+h) – f(x)]/h > a.

ε is the distance above or below f ´(x). δ is a limit on the
distance between h and 0 that will guarantee that [f(x+h) –
f(x)]/h is within this distance of f ´(x).