What's the Point

In the last section, we found the equation of a line when we were given two points
that were on the line. In that case, one of the points was always on the y-axis, so
that we always knew the “starting point” or y- intercept of the line . In this case, we
were able to find an equation for our line.

Suppose we didn’t know what our starting point was? Suppose we did know some
of the other points on the line, just not our starting points. Could we find the
equation of the line that contains those points? How many points would we need
before we had enough information to determine the equation of the line?

Team Work

As a team, discuss how many points are needed to have enough information to
determine the equation of a line. If you had that many points, what would you do
to find the equation of the line that contains them. Each team should create some
conjectures for these questions, and report them to the class.

Class Work

As a class, compile all of the team conjectures about how many points are needed
to determine the equation of a line, and about how to find the equation of a line
once you had that many points.

What did the class decide about the number of points that are needed to determine the equation of a line? Later in your mathematical careers, you will study geometry and find out much more about lines, and what we mean by “straight line”. For now, let’s concentrate on the following diagram.

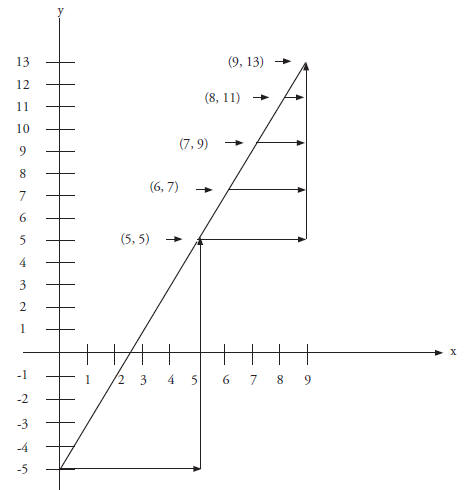
There are five points indicated on the diagram. Would we get a different value for the slope of the line if we picked different points? Let’s try some and see. We’ll use the following equation for the slope m.

m = difference in y values ⁄difference in x values =

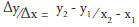
Suppose we pick the points (5, 5) and (9, 13). What would the value for m for these two points? We’ll make (x₁,y₁) = (5,5) and (x₂,y₂) = (9,13). Then we get

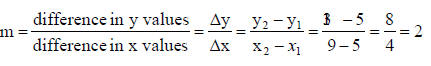
How about if we pick (x₁,y₁) = (8,11) and (x₂,y₂) = (6,7)? Then we get

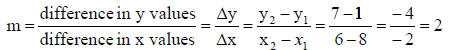
In fact, it wouldn’t matter which two points we picked. We would always get a slope equal to two for this line. Try a few more pairs of points to experiment for yourself.

In geometry, you will find precisely why this is true. It has to do with a property of certain types of triangles that are called “similar” to each other. Can you see the triangles in the diagram formed by looking at the various Δx and Δy values for pairs of points on the line? All of these triangles are similar to each other. Triangles formed in this way from points on a line will always be similar to each other.

We’ll use this property to find an important form of the equation for a line. Since we can pick any points at all and we would always get a slope of 2, let’s set (x₁,y₁) = (5,5) and then leave (x₂,y₂) as variables. Then we get the equation,

Since there really aren ’t two points anymore, we’ll drop the subscripts, and we’ll write

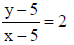
If we multiply both sides of this equation by x-5, we’ll get

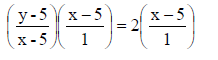
Since we get

y-5 = 2(x-5)

Since this equation contains the information about the slope of the line and contains the coordinates of a point , this is called the point-slope equation of a line. Since we could have used any point for (x₁, y₁), sometimes the point slope equation is written as

y - y₁ = m(x - x₁)

where m is the slope of the line.
Let’s find the point-slope equation of the line using another point. We could pick (x₁,y₁) = (8,11) and then our equation would be

y - 11 = 2(x - 8)

Team Work

As a team, discuss reasons why you think that we came up with two different equations for the same line. Did we make a mistake? Can the same line have more than one equation?

Class Work

As a class, compile all of the team discussions about the reasons why we came up with two different equations for the same line. Did we make a mistake?
What did your class decide? Did we make a mistake?
Here are the two equations we found for the line.

y-5 = 2(x-5)
y-11 = 2(x-8)

Let’s “ solve for y ” in each equation. What “solve for y” means is that we will try and get the y variable all by itself on one side of the equation. To do this, we will multiply through on the right hand side of each equation using the distributive law .

Next we’ll add the additive inverse on both sides to get y by itself on the left.

That’s the solution to the mystery ! Both equations lead to the same slope-intercept equation for the line. The two equations just looked different at first, but had the same information in them the entire time. Let’s start with another point and verify this fact once again.
This time we’ll let (x₁,y₁) = (7,9). Then our point-slope equation becomes

y - 9 = 2(x-7)

Following the same steps as before ,
y-9 = 2x – 14
y-9+9=2x-14+9
y=2x-5

Same equation once again.

Team Work

Find a point-slope equation for the lines that caontain the given points.. Next use the point-slope equation to solve for the slope-intercept equation for the line.

1.) (x₁, y₁) = (1 , 2) and (x₂, y₂) = (3, 8) .
a.) Find the slope of the line, m.

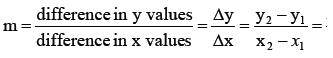
b.) Find a point-slope equation for the line
c.) Solve for the slope-intercept equation of the line.

2.) (x₁, y₁) = (5 , 2) and (x₂, y₂) = (3, 10) .
a.) Find the slope of the line, m.

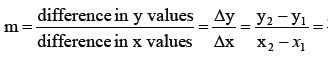
b.) Find a point-slope equation for the line
c.) Solve for the slope-intercept equation of the line.

3.) (x₁, y₁) = (-1 , 2) and (x₂, y₂) = (2, 11).
a.) Find the slope of the line, m.

b.) Find a point-slope equation for the line
c.) Solve for the slope-intercept equation of the line.

4.) (x₁, y₁) = (4 , 3) and (x₂, y₂) = (1, 6).
a.) Find the slope of the line, m.

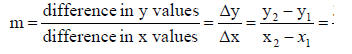
b.) Find a point-slope equation for the line
c.) Solve for the slope-intercept equation of the line.