Calculus Homework

I. The following questions refer to the function given in problem #2 page 169.

A. Quadratic functions are those of the form f(x)=ax²+bx+c, where a, b and c are
numbers, and a is nonzero. What are a, b and c in this problem?

B. A blue box at the bottom of page 46 tells us how to find the coordinates of the
vertex of a quadratic function and whether the graph opens upward or downward.
Use it to find the vertex of the quadratic function given in problem #2. Is the
parabola opening upward or downward?

C. Find any x- and y- intercepts . (If you need a reminder, the True/False problem #60
page 16 is true, and holds for any function, not just a linear one . See also
Example 5, page 47.)

D. Graph the function, on the domain [-6,6] by hand. Draw your graph large enough
to see (at least 1/4 page ).

E. Do problem #2 page 169.

F. Read the green note on page 163. On your graph, draw the secant line whose
slope is equal to the average you calculated in part (E).

G. Do problem #14 page 169 (which deals with the same formula as #2). Show your
work, carefully. (See Example 3 page 166).

II. The following exercises refer to the function given in problem #18 page 169. Write
the formula for f(x) at the top of the problem, for easy reference.

A. Find f(3).

B. Find f(3+h). Your answer will have h' s in it. (Stuck? See Example 4(c) page 37,
and the paragraph at the top of page 38).

C. Find a formula for the average rate of change for the function f between x=3 and
x=3+h. Again your answer will have h' s in it. Answer in a sentence, "The
average rate of change for ... is ..."Use the " lim" notation to write the formula for
the instantaneous rate of change of f at x=3.

D. Copy the following table onto your homework and fill in the average rates of
change for each value of h , rounding to three decimal places.

h	Average rate of change between x=3 and x=3+h [f(3+h)-f(3)]/h
Approaching 0 from below
Approaching 0 from above

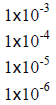E. What is the instantaneous rate of change of f at x=3?

III. Do problem #28 page 171.

A. Parts (a) and (b): show and explain your work clearly, and write your final
answers as sentences. In part (d), of course, write sentences.

B. Use Excel to do part (c).

• Open Microsoft Excel. In the file menu, choose Page setup.... Click on Landscape,
and click OK.

• Type “Days since May 25” into cell A1. To adjust the column width, put your curser
over the line between the A and the B at the top of the first two columns . Your curser
will look like a vertical line with arrows pointing to the left and right. Click and drag
the border between the two columns to the desired width. Type “Body mass (kg)”
into cell B1, and adjust the column width as necessary.

Excel cannot graph a smooth curve, as a graphing calculator does. Instead, it can plot
points and connect the dots. If you put enough dots, it will look like a smooth curve.
Since you want to graph this function on the domain [5,125], in column A, you will enter
t values starting at 5 and ending at 125. The more t values you include between 5 and
125, the smoother the curve will look. Here, the instructions direct you to count by 5’s,
entering the numbers 5, 10, 15, etc... Fortunately Excel will do the counting for you.

• Type 5 into cell A2. In Cell A3, type “=A2+5” (not including the quotes), and hit
enter or click check in the formula bar. The number 10 should appear in cell A3,
since the equals sign tells excel to do a computation. Here is how to fill this formula
down to cell A26. With cell A3 selected, put your curser on the bottom right hand
side of the cell. Your curser should change into a small black plus shape. Hold the
mouse so that the curser shows that plus shape, press the left mouse button, and
holding it down, drag to cell A26. You should now see the numbers 5 through 125 in
these cells, counting by 5’s.

• Next, we want to calculate M(5) and put the answer in cell B2. To do this, you will
type the formula for M(t) with the t’s replaced by A2. So in cell B2, type
“=27.5+0.3*A2-0.001*A2^2.” Fill this formula down to cell B26. Now in columns A
and B, you have a table of values for the function M(t).

• Save your work.

You will now graph this function.

• Select cells A2 through B26.

• Choose Chart... from the Insert menu. A dialog box called “Chart Wizard – Step 1
of 4 – Chart Type” will appear. Click on x-y scatter plot. There are five chart subtypes
shown. Click on the third one (with curve but without the dots). Click Next.

• The title of the dialog box changes to Step 2 of 4 – Chart Source Data. We won’t use
these options at this time. Click Next.

• Step 3 of 4 – Chart Options. Click on the Titles tab, if it is not already selected. Type
“Mass of Bighorn Yearlings” for the title of your chart. For Value (X) axis, type
“Days since May 25,” and for Value (Y) axis, type “Body Mass (kg).” Click the tab
at the top of the dialog box that says Gridlines. Check Major gridlines under Value
(X) axis and Value (Y) axis. Click Next.

• Step 4 of 4 – Chart Location. This dialog box allows you to choose whether the chart
will appear in a new window, or as a part of the worksheet containing the data. Mark
the “As an object in” radio button, if it is not already marked. Click Finish.

• Click on the box in the chart that says Series 1. Black squares should appear around
the box. Press Delete.

Now you will change the scale on the y-axis so that it shows the range [25,65], as
specified in the problem.

• Right click on the y-axis of the chart. Choose Format axis from the drop down menu.
Click the tab that says Scale at the top of the dialog box. The checkboxes under the
word Auto indicate that Excel is automatically choosing these values. Uncheck the
boxes before “Maximum,” “Minimum” and “Value (X) axis crosses at.” Enter 25 for
 the Minimum, 65 for the Maximum, and 25 for the Value (X) axis crossing. The
major unit and minor unit checkboxes should be checked. Click OK.

• Right click on the x-axis of the chart. Change the scale so that the range [5,125]
shows, as specified in the problem. Click OK.

• Save your work.

• Click on the white background of the chart. Choose Print... from the File menu.

IV. Write a paragraph that explains your answer to problem #22, page 169. Write your
answer to the question in a topic sentence, and use the rest of the paragraph to support
your claim.