Dividing Fractions
Class Activity 0C: "How Many in One Group?" Frac-
tion Division Problems
Class Activity 0D: Are These Division Problems?
Exercises for Section 0.1 on Dividing Fractions
1. Write a "how many groups?" story problem for
Use the story
problem and a diagram to help you solve the problem .
2. Write a "how many in one group?" story problem for
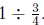Use the
situation of the story problem to help you explain why the answer is
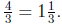
3. Annie wants to solve the division problem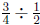 
by using the following
story problem:
I need 
cup of
chocolate chips to make a batch of cookies.
How many batches of cookies can I make with
of a cup of
chocolate chips?
Annie draws a diagram like the one in Figure 3. Explain why it would
be easy for Annie to misinterpret her diagram as showing that
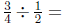
How should Annie interpret her diagram so as
to conclude that
4. Which of the following are solved by the division problem
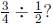For
those that are, which interpretation of division is used? For those that
are not, determine how to solve the problem, if it can be solved.
(a) of a bag of jelly
worms make a cup. How
many cups of jelly
worms are in one bag?
|
1/2 cup makes one batch |
 |
1/4 cup left |
Figure 3: How Batches of Cookies Can We Make With
of a Cup of Chocolate
Chips if 1 Batch Requires
Cup of Chocolate
Chips?
(b)
of a bag of jelly
worms make
a cup. How many bags
of jelly
worms does it take to make one cup?
(c) You have of a bag
of jelly worms and a recipe that calls for
of
a cup of jelly worms. How many batches of your recipe can you
make?
(d) You have of a cup
of jelly worms and a recipe that calls for
of
a cup of jelly worms. How many batches of your recipe can you
make?
(e) If of a pound of
candy costs of a
dollar, then how many pounds
of candy should you be able to buy for 1 dollar?
(f) If you have of a
pound of candy and you divide the candy in
,
then how much candy will you have in each portion?
(g) If of a pound of
candy costs $1, then how many dollars should
you expect to pay for
of a pound of candy?
5. Frank, John, and David earned $14 together. They want to divide it
equally, except that David should only get a half share, since he did half
as much work as either Frank or John did (and Frank and John worked
equal amounts). Write a division problem to find out how much Frank
should get. Which interpretation of division does this story problem
use?
6. Bill leaves a tip of $4.50 for a meal. If the tip is 15% of the cost of
the meal, then how much did the meal cost? Write a division problem
to solve this. Which interpretation of division does this story problem
use?
7. Compare the arithmetic needed to solve the following
problems.
(a) What fraction of a 
cup measure is filled when we pour in 
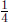cup
of water?
(b) What is one quarter of
cup?
(c) How much more is
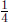cup than cup?
(d) If 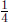cup of water fills
of a plastic
container, then how much
water will the full container hold?
8. Use the meanings of multiplication and division to solve the following
problems.
(a) Suppose you drive 4500 miles every half year in your car. At the
end of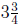 
years, how many miles will you have
driven?
(b) Mo used 128 ounces of liquid laundry detergent in
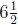weeks. If
Mo continues to use laundry detergent at this rate, how much will
he use in a year?
(c) Suppose you have a 32 ounce bottle of weed killer concentrate.
The directions say to mix two and a half ounces of weed killer
concentrate with enough water to make a gallon. How many gallons
of weed killer will you be able to make from this bottle?
9. The line segment below is
of a unit long. Show
a line segment that
is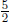 
of a unit long. Explain how this problem
is related to fraction
division.
unit
Answers To Exercises For Section 0.1 on Dividing Frac-
tions
1. A simple "how many groups?" story problem for
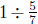is "how many
of a cup of water are in 1 cup of water?" Figure 4 shows 1 cup of water
and shows 
of a cup of water shaded. The
shaded portion is divided
into 5 equal parts and the full cup is 7 of those parts. So the full cup
is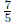 
of the shaded part. Thus there are
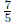of of a
cup of water in 1
cup of water, so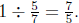 
| 1 cup |  |
of a cup |
 |
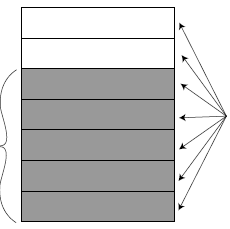 each piece isof the shaded portion |
Figure 4: Showing Why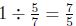 
by Considering How Many
of a Cup of
Water are in 1 Cup of Water
2. A "how many in one group?" story problem for
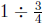is "if 1 ton of
dirt fills a truck
full, then how many tons of dirt will be needed to
fill the truck completely full?". We can see that this is a "how many
in one group?" type of problem because the 1 ton of dirt fills
of a
group (the truck) and we want to know the amount of dirt in 1 whole
group. Figure 5 shows a truck bed divided into 4 equal parts with 3
of those parts filled with dirt. Since the 3 parts are filled with 1 ton
of dirt, each of the 3 parts must contain
of a ton of dirt. To
ll the
truck completely, 4 parts, each containing
of a ton of dirt are
needed.
Therefore the truck takes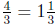 
tons of dirt to
fill it completely, and
so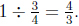
|
the 1 ton of dirt is divided equally among 3 parts |
 truck bed |
4 parts are needed to fill the truck; each part is 1/3 of a ton, so 4/3 tons of dirt are needed to fill the truck |
Figure 5: Showing Why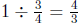 
by Considering How Many Tons of Dirt
it Takes to Fill a Truck if 1 Ton Fills it
Full
3. Annie's diagram shows that she can make 1 full batch of cookies from
her
of a cup of chocolate
chips and that cup of
chocolate chips will
be left over. Because
cups of chocolate chips are left over, it would
be easy for Annie to misinterpret her picture as showing
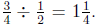
But the answer to the problem is supposed to be the number of batches
Annie can make. In terms of batches , the remaining
cup of chocolate
chips makes of a batch
of cookies. We can see this because 2 quarter-cup
sections make a full batch, so each quarter-cup section makes
of
a batch of cookies. So by interpreting the remaining
cup of chocolate
chips in terms of batches , we see that Annie can make
batches of
chocolate chips, thereby showing that 
not
4. (a) This problem can be rephrased as "if
of a cup of jelly
worms
fill of a bag, then
how many cups fill a whole bag?", therefore
this is a "how many in one group?" division problem illustrating
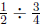, not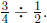 
Since 
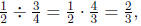there are
of a cup of
jelly worms in a whole bag.
(b) This problem is solved by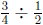 
,according to
the "how many in
each group?" interpretation. A group is a cup and each object is
a bag of jelly worms.
(c) This problem can't be solved because you don't know how many
cups of jelly worms are in
of a bag.
(d) This problem is solved b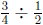y , according to
the "how many
groups?" interpretation. Each group consists of
of a cup of jelly
worms.
(e) This problem is solved by 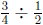, according to
the "how many in
one group?" interpretation. This is because you can think of the
problem as saying that
of a pound of candy fills
of a group
and you want to know how many pounds fills 1 whole group.
(f) This problem is solved by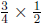 
, not
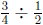. It is dividing in half,
not dividing by .
(g) This problem is solved b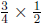y , according to
the "how many
groups?" interpretation because you want to know how many
pounds are in of a
pound. Each group consists of
of a pound
of candy.
5. If we consider Frank and John as each representing one group, and
David as representing half of a group, then the $14 should be dis-
tributed equally among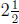 
groups. Therefore, this is a "how many in
one group" division problem. Each group should get
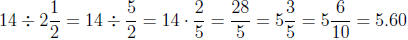
dollars. Therefore Frank and John should each get $5.60
and David
should get half of that, which is $2.80.
6. According to the "how many in one group?" interpretation, the problem
is solved by $4.50 ÷ 0.15 because $4.50 fills 0.15 of a group and we
want to know how much is in 1 whole group. So the meal cost
7. Each problem, except for the first and last, requires
different arithmetic
to solve it.
(a) This is asking:
equals what times ? We
solve this by calculating
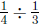, which is
. We can also think
of this as a division problem
with the "how many groups?" interpretation because we want to
know how many of a
cup are in of a cup.
According to the
meaning of division, this is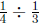 
.
(b) This is asking: what is
of
? We solve this by
calculating
(c) This is asking: what is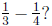 
The answer is
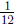which happens to
be the same answer as in part (b), but the arithmetic to solve it
is different.
(d) Since cup of water
fills of a plastic
container, the full container
will hold 3 times as much water, or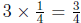 
of a
cup. We can
also think of this as a division problem with the "how many in one
group?" interpretation.
cup of water is put into
of a group.
We want to know how much is in one group. According to the
meaning of division it's 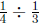, which again is
equal to .
8. (a) The number of
years in 
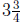years is
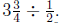There will be that
many groups of 4500 miles driven. So after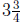
years you will have
driven
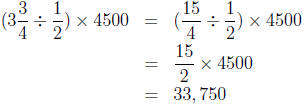
miles.
(b) Since one year is 52 weeks there are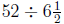 
groups of 
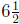weeks
in a year. Mo will use 128 ounces for each of those groups, so Mo
will use
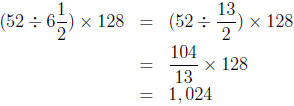
ounces of detergent in a year.
(c) There are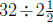 
groups of
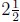ounces in 32 ounces. Each of those
groups makes 1 gallon. So the bottle makes 
gallons
of weed killer.
9. One way to solve the problem is to determine how many
units are in
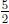
units. This will tell us how many of the
unit long segments to
lay end
to end in order to get the 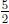unit long
segment. Since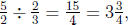 
there are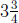 
segments of length
units in a segment of
length 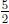units.
So you need to form a line segment that is 3 times as long as the one
pictured, plus another
as long:
| Prev | Next |