Math 112 Practice Test 4

MULTIPLE CHOICE . Choose the one alternative that best completes the statement or answers the question.

Use long division to determine whether the binomial is a factor of f (x).

A) Yes
B) No

Use synthetic division to find the quotient and the remainder.

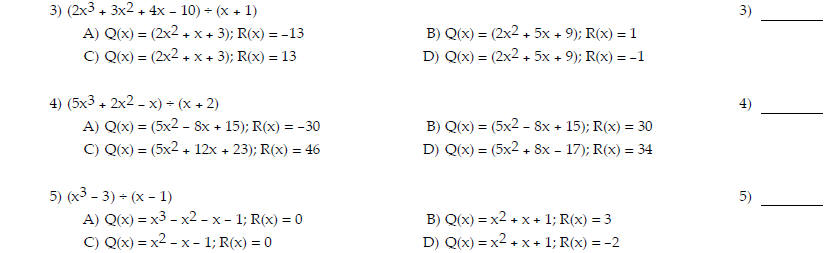
Use synthetic division to find the function value .

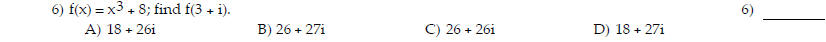
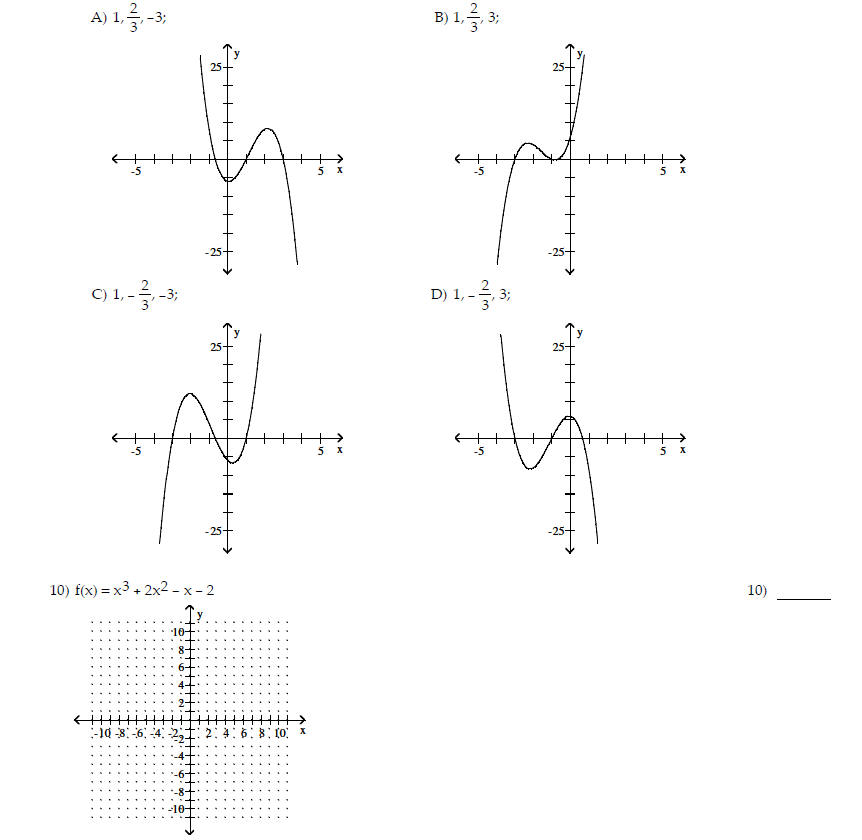
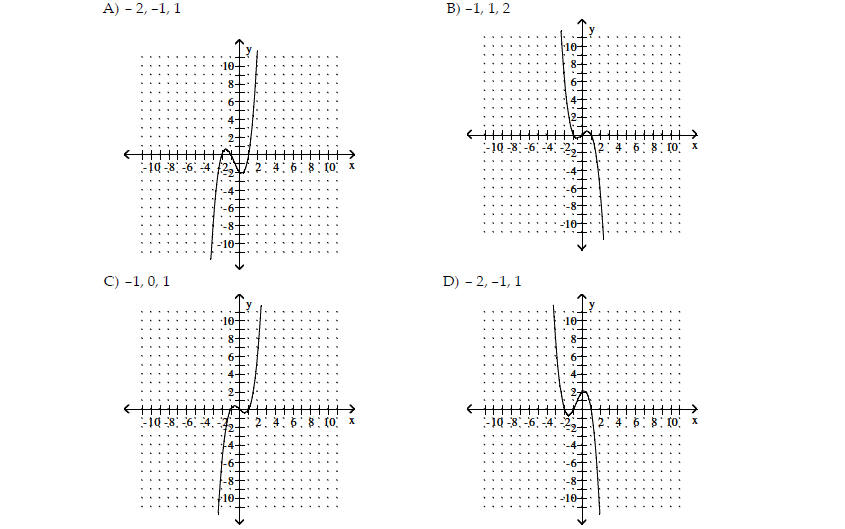
Factor the polynomial f (x). Then solve the equation f(x) = 0.

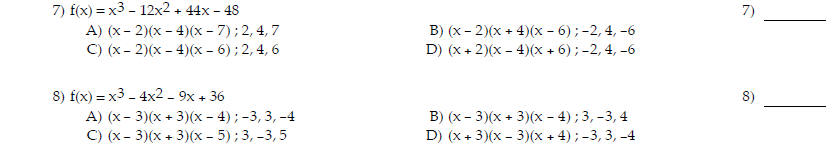
Graph the polynomial function. Use synthetic division and the remainder theorem to find the zeros.

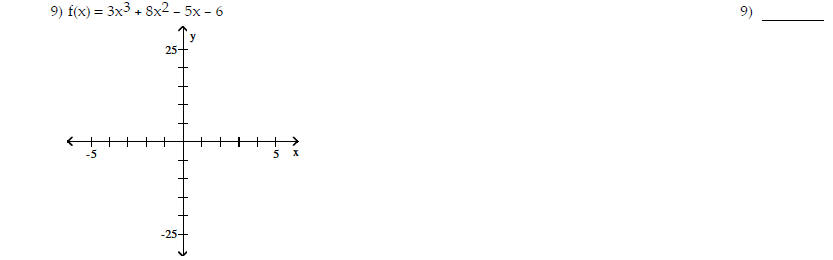
Find the requested polynomial.

11) Find a polynomial function of degree 3 with -1, 2, 4 as zeros.

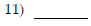12) Find a polynomial function of degree 3 with 4, 2i, -2i as zeros .

Provide the requested response.

13) Suppose that a polynomial function of degree 5 with rational coefficients has -3, 3i, as zeros. Find the other zeros.

14) Suppose that a polynomial function of degree 4 with rational coefficients has 6, 4, 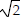as zeros. Find the other zero.

Find a polynomial function of lowest degree with rational coefficients that has the given numbers as some of its zeros.

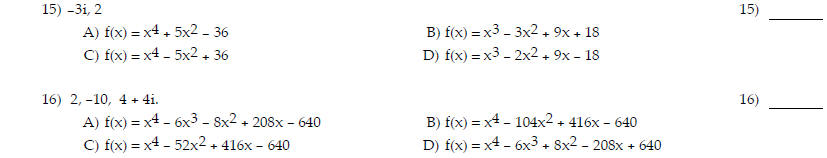
Given that the polynomial function has the given zero, find the other zeros.

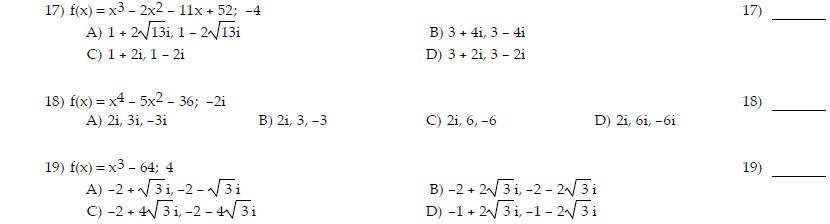
Give all possible rational zeros for the polynomial .

Given the polynomial function f(x), find the rational zeros, then the other zeros (that is, solve the equation f (x) = 0), and
factor f(x) into linear factors .

A) -8, -2, 2; f(x) = (x + 8)(x + 2)(x - 2)
B) , multiplicity 2; - , multiplicity 2;
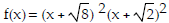
C) , multiplicity 2; -2;

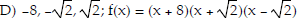
Find only the rational zeros.

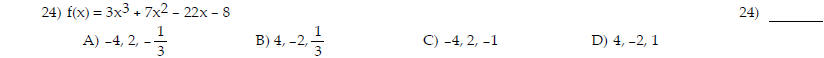
Match the equation with the appropriate graph.

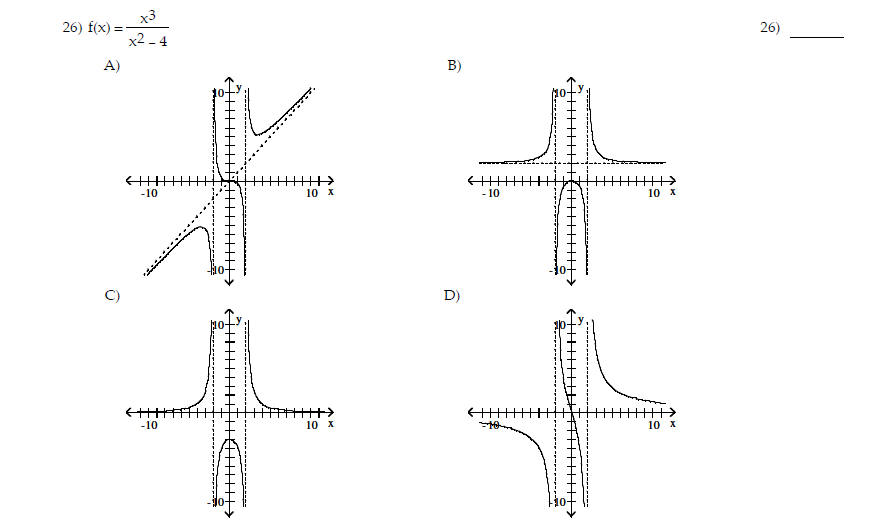
Find the vertical asymptote(s) of the graph of the given function.

Find the horizontal asymptote, if any, of the rational function.

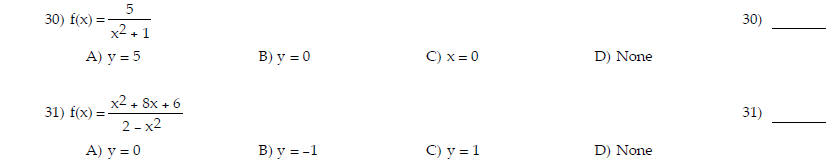
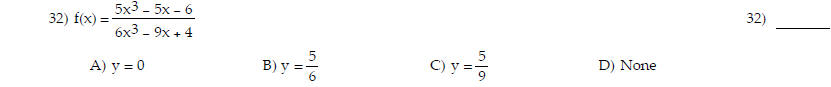
Find the oblique asymptote, if any, of the rational function.

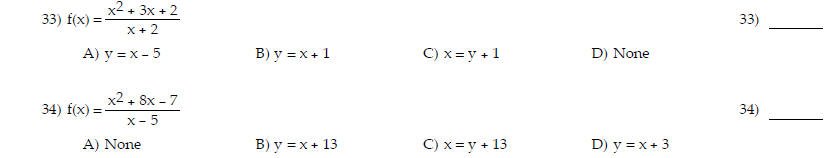
State the domain of the rational function.

Solve the given inequality (a related function is graphed).

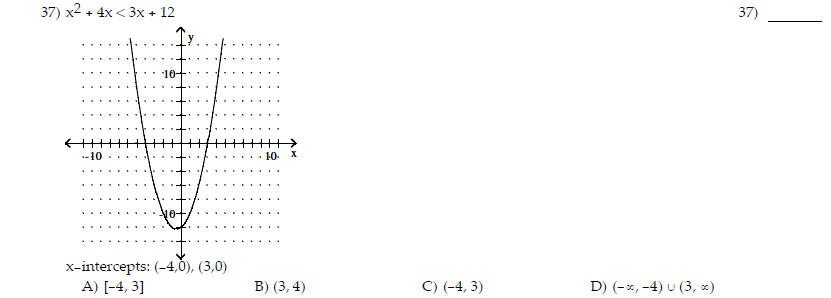
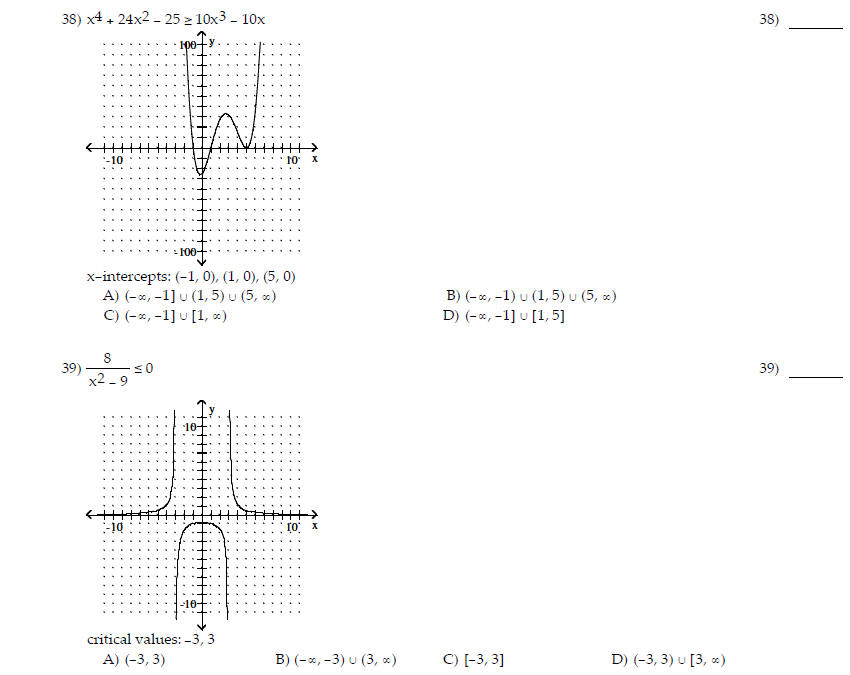
Solve.

Solve the system .

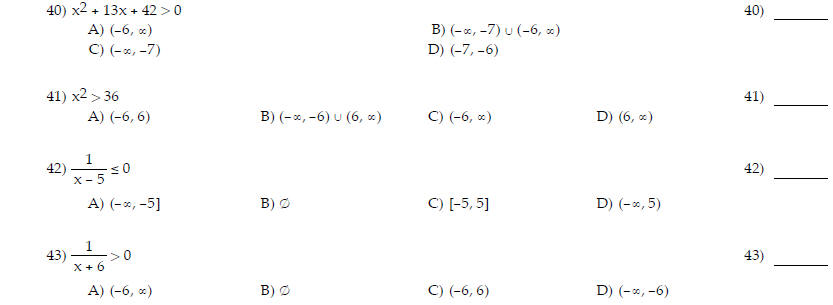
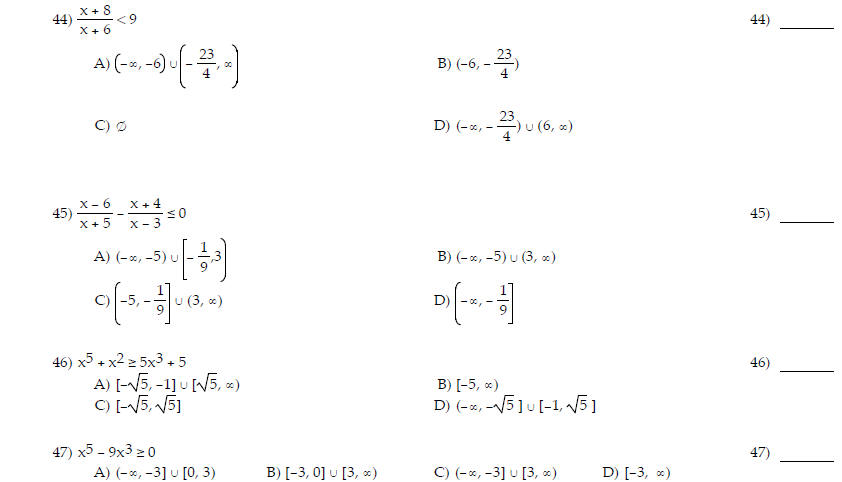
Answer Key
Testname: MATH112 PRACTICE TEST 4 (3.3-3.6, 8.2)

1) A
2) B
3) A
4) A
5) D
6) C
7) C
8) B
9) C
10) A
11) A
12) C
13) A
14) B
15) D
16) C
17) D
18) B
19) B
20) C
21) C
22) C
23) D
24) A
25) B
26) A
27) B
28) A
29) D
30) B
31) B
32) B
33) B
34) B
35) D
36) D
37) C
38) C
39) A
40) B
41) B
42) D
43) A
44) A
45) C
46) A
47) B
48) B
49) C
50) C