Quadratic Regression

The following table lists the tuition cost per quarter for taking 15 credits at Green River Community
College since 1980.

Year	Cost
1980	$102	Year	Cost
1981	102	1994	$432
1982	173	1995	450
1983	173	1996	467
1984	193	1997	486
1985	193	1998	505
1986	233	1999	528
1987	253	2000	547
1988	260	2001	581
1989	274	2002	661
1990	294	2003	714
1991	320	2004	771
1992	333	2005	815
1993	375	2006	862

Use your calculator ’s linear regression function to find a line of best fit for the data. What is
the linear function of best fit? (Hint: It might make things easier if you use ‘years since 1980’ as
the variable x instead of the year number – so 1980 would be x = 0, and 2006 would be x = 26.)

Use the function you found in part 1 to estimate the cost of taking 15 credits at GRCC in 2007.
Also estimate the cost in 2010 and 2020.

Plot both the data and the line on your calculator to see how well it fits. Does it look like a
good fit to you?

Next, use your calculator to find a quadratic regression for the data. What is the quadratic
function of best fit?

Plot the data and your quadratic function on your calculator. How well does it fit? Does it look
like it fits better than the line does?

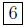Now use the quadratic function to try to predict the cost of taking 15 credits at GRCC in 2007,
2010 and 2020.

Do you think the linear function or the quadratic function gives a better prediction? Why?