College Algebra Test

9. Answer each of the following questions concerning the graph to the lower -right.
(a) Determine the range and domain of the function f from its graph, given to the right below. Use interval notation.
Solution:

Domain = [−3, 3]

Range = [ 0, 2]

(b) State the intervals of increase and decrease.

Interval of increase = [−3, 0]

Interval of decrease = [ 1, 3]

10. The graph of the function y = x^2 is shifted horizontally 2 units to the left, and shifted 4 units vertically upwards.
Write the equation of the resulting function:

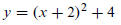
11. Sketch each of the following graphs using transformation methods .

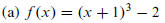Solution: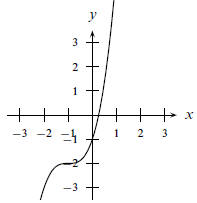

(b) g(x) = |x + 1|.
Solution: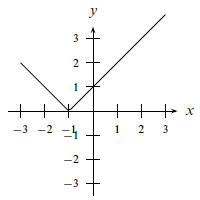

12. Solve the absolute inequality : |1 − 2x| ≥ 6

Solution: We have, again,

\|1 − 2x\| ≥ 6
1 − 2x ≥ 6	or	1 − 2x ≤ −6
−2x ≥ 5	or	−2x ≤ −7
x ≤ −5/2	or	x ≥ 7/2

The solution set is (−∞,−5/2) ∪ [7/2,∞)

13. Answer each of the following concerning piecewise-defined function

(a) (2 pts) Calculate each of the following :

f (4)= −3

f (−3) = 9

(b) (4 pts) Graph the function on the axis to the right. Be
sure to indicate the value of the function at x = 0 on
the graph.

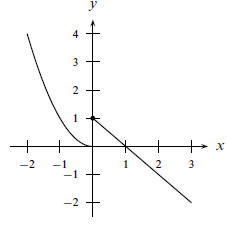
14. Let f (x) = 2x^2 − x and g(x) = 3x + 1, perform the following compositions.

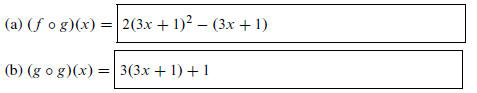
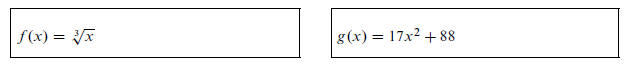
15. Consider the functionFind functions f and g such that

16. Solve the following system using the elimination method . Leave your answer as a solution set.

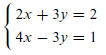
Solution Set ={(1/2, 1/3)}

Solution: We use the elimination method:

2x + 3y = 2	(1)
4x − 3y = 1	(2)
4x + 6y = 4	(3)	multiply (1) by 2
4x − 3y = 1	(2)	copy of equation (2)
9y = 3	(4)	subtract (2) from (3)
y = 1/3	(5)	solve
2x + 3(1/3) = 2		substitute (5) into (1)
x = 1/2		solve!
The solution set is { (1/2, 1/3) }