Properties of Logarithms and Solving Exponential Equations
Properties of Logarithms and Solving Exponential Equations
In Exercises 1-10, use a calculator to
evaluate the function at the given value
p. Round your answer to the nearest
hundredth.
1. f(x) = log 4(x); p = 57.60.
2. f(x) = log 4(x); p = 11.22.
3. f(x) = log 7(x); p = 2.98.
4. f(x) = log3(x); p = 2.27.
5. f(x) = log6(x); p = 2.56.
6. f(x) = log8(x); p = 289.27.
7. f(x) = log8(x); p = 302.67.
8. f(x) = log5(x); p = 15.70.
9. f(x) = log8(x); p = 46.13.
10. f(x) = log4(x); p = 15.59.
In Exercises 11-18, perform each of the
following tasks.
a) Approximate the solution of the given
equation using your graphing calculator .
Load each side of the equation
into the Y= menu of your calculator .
Adjust the WINDOW parameters
so that the point of intersection of
the graphs is visible in the viewing
window. Use the intersect utility
in the CALC menu of your calculator
to determine the x- coordinate of the
point of intersection. Then make an
accurate copy of the image in your
viewing window on your homework
paper.
b) Solve the given equation algebraically ,
and round your answer to the nearest
hundredth.
11. 20 = 3(1.2)x
12. 15 = 2(1.8)x
13. 14 = 1.45x
14. 16 = 1.84x
15. −4 = 0.2x − 9
16. 12 = 2.9x + 2
17. 13 = 0.1x+1
18. 19 = 1.2x-6
In Exercises 19-34, solve the given equation
algebraically , and round your answer
to the nearest hundredth.
19. 20 = ex-3
20. −4 = ex − 9
21. 23 = 0.9x + 9
22. 10 = ex + 7
23. 19 = ex + 5
24. 4 = 7(2.3)x
25. 18 = ex+4
26. 15 = ex+6
27. 8 = 2.73x
28. 7 = ex+1
29. 7 = 1.18x
30. 6 = 0.2x-8
31. −7 = 1.3x − 9
32. 11 = 3(0.7)x
33. 23 = ex + 9
34. 20 = 3.2x+1
35. Suppose that you invest $17,000 at
6% interest compounded daily . How many
years will it take for your investment to
double? Round your answer to the nearest
hundredth.
36. Suppose that you invest $6,000 at
9% interest compounded continuously . How
many years will it take for your investment
to double? Round your answer to
the nearest hundredth.
37. Suppose that you invest $16,000 at
6% interest compounded daily. How many
years will it take for your investment to
reach $26,000? Round your answer to
the nearest hundredth.
38. Suppose that you invest $15,000 at
5% interest compounded monthly. How
many years will it take for your investment
to double? Round your answer to
the nearest hundredth.
39. Suppose that you invest $18,000 at
3% interest compounded monthly. How
many years will it take for your investment
to double? Round your answer to
the nearest hundredth.
40. Suppose that you invest $7,000 at
5% interest compounded daily. How many
years will it take for your investment to
reach $13,000? Round your answer to
the nearest hundredth.
41. Suppose that you invest $16,000 at
9% interest compounded continuously. How
many years will it take for your investment
to double? Round your answer to
the nearest hundredth.
42. Suppose that you invest $16,000 at
2% interest compounded continuously. How
many years will it take for your investment
to reach $25,000? Round your answer
to the nearest hundredth.
43. Suppose that you invest $2,000 at
5% interest compounded continuously. How
many years will it take for your investment
to reach $10,000? Round your answer
to the nearest hundredth.
44. Suppose that you invest $4,000 at
6% interest compounded continuously. How
many years will it take for your investment
to reach $10,000? Round your answer
to the nearest hundredth.
45. Suppose that you invest $4,000 at
3% interest compounded daily. How many
years will it take for your investment to
reach $14,000? Round your answer to
the nearest hundredth.
46. Suppose that you invest $13,000 at
2% interest compounded monthly. How
many years will it take for your investment
to reach $20,000? Round your answer
to the nearest hundredth.
47. Suppose that you invest $20,000 at
7% interest compounded continuously. How
many years will it take for your investment
to reach $30,000? Round your answer
to the nearest hundredth.
48. Suppose that you invest $16,000 at
4% interest compounded continuously. How
many years will it take for your investment
to double? Round your answer to
the nearest hundredth.
49. Suppose that you invest $8,000 at
8% interest compounded continuously. How
many years will it take for your investment
to double? Round your answer to
the nearest hundredth.
50. Suppose that you invest $3,000 at
3% interest compounded daily. How many
years will it take for your investment to
double? Round your answer to the nearest
hundredth.
8.6 Answers
1. 2.92
3. 0.56
5. 0.52
7. 2.75
9. 1.84
11.
a)
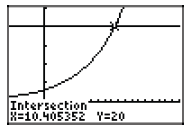
b) 10.41
13.
a)
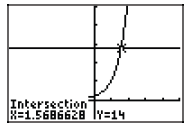
b) 1.57
15.
a)

b) −1.00
17.
a)
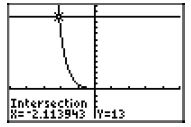
b) −2.11
19. 6.00
21. −25.05
23. 2.64
25. −1.11
27. 0.70
29. 2.55
31. 2.64
33. 2.64
35. 11.55 years
37. 8.09 years
39. 23.13 years
41. 7.70 years
43. 32.19 years
45. 41.76 years
47. 5.79 years
49. 8.66 years
| Prev | Next |