The Slope-Intercept Form of a Line Activity

Introduction

In this module, you will explore the slope- intercept form of a linear equation. You
will see how changes in the slope and y -intercept of a line affect its graph. You
will also learn how to graph equations in slope -intercept form on your own.

The Slope-Intercept Form of a Line (y = m x + b)

The Slope or Steepness

The general slope-intercept form of a line is given by the equation y = m x + b. In
this equation, m represents the slope or steepness of the line.

1. Vary the slope of the line in the Gizmo using the m slider.

a. What do lines with a positive slope look like? (Do they go upward
or downward from left to right?)

b. What do lines with a negative slope look like?

2. Set m to 2. (The quickest way to do this is to click on the value to the right
of the slider, type 2, and press Enter .) Look at the steepness of the line.
Then set m to 0.2.

a. How did the steepness of the line change?

b. Will the line become steeper or less steep if you increase the slope
to 0.5? Use the Gizmo to check your answer.

c. Set m = −2. What does this line look like?

d. Sketch a line with m = −0.5 on the coordinate grid below. Then use
the Gizmo to check your answer.

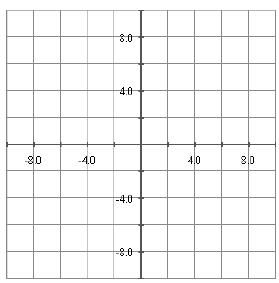
e. Set m = 0. What kind of line is this?

3. Set m = 3. Then click on the Table tab. Set the Min to -5, Max to 5, and
the Step to 1. Then click on Compute to view a table of x and y values.

a. With m = 3, how much does y increase as x increases by steps of
1?

b. What if m = 0.5? Check your answer using the Gizmo.

4. Click on the Controls tab if necessary. You can adjust the slope of the
line by clicking and dragging the line. Click on the line to the right of the y-axis
and drag it up and down to adjust the slope.

a. As you drag up, does the slope of the line increase or decrease?
(For a hint, watch how the m slider moves while you drag the line.)

b. Now use your mouse to click and drag the line to the left of the y-axis.
When you drag up, what happens to the slope of the line? Is
this different from before ? Why or why not?

5. Turn on Show Triangle by clicking in the box. This will allow you to see
the slope in rise over run form.

a. What does the triangle look like when the line is very steep?

b. What does the triangle look like when the slope of the line is very
close to zero ?

c. What is true of the rise and run when the slope is positive?

d. What is true of the rise and run when the slope is negative?

e. Drag the line so that it is perfectly vertical . What happened to the
triangle? What is the slope now?

f. Can a vertical line be represented in slope-intercept form? Why or
why not? Give an example of an equation in slope-intercept form of
a line that would be almost vertical.

The y-intercept

In the slope-intercept form of the equation of a line, y = m x + b, b is the y-intercept
of the line.

1. Vary the value of the y -intercept of the line using the b slider.

a. In which direction does the line move when b increases? When b
decreases?

b. What is always true of the line when the y-intercept is positive?

c. What is always true of the line when the y-intercept is negative?

d. How does varying the y-intercept affect the slope of the line?

2. What is the y-intercept of the line y = x + 2.3? Write down your answer.
Then check it by graphing the line using the Gizmo.

3. Set b = −4. Now vary the slope of the line using the m slider. On the
graph, you can see many lines that have a y-intercept of −4.

a. Write down the slope-intercept equations of three different lines
with a y-intercept of −4. What do these equations have in common ?

i.
ii.
iii.

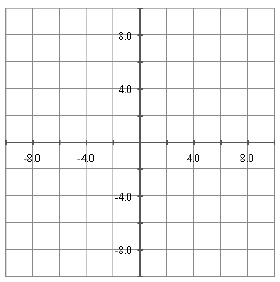
b. Write down the slope-intercept equations of three different lines
with a y-intercept of 1.5. Sketch the graphs of these lines using
different colors on the coordinate grid below. Use the Gizmo to
check your answers.

i.
ii.
iii.

Putting it all Together

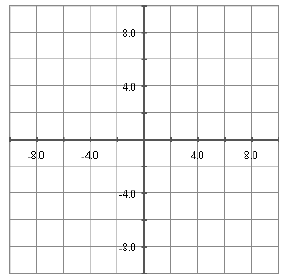
1. Sketch the graph of the line y = 2x − 3 on the coordinate grid below.
Check your answer by typing in the appropriate values for m and b in the
Gizmo. (Press Enter after typing in each value.)

2. Which of the following lines goes downwards from left to right? Circle your
answer .

a. y = 2x +5
b. y = 2x − 6
c. y = 2x
d. y = −2x + 20

Lines that go downwards from left to right have negative slopes. In the
slope-intercept form, y = m x + b, m represents the slope of the line.
Therefore, the line y = −2x + 20 has a slope of −2, and will go downwards
from left to right.

3. Which line is horizontal? Circle your answer.

a. y = 2
b. y = 2x
c. y = −2x
d. y = x

Horizontal lines have slopes of 0. In the slope-intercept form, y = m x + b,
m represents the slope of the line. y = 2 can also be written as the
equivalent equation y = 0x + 2. Therefore, y = 2 has a slope of 0.

4. Which line passes through the origin? Circle your answer.

a. y = 3
b. y = x – 3
c. y = −3x
d. y = 2x + 1

y = −3x. Any line in the form y = m x has a y-intercept of 0, and will
therefore pass through the origin.

5. What is the equation of a line with a y-intercept at (0, 6) and a slope of 3?
Circle your answer.

a. y = 3x + 6
b. y = 6x + 3
c. y = 3x − 6
d. y = −6x − 3

In the slope-intercept form, y = m x + b, m represents the slope of the line
and b represents the y-intercept. Substituting m = 3 and b = 6 into the
slope-intercept form results in y = 3x + 6.