College Algebra
Tutorial 2:
Integer Exponents
 Learning Objectives Learning Objectives
|
After completing this tutorial, you should be able to:- Use the definition of exponents.
- Simplify exponential expressions involving multiplying like bases, zeroas an exponent, dividing like bases, negative exponents, raising a baseto two exponents, raising a product to an exponent and raising a quotientto an exponent.
|
 Introduction Introduction
|
| This tutorial covers the basic definition and rules of exponents. The rules it covers are the product rule, quotient rule, power rule, productsto powers rule, quotients to powers rule as well as the definitions forzero and negative exponents. Exponents are everywhere in algebra and beyond. Let's see what we can do with exponents. |
 Tutorial Tutorial
|
| Definition of Exponents 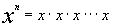
(note there are n x'sin the product)
x = base, n = exponent |
| Exponents are another way towrite multiplication. The exponent tells you how many times a base appears in a PRODUCT.
 Example1: Evaluate Example1: Evaluate 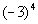 . .
|
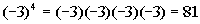 | *Write the base -3 in a product 4 times
*Multiply |
 Example2: Evaluate Example2: Evaluate 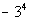 . . |
 | *Negate 3 to the fourth
*Put a - in front of 3 written in a product4 times
*Multiply |
| Hey, this looks a lot like example 1!!!! It may look alike, but they ARE NOT exactly the same. Can yousee the difference between the two?? Hopefully, you noticed thatin example 1, there was a ( ) around the - and the 3. In this problem,there is no -. This means the - is NOT part of the base, so it willnot get expanded like it did in example 1. It is interpreted as finding the negative or opposite of 3 to thefourth power. |
 Example3: Evaluate Example3: Evaluate 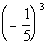 . . |
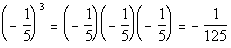 | *Write the base -1/5 in a product 3 times
*Multiply |
Multiplying Like Bases With Exponents
(The Product Rule for Exponents)Specific Illustration |
| Lets first start by using the definition of exponentsto help you to understand how we get to the law for multiplying like baseswith exponents: 
Note that 2 + 3 = 5, which is the exponent we ended up with. Wehad 2 xs written in a product plus another3 xs written in the product for a total of5 xs in the product. To indicate thatwe put the 5 in the exponent.
Let's put this idea together into a general rule: |
Multiplying Like Bases With Exponents
(Product Rule for Exponents)in general, 
|
| In other words, when you multiply like basesyou add your exponents. The reason is, exponents count how many of your base you have ina product. So if you are continuing that product, you are addingon to the exponents.
 Example4: Use the product rule to simplify the expression Example4: Use the product rule to simplify the expression  . .
|
 | *When mult. like bases you add your exponents |
 Example5: Use the product rule to simplify the expression Example5: Use the product rule to simplify the expression 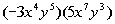 . . |
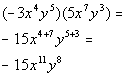 |
*When mult. like bases you add your exponents |
| Zero as an exponent 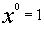
|
| Except for 0, any base raised to the 0 powersimplifies to be the number 1. Note that the exponent doesnt become 1, but the whole expression simplifiesto be the number 1.  Example6: Evaluate Example6: Evaluate 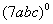 . .
|
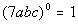 | *Any expression raised to the 0 power simplifiesto be 1 |
 Example7: Evaluate Example7: Evaluate 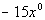 . . |
| Be careful on this example. Order of operations says to evaluateexponents before doing any multiplication. This means we need tofind x raised to the 0 power first and thenmultiply it by -15. |
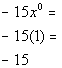 |
*x raised to the0 power is 1
*Multiply |
Dividing Like Bases With Exponents
(Quotient Rule for Exponents)Specific Illustration |
| Lets first start by using the definition of exponentsto help you to understand how we get to the law for dividing like baseswith exponents: 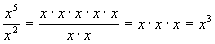
Note how 5 - 2 = 3, the final answers exponent. When you multiplyyou are adding on to your exponent, so it should stand to reason that whenyou divide like bases you are taking away from your exponent. Let's put this idea together into a general rule: |
Dividing Like Bases With Exponents
(Quotient Rule for Exponents)in general,
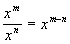
|
| In other words, when you divide like basesyou subtract their exponents. Keep in mind that you always take the numerators exponent minus yourdenominators exponent, NOT the other way around.
 Example8: Find the quotient Example8: Find the quotient 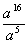 . .
|
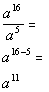 |
*When div. like bases you subtract your exponents |
 Example9: Find the quotient Example9: Find the quotient 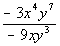 . . |
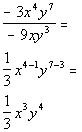 | *When div. like bases you subtract your exponents |
Negative Exponents
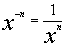 or or 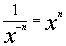 |
Be careful with negative exponents. Thetemptation is to negate the base, which would not be a correct thing todo. Since exponents are anotherway to write multiplication and the negative is in the exponent, to writeit as a positive exponent we do the multiplicative inverse which is totake the reciprocal of the base.
 Example10: Simplify Example10: Simplify 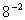 . .
|
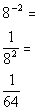 | *Rewrite with a pos. exp. by taking recip.of base
*Use def. of exponentsto evaluate |
 Example11: Simplify Example11: Simplify  . . |
 | *Rewrite with a pos. exp. by taking recip.of base *Use def. of exponentsto evaluate |
Base Raised to Two Exponents
(Power Rule for Exponents)
Specific Illustration |
| Lets first start by using the definition of exponentsas well as the law for multiplying like basesto help you to understand how we get to the law for raising a base to twoexponents: 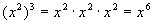
Note how 2 times 3 is 6, which is the exponent of the final answer. We can think of this as 3 groups of 2, which of course would come out tobe 6. |
Base Raised to two Exponents
(Power Rule for Exponents)
in general,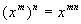
|
| In other words, when you raise a base to twoexponents, you multiply those exponents together. Again, you can think of it as n groups ofmif it helps you to remember.
 Example12: Simplify Example12: Simplify 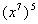 . .
|
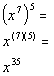 | *When raising a base to two powers you mult.your exponents |
 Example13: Simplify Example13: Simplify 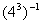 . . |
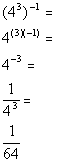 |
*When raising a base to two powers you mult.your exponents*Use the definitionof neg. exponents to rewrite as the recip. of base *Use the def. of exponentsto evaluate |
A Product Raised to an Exponent
(Products to Powers Rule for Exponents)
Specific Illustration |
| Lets first start by using the definition of exponentsto help you to understand how we get to the law for raising a product toan exponent: 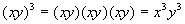
Note how both bases of your product ended up being raised by the exponentof 3. |
A Product Raised to an Exponent
(Products to Powers Rule for Exponents)
in general,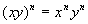
|
In other words, when you have a PRODUCT (nota sum or difference) raised to an exponent, you can simplify by raisingeach base in the product to that exponent.
 Example14: Simplify Example14: Simplify 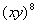 . .
|
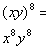 | *When raising a product to an exponent, raiseeach base of the product to that exponent |
 Example15: Simplify Example15: Simplify 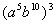 . . |
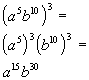 | *When raising a product to an exponent, raiseeach base of the product to that exponent *Mult. exponents when using powerrule for exponents |
A Quotient Raised to an Exponent
(Quotients to Powers Rule for Exponents)
Specific Illustration |
| Lets first start by using the definition of exponentsto help you to understand how we get to the law for raising a quotientto an exponent: 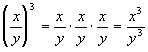
Since division is really multiplication of the reciprocal, it has thesame basic idea as when we raised a product to an exponent. |
A Quotient Raised to an Exponent
(Quotients to Powers Rule for Exponents)
in general,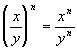
|
In other words, when you have a QUOTIENT (nota sum or difference) raised to an exponent, you can simplify by raisingeach base in the numerator and denominator of the quotient to that exponent.
 Example16: Simplify Example16: Simplify  . .
|
 |
*When raising a quotient to an exponent, raiseeach base of the quotient to that exponent *Use def. of exponentsto evaluate |
| Of course, we all know that life isnt so cut and dry. A lotof times you need to use more than one definition or law of exponents toget the job done. What we did above was toset the foundation to make sure you have a good understanding of the differentideas associated with exponents. Next we will work throughsome problems which will intermix these different laws. |
| Simplifying an Exponential Expression |
| When simplifying an exponential expression, write it so thateach base is written one time with one POSITIVE exponent. In other words, write it in the most condense form you can making surethat all your exponents are positive. A lot of times you have to use more than one rule to get the job done. As long as you use the rule appropriately you should be fine. |
 Example17: Simplify the exponential expression Example17: Simplify the exponential expression 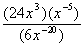 . . |
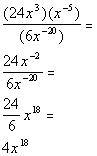 | *When mult. like basesyou add your exponents: 3 + (-5) = -2
*When div. like basesyou subtract your exponents: -2 - (-20) = 18
|
 Example18: Simplify the exponential expression Example18: Simplify the exponential expression 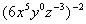 . . |
 | * |
 Example19: Simplify the exponential expression Example19: Simplify the exponential expression 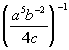 . . |
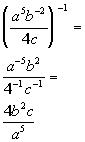 |
* |
| Be careful going into the last line. Since bdoesn'thave a negative exponent, we DO NOT take the reciprocal of b. The other bases each have a negative exponent, so those bases we have totake the reciprocal of. |
 Practice Problems Practice Problems
|
| These are practice problems to help bring you to the next level. It will allow you to check and see if you have an understanding of thesetypes of problems. Math works just like anythingelse, if you want to get good at it, then you need to practice it. Even the best athletes and musicians had help along the way and lots ofpractice, practice, practice, to get good at their sport or instrument. In fact there is no such thing as too much practice. To get the most out of these, you should work the problem out onyour own and then check your answer by clicking on the link for the answer/discussionfor that problem. At the link you will find the answeras well as any steps that went into finding that answer. |
 PracticeProblems 1a - 1f: PracticeProblems 1a - 1f:Simplify the exponential expression. |
1a. 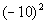
(answer/discussionto 1a) | 1b. 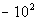
(answer/discussionto 1b) |
1c. 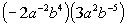
(answer/discussionto 1c) | 1d. 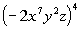
(answer/discussionto 1d) |
1e. 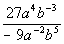
(answer/discussionto 1e) | 1f. 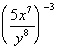
(answer/discussionto 1f) |
 Need Extra Help on These Topics? Need Extra Help on These Topics?
|
The following are webpages that can assistyou in the topics that were covered on this page:
int_alg_tut23_exppart1.htm
This website gives the definition of and some of the basic rules forexponents.
This webpage gives the definition of exponents.
int_alg_tut24_exppart2.htm
This website helps you with some of the basic rules for exponents.
This website gives an overview of various rules for exponents.
This webpage gives an overall review of exponents.
rulesofexponents.htm
This website helps you with some of the basic rules for exponents.
This webpage goes over the rules of exponents.
This website helps you with the product rule for exponents.
This website helps you with the quotient rule for exponents.
radexpex1.htm
This website helps you with the quotient rule for exponents.
This website helps you with the rule for raising a base to two exponents.
|
for somemore suggestions. |
All contents
June 22, 2003 |