Synthetic Division

Abstract
This handout is a supplement to Section 10.8 of the textbook used in my Math 100 class. The material
here is not discussed in the textbook.

What is synthetic division ?
In Section 10.8 of our textbook, we discussed long division of polynomials. For example, using long division,
we found that


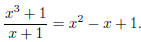
For certain types of polynomial division problems , there is an easy method known as synthetic division,
which yields the quotient very quickly. As we mentioned in lecture, synthetic division also has an extremely
useful application, which we examine here as well. Let us begin by finding a quotient of two polynomials
with long division.

Example 1. Let us perform the division indicated by 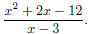Using long division as we did in lecture,1
we obtain the result in Figure 1.

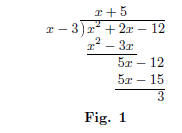
Hence,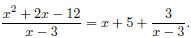

Using synthetic division, we use only the coefficients of the polynomial x² +2x−12 and the opposite of the
−3 in the polynomial x − 3.

Example 2. Use synthetic division to perform the polynomial division indicated by We write 3 (the
opposite of the −3 in x−3) and the coefficients of x² +2x−12 in a row, and then we box off the numbers as shown
in Figure 2.

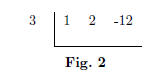
The first step is to bring the 1 straight down as shown in Figure 3.

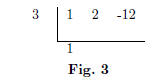
Next, multiply the 3 by the 1 on the bottom row, and write the result in the blank spot just below the 2.


Add the 2 and 3, and write the result in the same column.


Multiply the 3 and the 5, and write the result in the spot just below the −12.


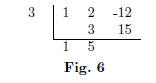
Finally, add the −12 and the 15, and write the result in the same column.

Notice that the last line “1 5 3” gives the coefficients and the remainder that we are seeking. That is, the last
line “1 5 3” means x + 5 with a remainder of 3. Thus,


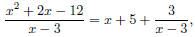
which is precisely the answer we obtained when we used long division of polynomials.
How do we know that the “1 5 3” does not mean x² + 5x + 3? The polynomial which we are dividing is of
degree 2, so the quotient is a polynomial of degree 1 less than that.

When does synthetic division work?

Synthetic division can only be used when one is dividing by a polynomial of degree 1 and which has a leading
coefficienct of 1. For example, synthetic division can be used to do the division problems
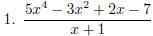and


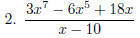
because we are dividing by x + 1 and x − 10, respectively. On the other hand, we cannot use synthetic
division in the division problems
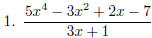  and


because the coefficient of x is 3 in the first problem and because we are dividing by a second-degree polynomial
in the second problem. To understand why this is the case, let us see why synthetic division works.

Why does synthetic division work?

Synthetic division is nothing magical. It is long division. It works quite simply because we are dividing by
a polynomial of degree 1 with leading coefficient 1. Synthetic division really is the act of writing down only
the essential parts of long division. Let us put Example 1 and Example 2 side by side to see this.

Example 3. Let us perform the division indicated by 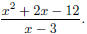We show the long division steps on the left
and the accompanying synthetic division step on the right in each figure. First, we write the problem so that we can
start the division processes.

Step 1 Setting up the division.

Step 2 Bringing the 1 straight down is really writing the x in the quotient. The 1 is the coefficient of x; i.e., the 1
is really shorthand for 1x.


Step 3 Multiplying the 3 by the 1 is the same step as multiplying the x by the x − 3, except that we are really only
writing down the term −3x and not writing down the x² term since it is going to cancel in this step.


Step 4 Since the leading coefficient of x − 3 is 1, we know we are going to have to multiply x − 3 by 5 to obtain the
5x in the 5x − 12. Thus, when we add the 2 and the 3 in synthetic division, we are simultaneously doing two steps
in the long division process: subtracting the x ² − 3x from the x² + 2x (We don’t write the x² terms in the synthetic
division steps since they cancel anyway.) and writing the +5 in the quotient.



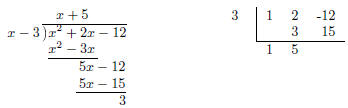
Step 5 Multiplying the 3 by the 5 in synthetic division is the same as multiplying the 5 by the x − 3 in long
division and writing the 5x − 15.


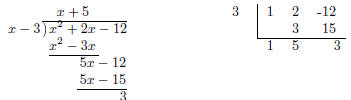
Step 6 Adding the −12 and the 15 in synthetic division is the same as subtracting the 5x − 15 from the 5x − 12
(i.e., 5x − 12 − (5x − 15) = −12 + 15) in long division. In both processes we obtain the remainder of 3.

Exercise. Do the division indicated by 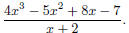Use both long division and synthetic division, and write
the matching steps for each side-by-side as in Example 3.