Rational Expressions

Operations with Rational Expressions
The operations of addition, subtraction, multiplication, and division with rational expressions follow the same
rules that are used with common fractions. The key, as with simplifying rational expressions, is to factor the
polynomials .

I. Multiplication of Rational Expressions

Recall with fractions Also recall when mulitplying fractions that you may
cross-cancel; that is, reduce common factors of any numerator with any denominator.
Example 1:

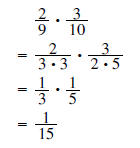
The same is true of multiplying rational expressions. As before, the key is to factor the polynomials first.

Example 2: Simplify

Solution : First factor the polynomials. Don’t forget to cancel common factors!

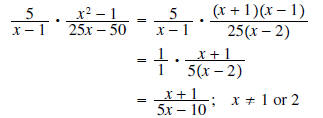
Before looking at the next example, recall our strategies for factoring:
1. Factor out any common factors
2. If more than 3 terms, try factoring by grouping
3. Recognize special products
4. Factor trinomials using product /sum strategies:
a) 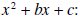factors into (x+m)(x+n) where
mn=c and m+n=b
b) 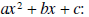split bx into mx+nx, where
mn=ac and m+n=b, then factor by grouping.

Example 3: Simplify

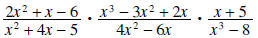
Solution:

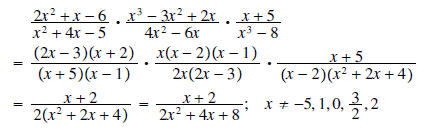
Example 4: Simplify

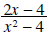
Solution:

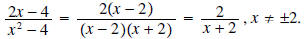
II. Division of Rational Expressions

Recall with fractions 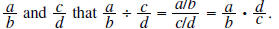In other words, dividing by a fraction is the
same as multiplying by its reciprocal. The same is true for rational expressions. As before, the key to making
the work easier is to factor the polynomials first.

Example 5:

Solution: Begin by writing the second term as

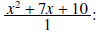
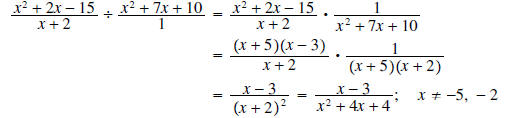
Notice that the x+2 terms cannot be cancelled since both are in the denominator.

Multiplication and Division can also appear together. Only take the reciprocal of the fractions you are
dividing.

Example 6:

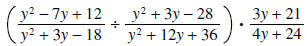
Solution: We only take the reciprocal of the second fraction:

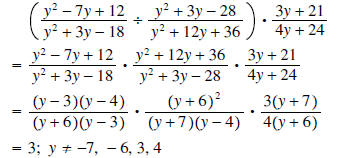
Question: Explain why the values -7, -6, 3, and 4 are excluded in the previous example.
Answer: The easiest way to understand why these values have been excluded is to write

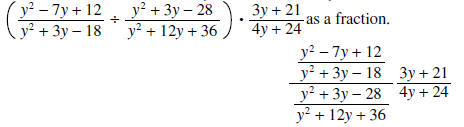
y≠-6 follows since 4y+24 cannot equal 0.
We also get y≠3 because the term

The next observation is that the denominator of

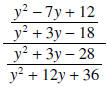
that is,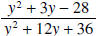cannot equal zero. Factoring the numerator and denominator of this rational expression
we have

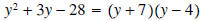
The numerator is zero if y =-7 or if y=4. This accounts for excluding the numbers -7 and 4. The
denominator of

factors into which means we have to exclude -6, but we have already done that.

Question: What values of x are not allowed in the rational expression

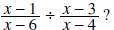
Answer: 3, 4, 6 (The value 3 is not allowed because is zero at x=3 and we cannot divide by 0.
x=4 is not allowed because we have the term x-4 in the denominator. Similarly x=6 is not allowed
because the term x-6 is the denominator of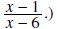

III. Addition and Subtraction of Rational Expressions

Recall that addition and subtraction is done by first finding a common denominator. The least common
denominator (LCD) of several fractions is the product of all prime factors of the denominators. A factor only
occurs more than once in the LCD if it occurs more than once in any one fraction. Once you have the LCD,
convert each fraction to an equivalent fraction with the LCD, then add or subtract the numerators.

Example 7:

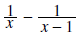
Solution: The LCD here is 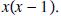We convert each fraction to an equivalent one with this
denominator: That is,

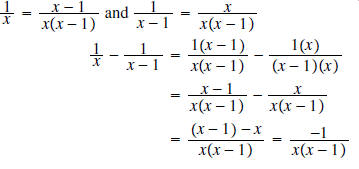
Example 8:

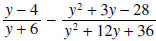
Solution: We must factor the denominators first to find the LCD:

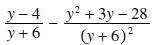
The LCD is 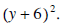We now convert each fraction to an equivalent fraction using this denominator:

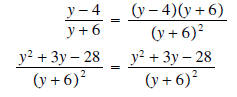
Next subtract the two expressions

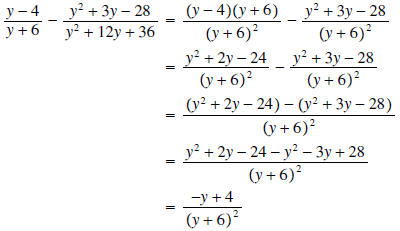
Note that the minus sign in the numerator was distributed across the expression

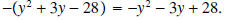
Question: What is the common denominator in

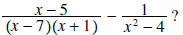
Answer: