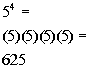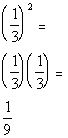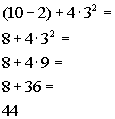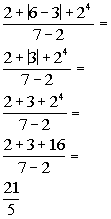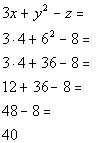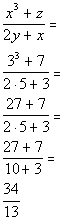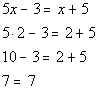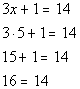Beginning Algebra
Tutorial 4:
Introduction to Variable Expressions
and Equations
 Learning Objectives
Learning Objectives
|
After completing this tutorial, you should be able to:
-
Evaluate an exponential expression.
-
Simplify an expression using the order of operations.
-
Evaluate an expression.
-
Know when a number is solution to an equation or not.
-
Translate an english expression into a math expression.
-
Translate an english statement in to a math equation.
|
 Introduction
Introduction
|
| This tutorial will go over some key definitions and phrases used when
specifically working with algebraic expressions as well as evaluating them.
We will also touch on the order of operations. It is very IMPORTANT
that you understand some of the math lingo that is used in an algebra class,
otherwise it may all seem Greek to you. Knowing
the terms and concepts on this page will definitely help you build an understanding
of what a variable is and get you more comfortable working with them,
I know you will do a lot better than Baloo in this cartoon link.
Variables are a HUGE part of algebra, so it is very important for you to
feel at ease around them in order to be successful in algebra. So
let's get going and help you get on the road to being variable savvy. |
 Tutorial
Tutorial
|
| An exponent tells you how many times that you write a base in a PRODUCT.
In other words, exponents are another way to write MULTIPLICATION.
Lets illustrate this concept by rewriting the product (4)(4)(4)
using exponential notation:
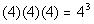
In this example, 4 represents the base and 3 is the exponent.
Since 4 was written three times in a product, then our exponent is 3.
We always write our exponent as a smaller script found at the top right
corner of the base.
You can apply this idea in the other direction. Lets say you
have it written in exponential notation and you need to evaluate it. The
exponent will tell you how many times you write the base out in a product.
For example if you had 7 as your base and 2 as your exponent and you wanted
to evaluate out you could write it out like this:

|
 Example
1: Evaluate Example
1: Evaluate 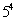 |
| In this problem, what is the base?
If you said 5, you are correct!
What is the exponent?
If you said 4, you are right!
Lets rewrite it as multiplication and see what we get for an answer: |
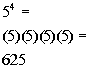 |
*Rewrite the base 5, four times in a product
*Multiply |
 Example
2: Evaluate Example
2: Evaluate 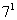 |
| In this problem, what is the base?
If you said 7, you are correct!
What is the exponent?
If you said 1, you are right!
Lets rewrite it as multiplication and see what we get for an answer: |
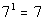 |
*Rewrite the base 7, one time in a product |
 Example
3: Evaluate Example
3: Evaluate 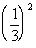 |
| In this problem, what is the base?
If you said 1/3, you are correct!
What is the exponent?
If you said 2, you are right!
Lets rewrite it as multiplication and see what we get for an answer: |
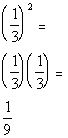 |
*Rewrite the base 1/3, two times in a product
*Multiply
|
| Note that when you have a 2 as an exponent, which is also known
as squaring the base. In this problem we could say that we are looking
for 1/3 squared. |
|
Order of Operations
Please
Parenthesis or grouping symbols
Excuse
Exponents (and radicals)
My Dear Multiplication/Division
left to right
Aunt Sally Addition/Subtraction
left to right |
| When you do have more than one mathematical operation, you need to
use the order of operations as listed above. You may have already heard
of the saying "Please Excuse My Dear Aunt Sally". It is just a way
to help you remember the order you need to go in when applying the order
of operations. |
 Example
4: Simplify Example
4: Simplify 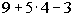 . . |
 |
*Multiply
*Add
*Subtract |
 Example
5: Simplify Example
5: Simplify 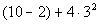 |
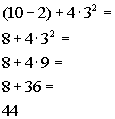 |
*Inside ( )
*Exponent
*Multiply
*Add |
 Example
6: Simplify Example
6: Simplify 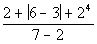 . . |
| Note that the absolute value symbol | | is a fancy grouping
symbol. In terms of the order of operations, it would be including
on the first line with parenthesis.
So in this problem, the first thing we need to do is work the inside
of the absolute value. And then go from there. |
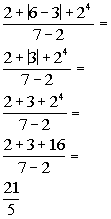 |
*Inside | |
*Exponent
*Add in num. and subtract in den.
|
| A variable is a letter that represents a number.
Don't let the fact that it is a letter throw you. Since it represents
a number, you treat it just like you do a number when you do various mathematical
operations involving variables.
x is a very common variable that is used
in algebra, but you can use any letter (a,
b,
c,
d,
....) to be a variable. |
| An algebraic expression is a number, variable or combination
of the two connected by some mathematical operation like addition, subtraction,
multiplication, division, exponents, and/or roots.
2x + y, a/5,
and 10 - r are all examples of algebraic expressions. |
| You evaluate an expression by replacing the variable with the
given number and performing the indicated operation. |
| When you are asked to find the value of an expression, that
means you are looking for the result that you get when you evaluate the
expression. |
| So keep in mind that vary means to change - a variable allows an
expression to take on different values, depending on the situation.
For example, the area of a rectangle is length times width. Well,
not every rectangle is going to have the same length and width, so we can
use an algebraic expression with variables to represent the area and then
plug in the appropriate numbers to evaluate it. So if we let the
length be the variable l and width be w,
we can use the expression lw. If a given
rectangle has a length of 4 and width of 3, we would evaluate the expression
by replacing l with 4 and w
with 3 and multiplying to get a value of 4 times 3 or 12.
Lets step through some examples that help illustrate these ideas. |
 Example
7: Evaluate the expression Example
7: Evaluate the expression 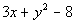 when x = 4, y =
6, z = 8.
when x = 4, y =
6, z = 8. |
| Plugging in the corresponding value for each variable and then evaluating
the expression we get: |
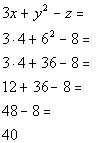 |
*Plug in 4 for
x, 6 for y, and 8 for z
*Exponent
*Multiply
*Add
*Subtract |
 Example
8: Evaluate the expression Example
8: Evaluate the expression 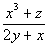 when x = 3, y =
5, and z = 7.
when x = 3, y =
5, and z = 7. |
| Plugging in the corresponding value for each variable and then evaluating
the expression we get: |
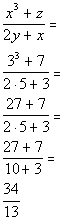 |
*Plug in 3 for x,
5 for y, and 7 for z
*Exponent
*Multiply
*Add
|
|
Equation
Two expressions set equal to each other.
|
|
Solution
A value, such that, when you replace the variable with it, it makes
the equation true.
(the left side comes out equal to the right side)
|
|
Solution Set
Set of all solutions.
|
 Example
9: Is 2 a solution of Example
9: Is 2 a solution of  ? ? |
| Replacing x with
2 we get: |
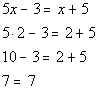 |
*Plug in 2 for x
*Evaluate both sides |
| Is 2 a solution?
Since we got a TRUE statement (7 does in fact equal 7), then
2
is a solution to this equation. |
 Example
10: Is 5 a solution of Example
10: Is 5 a solution of 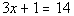 ? ? |
| Replacing x with
5 we get: |
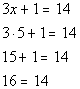 |
*Plug in 5 for x
*Evaluate both sides |
| Is 5 a solution?
Since we got a FALSE statement (16 does not equal 14), then 5
is not a solution. |
Translating an
English Phrase Into an
Algebraic Expression
|
| Sometimes, you find yourself having to write out your own algebraic
expression based on the wording of a problem.
In that situation, you want to
-
read the problem carefully,
-
pick out key words and phrases and determine their equivalent mathematical
meaning,
-
replace any unknowns with a variable, and
-
put it all together in an algebraic expression.
The following are some key words and phrases and
their translations: |
| Addition: sum, plus, add to, more than,
increased by, total |
| Subtraction: difference of, minus,
subtracted from, less than, decreased by, less |
| Multiplication: product, times, multiply,
twice, of |
| Division: quotient divide, into,
ratio |
 Example
11: Write the phrase as an algebraic expression. Example
11: Write the phrase as an algebraic expression.
The sum of a number and 10. |
| In this example, we are not evaluating an expression, so we will not
be coming up with a value. However, we are wanting to rewrite it
as an algebraic expression.
It looks like the only reference to a mathematical operation is the
word sum. So, what operation will we have in this expression?
If you said addition, you are correct!!!
The phrase 'a number' indicates that it is an unknown number.
There was no specific value given to it. So we will replace
the phrase 'a number' with the variable x.
We want to let our variable represent any number that is unknown
Putting everything together, we can translate the given english phrase
with the following algebraic expression: |
| The sum of a number and 10
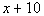
|
*'sum' = +
*'a number' = variable x |
 Example
12: Write the phrase as an algebraic expression. Example
12: Write the phrase as an algebraic expression.
The product of 5 and a number. |
| Again, we are wanting to rewrite this as an algebraic expression, not
evaluate it.
This time, the phrase that correlates with our operation is 'product'
- so what operation will we be doing this time? If you said
multiplication,
you are right on.
Again, we have the phrase 'a number', which again is going to be replaced
with a variable, since we do not know what the number is.
Lets see what we get for this answer: |
| The product of 5 and a number
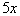
|
*'product' = multiplication
*'a number' = variable x |
|
Translating a Sentence into an Equation
|
| Since an equation is two expressions set equal to each other, we will
be using the same mathematical translations we did above. The difference
is we will have an equal sign between the two expressions.
The following are some key words and phrases
that translate into an equal sign (=): |
| Equal Sign (=) : equals, gives, is,
yields, amounts to, is the same as |
 Example
13: Write the sentence as an equation.
Let x represent the unknown number. Example
13: Write the sentence as an equation.
Let x represent the unknown number.
The quotient of 3 and a number is ½. |
| Do you remember what quotient translates into? If
you said division, you are doing great.
'Is' will be replaced by the symbol =.
Lets put together everything going left to right: |
|
The quotient of 3 and a number is ½

|
 Example
14: Write the sentence as an equation.
Let x represent the unknown number. Example
14: Write the sentence as an equation.
Let x represent the unknown number.
7 less than 3 times a number is the same as 0. |
| Do you remember what less than translates into?
If you said subtraction, you are doing great.
Do you remember what times translates into? If you
said multiplication, you are correct.
'Is the same as' will be replaced by the symbol =.
Lets put together everything going left to right: |
|
7 less than 3 times a number is the same as 0.
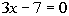
|
 Practice Problems
Practice Problems
|
| These are practice problems to help bring you to the next level.
It will allow you to check and see if you have an understanding of these
types of problems. Math works just like anything
else, if you want to get good at it, then you need to practice it.
Even the best athletes and musicians had help along the way and lots of
practice, practice, practice, to get good at their sport or instrument.
In fact there is no such thing as too much practice.
To get the most out of these, you should work the problem out on
your own and then check your answer by clicking on the link for the answer/discussion
for that problem. At the link you will find the answer
as well as any steps that went into finding that answer. |
 Practice
Problems 1a - 1b: Practice
Problems 1a - 1b:
Evaluate.
|
1a. 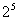
(answer/discussion
to 1a) |
1b. 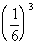
(answer/discussion
to 1b) |
 Practice
Problems 2a - 2b: Practice
Problems 2a - 2b:
Simplify each expression.
|
2a. 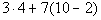
(answer/discussion
to 2a) |
2b. 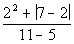
(answer/discussion
to 2b) |
 Practice
Problem 3a: Practice
Problem 3a:
Evaluate the expression if x = 1, y =
2, and z = 3.
|
3a. 
(answer/discussion
to 3a) |
 Practice
Problems 4a - 4b: Practice
Problems 4a - 4b:
Decide whether the given number is a solution of the
given equation.
|
4a. Is 0 a solution to 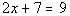 ? ?
(answer/discussion
to 4a) |
4b. Is 8 a solution to  ?
?
(answer/discussion
to 4b) |
 Practice
Problems 5a - 5b: Practice
Problems 5a - 5b:
Write each phrase as an algebraic expression.
Let x represent the unknown number.
|
5a. 9 less than 5 times a number.
(answer/discussion
to 5a) |
5b. The product of 12 and a number.
(answer/discussion
to 5b) |
 Practice
Problems 6a - 6b: Practice
Problems 6a - 6b:
Write each sentence as an equation. Let x
represent
the unknown number.
|
6a. The sum of 10 and 4 times a number is the same as 18.
(answer/discussion
to 6a) |
6b. The quotient of a number and 9 is 1/3.
(answer/discussion
to 6b) |
 Need Extra Help on These Topics?
Need Extra Help on These Topics?
|
The following are web pages that can assist
you in the topics that were covered on this page:
This webpage goes over the order of operations.
This webpage helps with translating english into math. |
for some
more suggestions. |
All contents
Jan. 8, 2002 |
 Example
1: Evaluate
Example
1: Evaluate