Thank you for visiting our site! You landed on this page because you entered a search term similar to this: slope formula, Quadratic formula, slope intercept formula sheet. We have an extensive database of resources on slope formula, Quadratic formula, slope intercept formula sheet. Below is one of them. If you need further help, please take a look at our software "Algebrator", a software program that can solve any algebra problem you enter!
|
Average Monthly Expenses |
||||||
|
1990 |
1991 |
1992 |
1993 |
1994 |
1995 |
|
|
Rent/House Payments |
550 |
570 |
580 |
620 |
630 |
670 |
|
Utilities |
65 |
72 |
83 |
75 |
72 |
75 |
|
Food |
160 |
180 |
185 |
195 |
220 |
225 |
|
Transportation |
230 |
227 |
223 |
215 |
215 |
205 |
|
Entertainment |
35 |
35 |
40 |
42 |
42 |
45 |
2. Find the slope of a line fitted to each type of expense, to see how that expense changed during the time period 1990 through 1995. To find the slope for Rent/House Payments, position the cursor in, say, cell H4 and type =SLOPE(B4:G4,B3:G3). The slope of the best-fitting line will be displayed. Cursor to cell H5 and similarly type =SLOPE(B5:G5,B3:G3). Notice that the first cell range denotes the expenses and the second cell range denotes the year. Continue to find these slopes for all the five types of expenses.
If you have had some experience with cutting and pasting Excel formulas, you could type =SLOPE(B4:G4,B$3:G$3) in cell H4 and then copy this formula to cells H5 through H8.
|
Average Monthly Expenses |
|||||||
|
slope |
|||||||
|
1990 |
1991 |
1992 |
1993 |
1994 |
1995 |
||
|
Rent/House Payments |
550 |
570 |
580 |
620 |
630 |
670 |
23.42857 |
|
Utilities |
65 |
72 |
83 |
75 |
72 |
75 |
1.2 |
|
Food |
160 |
180 |
185 |
195 |
220 |
225 |
13 |
|
Transportation |
230 |
227 |
223 |
215 |
215 |
205 |
-4.82857 |
|
Entertainment |
35 |
35 |
40 |
42 |
42 |
45 |
2.085714 |
The slope indicates approximately how much the expenses are increasing or decreasing per year. For example Rent/House Payments increased approximately $23.43 per year. Transportation decreased approximately $4.83 per year.
3. Now make a graph showing how all these expenses changed between 1990 and 1995.
Highlight cells B3 through G8 and click on the graph icon. Choose XY(Scatter) and then click on the fourth subtype picture "Scatter with data points connected by lines." Then click Next.
Click on the Series Tab and type in the names of the five types of expenses. Click Next.
Click on the Titles tab and type "Monthly Expenses" for the Chart Title. Type "Year" for the Value(X)Axis and "Dollars" for the Value(Y)Axis. Click Next.
Click on As Object in and then click Finish. You can move or resize your graph as you wish. By clicking or double-clicking on any part of your graph, you can change the size, font, placement, etc. of that part. Your final graph should look similar to the following graph.
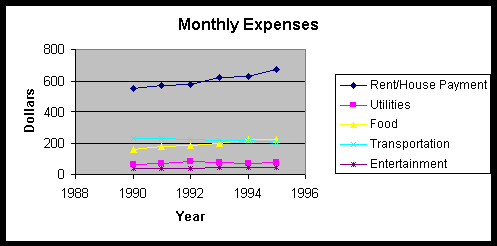
4. You can choose to graph only some of these expenses. For example, suppose you want the graph to show only Utility and Entertainment expenses. Highlight cells B3 through G3. Then hold down the Ctrl key and highlight cells B5 through G5. Next hold down the Ctrl key again and highlight cells B8 through G8. (All three of these rows should now be highlighted.) Next click on the graph icon and proceed as before. Your graph should look something like this:
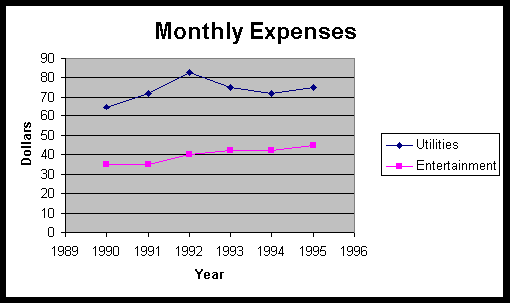
--------------------------------------------------------------------------------------------------------------------
|
Nuclear Energy Activity Worksheet |
Name ____________________ |
1. (3 points) Access the site and search for Nuclear Capacities. Find the table giving Historical and Projected Operable Nuclear Capacities by Region.
2. (3 points) Name three countries whose projected nuclear capacities are increasing over the next twenty years.
3. (3 points) Name three countries whose projected nuclear capacities are decreasing over the next twenty years.
4. (3 points) You should now copy Table 1 to your spreadsheet. Click and highlight the whole table, then click on Edit and then on Copy. Next open your spreadsheet. Click on cell A1, then click on Edit and then on Paste. All the data from Table 1 should now be transferred to the spreadsheet into columns A through G.
5. (12 points) Calculate the slope of the best-fit line for the data of each of your six countries. You might record this data in Column I. In Excel, for example, if the Country A data were in row 12, and since the years are in row 3, you would position the cursor at cell I1 and then type the command
= SLOPE(B12:G12,B3:G3)
|
COUNTRY |
SLOPE |
|
__________________________________ |
______________ |
|
__________________________________ |
______________ |
|
__________________________________ |
______________ |
|
__________________________________ |
______________ |
|
__________________________________ |
______________ |
|
__________________________________ |
______________ |
6. (15 points) Make a scatterplot with the data connected by line segments for these six countries. Print out a copy of your spreadsheet.
7. (5 points) Which of these six countries will be increasing their nuclear power the fastest? What is the "rate of change" of nuclear power in this country? Interpret this value.
8. (5 points) Which of these six countries will be decreasing their nuclear power the fastest? What is the "rate of change" of nuclear power in this country? Interpret this value.
9. (5 points) Which of these six countries has the smallest "rate of change" of their nuclear capacity? Explain why. What is this rate of change?
10. (5 points) In the year 2020, which of these six countries will be producing the most nuclear power? Which will be producing the least?
11. (5 points) Look at your original table with all the data for all the countries. In the year 2020, which country will be producing the most nuclear power? Which will be producing the least?
12. (5 points) Is there any country for which the rate of change of its nuclear power production is zero? Describe this countrys production.
--------------------------------------------------------------------------------------------------------------------
Nuclear Energy Activity Worksheet (Sample Answers)
1. (3 points) Access the site and search for Nuclear Capacities. Find the table giving Historical and Projected Operable Nuclear Capacities by Region.
2. (3 points) Name three countries whose projected nuclear capacities are increasing over the next twenty years.
India, China, Japan
3. (3 points) Name three countries whose projected nuclear capacities are decreasing over the next twenty years.
United States, Bulgaria, Germany
4. (3 points) You should now copy Table 1 to your spreadsheet. Click and highlight the whole table, then click on Edit and then on Copy. Next open your spreadsheet. Click on cell A1, then click on Edit and then on Paste. All the data from Table 1 should now be transferred to the spreadsheet into Columns A through G.
Data for the above countries:
|
1996 |
2000 |
2005 |
2010 |
2015 |
2020 |
|
|
US |
100817 |
95605 |
86800 |
80357 |
63881 |
49217 |
|
Bulgaria |
3538 |
3538 |
2722 |
2722 |
1906 |
1906 |
|
Germany |
22282 |
21063 |
20083 |
16120 |
11800 |
5250 |
|
India |
1695 |
2503 |
3103 |
5913 |
7640 |
9890 |
|
China |
2167 |
2167 |
6737 |
11542 |
14700 |
18760 |
|
Japan |
42369 |
43525 |
44321 |
47526 |
53623 |
54107 |
5. (6 points) Calculate the slope of the best-fit line for the data of each of your six countries. You might record this data in Column I. In Excel, for example, if the Country A data were in row 12, , and since the years are in row 3, you would position the cursor at cell I1 and then type the command
= SLOPE(B12:G12,B3:G3)
|
COUNTRY United States Bulgaria Germany India China Japan |
SLOPE -2123.39 -76.99 -692.17 349.74 740.91 544.99 |