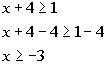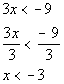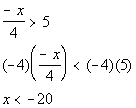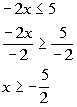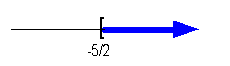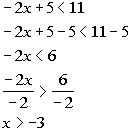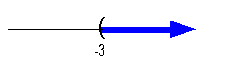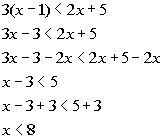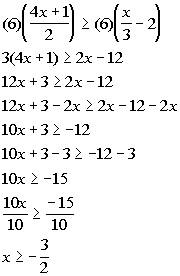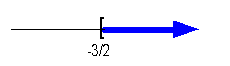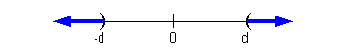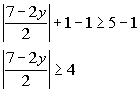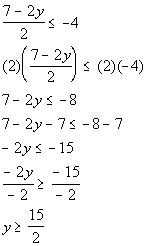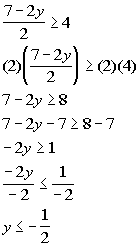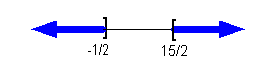College Algebra
Tutorial 22:
Linear Inequalities
 Learning Objectives Learning Objectives |
After completing this tutorial, you should be able to: - Use the addition, subtraction, multiplication, and division propertiesof inequalities to solve linear inequalities.
- Solve linear inequalities involving absolute values.
- Write the answer to an inequality using interval notation.
- Draw a graph to give a visual answer to an inequality problem.
|
 Introduction Introduction |
| In this tutorial we will be looking at solving linearinequalities. When solving linear inequalities, we use a lot of the same conceptsthatwe use when solving linear equations (shown in Tutorial14: Linear Equations in One Variable). Basically, westillwant to get the variable on one side and everything else on the othersideby using inverse operations. The difference is, when a variableisset equal to one number, that number is the only solution. But,whena variable is less than or greater than a number, there are an infinitenumber of values that would be a part of the answer. We will alsorevisit the definition of absolute value and how it applies toinequalities. If you need a review on absolute values . You never know whenyouwill need to know about inequalities, so you better get started. |
 Tutorial Tutorial |
| Read left to right: a < b ais less than b
a < b ais less than or equal to b a > b ais greater than b
a > b a isgreater than or equal to b |
| Interval notation is a way to notate the range ofvalues that wouldmake an inequality true. There are two types of intervals, openandclosed (described below), each with a specific way to notate it so wecantell the difference between the two. Note that in the interval notations (found below), youwill see thesymbol 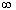 ,whichmeans infinity. ,whichmeans infinity.
Positive infinity (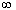 )means it goes on and on indefinitely to the right of the number - thereis no endpoint on the right. )means it goes on and on indefinitely to the right of the number - thereis no endpoint on the right. Negative infinity (-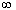 )means it goes on and on indefinitely to the left of the number - thereis no endpoint to the left. )means it goes on and on indefinitely to the left of the number - thereis no endpoint to the left. Since we don’t know what the largest or smallestnumbers are, we needto use infinity or negative infinity to indicate there is no endpointinone direction or the other. | In general, when using interval notation, you always putthe smallervalue of the interval first (on the left side), put a comma between thetwo ends, then put the larger value of the interval (on the rightside). You will either use a curved end ( or ) or a boxed end [ or ],dependingon the type of interval (described below). If you have either infinity or negative infinity oneither end, youalways use a curve for that end. This will indicate that there is nodefiniteendpoint in that direction, it keeps going and going. |
| An open interval does not include where yourvariable is equal tothe endpoint. To indicate this, we use a curved end as shownbelow. |
Inequality
x > a
x < a | Interval Notation for Open Intervals
(a, 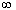 ) )
(-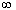 , a) , a) |
| When you graph an open ended end point, you use thesame curved end( or ) on the graph as you do in the intervalnotation. Also, darken in the part of the graph that is the solution. Forexample, |
| Inequality | Interval Notation for
Open Intervals | Graph | | x > 4 | (4, 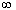 ) ) | 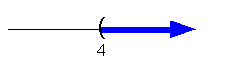 | | x < 4 | (-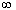 , 4) , 4) | 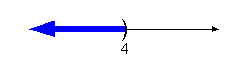 |
| A closed interval includes where your variable isequal to the endpoint. To indicate this, we use a boxed end as shownbelow. As mentioned above, even though a isincludedand has a boxed end, if it goes to either infinity or negative infinityon the other end, we will notate it with a curved end for that end only! |
Inequality
x > a
x < a | Interval Notation for Closed Intervals
[a, 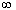 ) )
(-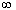 , a] , a] |
| When you graph a closed ended end point, you use thesame boxed end[ or ] on the graph as you do in the intervalnotation. Also, darken in the part of the graph that is the solution. Forexample, |
| Inequality | Interval Notation for
Closed Intervals | Graph | | x > 4 | [4, 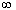 ) ) |  | | x < 4 | (-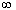 , 4] , 4] | 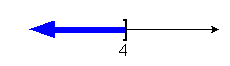 |
| Combining Open and ClosedIntervals |
| Sometimes one end of your interval is open and theother end is closed. You still follow the basic ideas described above. The closed endwill have a [ or ] on it’s end and the open end will have a( or ) on its end. |
Inequality
a < x < b
a < x < b | Interval Notation for Combining Open andClosed Intervals
(a, b]
[a, b) |
| Inequality | Interval Notation for
Combining Open and
Closed Intervals | Graph | | 3 < x < 6 | [3, 6) |  |
| Addition/Subtraction Propertyfor Inequalities If a < b, then a + c < b + c If a < b, then a - c < b - c |
In other words, adding orsubtracting thesame expression to both sides of an inequality does not change theinequality.
 Example1: Solve, write your answer in interval notation andgraphthe solution set: Example1: Solve, write your answer in interval notation andgraphthe solution set: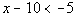 . .
|
 Interval notation: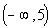 Graph:
 | *Inv. of sub. 10 is add. 10
*Open interval indicating allvalues less than5 *Visual showing all numbersless than 5 onthe number line |
| Note that the inequality stayed the same throughout theproblem. Adding or subtracting the same value to both sides does not change theinequality. The answer 'x is less than5' means thatif we put any number less than 5 back in the original problem, it wouldbe a solution (the left side would be less than the right side). As mentioned above, this means that we have more than just one numberforour solution, there are an infinite number of values that would satisfythis inequality. Interval notation:
We have an open interval since we are not including where it is equalto 5. x is less than 5, so5 is the largest value of the interval, so it goes on the right. Since there is no lower endpoint (it is ALL values less than 5), we putthe negative infinity symbol on the left side. The curved end on5 indicates an open interval. Negative infinity always has acurvedend because there is not an endpoint on that side. Graph:
We use the same type of notation on the endpoint as we did in theintervalnotation, a curved end. Since we needed to indicate allvaluesless than 5, the part of the number line that was to the left of 5 wasdarkened. |
 Example2: Solve, write your answer in interval notation and graphthesolution set: Example2: Solve, write your answer in interval notation and graphthesolution set: 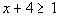 . . |
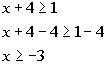 Interval notation: [-3, 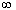 ) ) Graph:
 |
*Inv. of add 4 is sub. 4
*Closed interval indicating allvalues greaterthan or = -3 *Visual showing all numbersgreater than or= to -3 on the number line. |
| Note that the inequality stayed the same throughout theproblem. Adding or subtracting the same value to both sides does not change theinequality. The answer 'x is greaterthan or equal to-3' means that if we put any number greater than or equal to -3 back inthe original problem, it would be a solution (the left side would begreaterthan or equal to the right side). As mentioned above, this meansthat we have more than just one number for our solution, there are aninfinitenumber of values that would satisfy this inequality. Interval notation:
We have a closed interval since we are including where it is equalto -3. x is greater than orequalto -3, so -3 is our smallest value of the interval, so it goes on theleft. Since there is no upper endpoint (it is ALL values greater than orequalto -3), we put the infinity symbol on the right side. The boxedendon -3 indicates a closed interval. Infinity always has a curvedendbecause there is not an endpoint on that side. Graph:
We use the same type of notation on the endpoint as we did in theintervalnotation, a boxed end. Since we needed to indicateallvalues greater than or equal to -3, the part of the number line thatwasto the right of -3 was darkened. |
Multiplication/DivisionProperties for Inequalities
when multiplying/dividing by a positive value If a < b AND cis positive, then ac < bc If a < b AND cis positive, then a/c < b/c |
In other words, multiplyingor dividing thesame POSITIVE number to both sides of an inequality does not change theinequality.
 Example3: Solve, write your answer in interval notation andgraphthe solution set: Example3: Solve, write your answer in interval notation andgraphthe solution set: 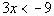 . .
|
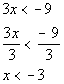 Interval notation: (-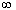 ,-3) ,-3) Graph:
 |
*Inv. of mult. by 3 is div. by 3
*Open interval indicating allvalues less than-3 *Visual showing all numbersless than -3 onthe number line
|
| Note that the inequality sign stayed the samedirection. Eventhough the right side was a -9, the number we were dividing both sidesby, was a positive 3. Multiplying or dividing both sides bythesame positive value does not change the inequality. Interval notation:
We have an open interval since there we are not including where itis equal to -3. x is lessthan -3, so -3 is our largest value of the interval, so it goes on theright. Since there is no lower endpoint (it is ALL values less than -3), weputthe negative infinity symbol on the left side. The curved end on-3 indicates an open interval. Negative infinity always has acurvedend because there is not an endpoint on that side. Graph:
We use the same type of notation on the endpoint as we did in theintervalnotation, a curved end. Since we needed to indicate allvaluesless than -3, the part of the number line that was to the left of -3wasdarkened. |
Multiplication/DivisionProperties for Inequalities
when multiplying/dividing by a negative value If a < b AND cis negative, then ac > bc If a < b AND cis negative, then a/c > b/c |
| In other words, multiplyingor dividing thesame NEGATIVE number to both sides of an inequality reverses the signofthe inequality. The reason for this is, when you multiply or divide anexpression bya negative number, it changes the sign of that expression. On thenumber line, the positive values go in a reverse or opposite directionthan the negative numbers go, so when we take the opposite of anexpression, we need to reverse our inequality to indicate this.
 Example4: Solve, write your answer in interval notation andgraphthe solution set: Example4: Solve, write your answer in interval notation andgraphthe solution set: 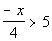 . .
|
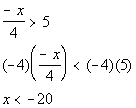 Interval notation: 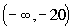 Graph:
 | *Inv. of div. by -4 is mult.by -4,
so reverse inequality sign
*Open interval indicating allvalues less than-20 *Visual showing all numbersless than -20 onthe number line
|
| I multiplied by a -4 to take care of both the negativeand the divisionby 4 in one step. In line 2, note that when I didshow the stepof multiplying both sides by a -4, I reversed my inequality sign. Interval notation:
We have an open interval since we are not including where it is equalto -20. x is less than -20,so -20 is our largest value of the interval, so it goes on theright. Since there is no lower endpoint (it is ALL values less than -20), weputthe negative infinity symbol on the left side. The curved end on-20 indicates an open interval. Negative infinity always has acurvedend because there is not an endpoint on that side. Graph:
We use the same type of notation on the endpoint as we did in theintervalnotation, a curved end. Since we needed to indicate allvaluesless than -20, the part of the number line that was to the left of -20was darkened. |
 Example5: Solve, write your answer in interval notation andgraphthe solution set: Example5: Solve, write your answer in interval notation andgraphthe solution set: 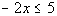 . . |
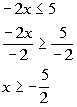 Interval notation:  Graph:
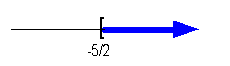 | *Inv. of mult. by -2 is div.by -2,
so reverse inequality sign
*Closed interval indicating allvalues greaterthan or = -5/2 *Visual showing all numbersgreater than or= -5/2 on the number line
|
| In line 2, note that when Idid show the stepof dividing both sides by a -2, that I reversed my inequality sign. Interval notation:
We have a closed interval since we are including where it is equalto -5/2. x is greater thanor equalto -5/2, so -5/2 is our smallest value of the interval so it goes ontheleft. Since there is no upper endpoint (it is ALL values greaterthan or equal to -5/2), we put the infinity symbol on the rightside. The boxed end on -5/2 indicates a closed interval. Infinityalwayshas a curved end because there is not an endpoint on that side. Graph:
We use the same type of notation on the endpoint as we did in theintervalnotation, a boxed end. Since we needed to indicateallvalues greater than or equal to -5/2, the part of the number line thatwas to the right of -5/2 was darkened. |
| Strategy for Solving a LinearInequality |
Step 1: Simplifyeach side if needed.
| This would involve things like removing ( ),removing fractions, addinglike terms, etc. | Step 2: Use Add./Sub.Properties to move the variable term on one side and all other terms tothe other side.
Step 3:Use Mult./Div.Properties to remove any values that are in front of the variable.
Note that it is the same basicconcept we usedwhen solving linear equations as shown in Tutorial14: Linear Equations in One Variable.
 Example6: Solve, write your answer in interval notation and graphthesolution set: Example6: Solve, write your answer in interval notation and graphthesolution set: 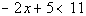 . .
|
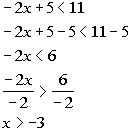 Interval notation: Graph:
 | *Inv. of add. 5 is sub. 5
*Inv. of mult. by -2 is div.both sides by-2, so reverse inequality sign
*Open interval indicating allvalues greaterthan -3
*Visual showing all numbersgreater than -3on the number line
|
Interval notation:
We have an open interval since we are not including where it is equalto -3. x is greater than -3,so-3 is our smallest value of the interval so it goes on the left. Since there is no upper endpoint (it is ALL values less than -3), weputthe infinity symbol on the right side. The curved end on -3indicatesan open interval. Infinity always has a curved end because thereis not an endpoint on that side. Graph:
We use the same type of notation on the endpoint as we did in theintervalnotation, a curved end. Since we needed to indicate allvaluesgreater than -3, the part of the number line that was to the right of-3was darkened. |
 Example7: Solve, write your answer in interval notation andgraphthe solution set: Example7: Solve, write your answer in interval notation andgraphthe solution set:  |
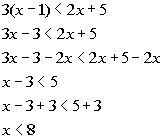 Interval notation: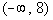 Graph:
 | *Distributive property
*Get xterms onone side, constants on the other side *Inv. of sub. 3 is add. by 3
*Open interval indicating allvalues less than-1/2
*Visual showing all numbersless than -1/2on the number line. |
Interval notation:
Again, we have an open interval since we are not including where itis equal to 8. This time xis less than 8, so 8 is our largest value of the interval so itgoeson the right. Since there is no lower endpoint (it is ALL valuesless than 8), we put the negative infinity symbol on the leftside. The curved end on 8 indicates an open interval. Negative infinityalways has a curved end because there is not an endpoint on that side. Graph:
Again, we use the same type of notation on the endpoint as we did inthe interval notation, a curved end. Since we needed to indicateall values less than 8, the part of the number line that was to theleftof 8 was darkened. |
 Example8: Solve, write your answer in interval notation andgraphthe solution set: Example8: Solve, write your answer in interval notation andgraphthe solution set: 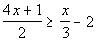 |
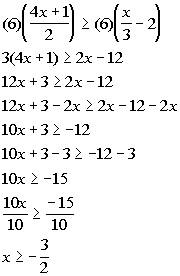 Interval notation: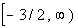 Graph:
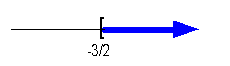 | *Mult. both sides by LCD of 6
*Get xterms onone side, constants on the other side
*Inv. of add. 3 is sub. by 3 *Inv. of mult. by 10 is div. by10
*Closed interval indicating allvalues greaterthan or equal to -3/2
*Visual showing all numbersgreater than orequal to -3/2 on the number line. |
Interval notation:
This time we have a closed interval since we are including where itis equal to -3/2. x isgreaterthan or equal to -3/2, so -3/2 is our smallest value of the interval soit goes on the left. Since there is no upper endpoint (it is ALLvalues greater than or equal to -3/2), we put the infinity symbol ontheright side. The boxed end on -3/2 indicates a closedinterval. Infinity always has a curved end because there is not an endpoint onthatside. Graph:
Again, we use the same type of notation on the endpoint as we did inthe interval notation, a boxed end this time. Since weneededto indicate all values greater than or equal to -3/2, the part of thenumberline that was to the right of -3/2 was darkened. |
| Solving a CompoundInequality |
| A compound linear inequality is one that has twoinequalities inone problem. For example, 5 < x+ 3 < 10 or -1 < 3x < 5. Yousolve them exactly the same way you solve thelinearinequalities shown above, except you do the steps to three"sides"(or parts) instead of only two. |
 Example9: Solve, write your answer in interval notation andgraphthe solution set: Example9: Solve, write your answer in interval notation andgraphthe solution set: 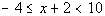 |
| This is an example of an compound inequality |
 Interval notation: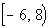 Graph:
 | *Inv. of add. 2 is sub. by 2
*Apply steps to all threeparts
*All values between -6 and 8,with a closedinterval at -6 (including -6)
*Visual showing all numbersbetween -6 and8, including -6 on the number line. |
Interval notation:
This time we have a mixed interval since we are including where itis equal to -6, but not equal to 8. xis between -6 and 8, including -6, so -6 is our smallest value of theintervalso it goes on the left and 8 goes on the right. The boxedendon -6 indicates a closed interval on that side. A curved end on 8indicatesan open interval on that side. Graph:
Again, we use the same type of notation on the endpoints as we didin the interval notation, a boxed end on the left and a curvedendon the right. Since we needed to indicate all values between -6and8, including -6, the part of the number line that is in between -6 and8 was darkened. |
| Solving an Absolute ValueInequality |
| Step 1: Isolatethe absolute value expression. |
| If there is a constant that is on the same side of theinequality thatthe absolute value expression is but is not inside the absolute value,use inverse operations to isolate the absolute value. |
| Step 2: Use thedefinition of absolute value to set up the inequality without absolutevalues. |
| A quick reminder, the absolute value measures theDISTANCE a numberis away from the origin (zero) on the number line. No matter ifthenumber is to the left (negative) or right (positive) of zero on thenumberline, the DISTANCE it is away from zero is going to be positive. Hence,the absolute value is always positive, (or zero if you are taking theabsolutevalue of 0). If you need a review on absolute values, feel free to . |
| If d is POSITIVEand |x|< d, then -d < x < d The graph below illustrates all the values on the numberline whosedistance would be less than d units awayfrom0. It shows us why we set up the inequality, shown above, the waywe do.
| If d is NEGATIVEand |x|< d, then there is no solution The absolute value is always positive, and any positivenumber is greaterthan any negative number, therefore it would be no solution. |
| If d isPOSITIVE and|x| > d, then x < -d OR x > d The graph, shown below, illustrates all the values onthe number linewhose distance would be greater than dunitsaway from 0. It shows us why we set up the inequality, shownabove,the way we do.
| If d is NEGATIVEand |x|> d, then x is all real numbers The absolute value is always positive, and any positivenumber is greaterthan any negative number, therefore all real numbers would work. |
| Step 3: Solvethe linear inequalities set up in step 2. |
| You will solve these linear inequalities just like theones shown above. |
 Example10: Solve, write your answer in interval notation andgraph the solution set: Example10: Solve, write your answer in interval notation andgraph the solution set: 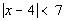 . . |
| Step 1: Isolatethe absolute value expression. |
| The absolute value expression is already isolated. |
| Step 2: Use the definitionof absolute value to set up the inequality without absolute values. AND Step 3: Solvethe linear inequalities set up instep2. |
| The distance that the expression x- 4is away from the origin needs to be less than 7. 
All numbers between -7 and 7 are less than 7 units awayfrom the origin. So, the expression x - 4 needs to bebetween-7 and 7. |
 Interval notation: Graph:
 | *Inv. of sub. 4 is add. by 4
*Apply steps to all three parts
*All values between -3 and 11
*Visual showing all numbersbetween -3 and11
|
Interval notation:
This time we have an open interval since we are not including eitherendpoint. x is between -3 and 11,so-3 is our smallest value of the interval so it goes on the left and 11goes on the right. The curved end on both numbers indicatean open interval on both sides. Graph:
Again, we use the same type of notation on the endpoints as we didin the interval notation, a curved end on both ends. Sincewe needed to indicate all values between -3 and 11, the part ofthenumber line that is in between -3 and 11 was darkened. |
 Example11: Solve, write your answer in interval notation andgraph the solution set: Example11: Solve, write your answer in interval notation andgraph the solution set: 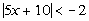 . . |
| Step 1: Isolatethe absolute value expression. |
| The absolute value expression is already isolated. |
| Step 2: Use the definitionof absolute value to set up the inequality without absolute values. AND Step 3: Solvethe linear inequalities set up instep2. |
| Be careful, since the absolute value (the left side) isalways positive,and positive values are always greater than negative values, the answeris no solution. There is no value that we can put in for xthat would make this inequality true. |
 Example12: Solve, write your answer in interval notation andgraph the solution set: Example12: Solve, write your answer in interval notation andgraph the solution set:  |
| Step 1: Isolatethe absolute value expression. |
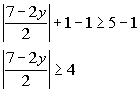 |
*Inv. of add. 1 is sub. 1 *Abs. value exp. isolated
|
| Step 2: Use the definitionof absolute value to set up the inequality without absolute values. AND Step 3: Solvethe linear inequalities set up instep2. |
| The distance that the expression (7- 2y)/2is away from the origin needs to be greater than or equal to 4. 
All numbers that are less than or equal to - 4 ORgreater than or equalto 4 are greater than or equal to 4 units away from the origin. Sothe expression (7 - 2y)/2 needs to belessthan or equal to - 4 OR greater than or equal to 4. |
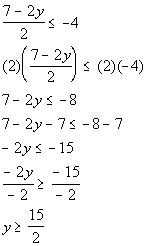 OR
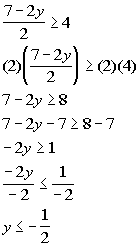
Interval notation: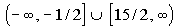 Graph:
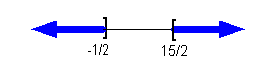 | *First inequality, where it isless than or= to -4 *Inv. of div. by 2 is mult. by 2
*Inv. of mult. by -2 is div. by-2,
so reverse inequality sign
*Second inequality, where it isgreater thanor = to 4 *Inv. of div. by 2 is mult. by 2
*Inv. of mult. by -2 is div. by-2,
so reverse inequality signs
*All values less than or = to-1/2 or greaterthan or = to 15/2 *Visual showing all numbersless than or =to -1/2 or greater than or = to 15/2
|
Interval notation:
This time we have two closed intervals since we are including theendpoints-1/2 and 15/2. In the first interval, yis less than orequal to -1/2, so -1/2 is our largest value of the interval so it goeson the right. Since there is no lower endpoint of that firstinterval,we put negative infinity on the left side. The boxed end on -1/2indicates a closed interval. Infinity always has a curved endbecausethere is not an endpoint on that side. In the second, interval, yis greaterthan or equal to 15/2, so 15/2 is our smallest value of the interval soit goes on the left. Since there is no upper endpoint of thatsecondinterval, we put the infinity symbol on the right side. The boxedend on 15/2 indicates a closed interval. Infinity always has acurvedend because there is not an endpoint on that side. Graph:
Again, we use the same type of notation on the endpoints as we didin the interval notation, a boxed end on both y= -1/2 and y = 15/2. Since weneededto indicate all values less than or equal to -1/2 OR greater than orequalto 15/2, the parts of the number line that are to the left of -1/2 andto the right of 15/2 were darkened. |
 Example13: Solve, write your answer in interval notation andgraph the solution set: Example13: Solve, write your answer in interval notation andgraph the solution set: 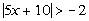 |
| Step 1: Isolatethe absolute value expression. |
| The absolute value expression is already isolated. |
| Step 2: Use the definitionof absolute value to set up the inequality without absolute values. AND Step 3: Solvethe linear inequalities set up instep2. |
| Again, be careful - since the absolute value (the leftside) is alwayspositive, and positive values are always greater than negative values, theanswer is all real numbers. No matter what value you plug infor x, when you take the absolute valuetheleft side will be positive. All positive numbers are greater than-2. |
 Practice Problems Practice Problems |
| These are practice problems to help bring you to thenext level. It will allow you to check and see if you have an understanding ofthesetypes of problems. Math works just likeanythingelse, if you want to get good at it, then you need to practiceit. Even the best athletes and musicians had help along the way and lots ofpractice, practice, practice, to get good at their sport or instrument. In fact there is no such thing as too much practice. To get the most out of these, you should work theproblem out onyour own and then check your answer by clicking on the link for theanswer/discussionfor that problem. At the link you will find the answeras well as any steps that went into finding that answer. |
 PracticeProblems 1a - 1c: PracticeProblems 1a - 1c: Solve, write your answer in intervalnotation and graphthe solution set. |
1a. 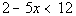
(answer/discussionto 1a) | 1b. 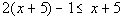
(answer/discussionto 1b) |
1c. 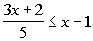
(answer/discussionto 1c) |
 PracticeProblems 2a - 2d: PracticeProblems 2a - 2d: Solve, write your answer in intervalnotation and graphthe solution set. |
2a. 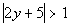
(answer/discussionto 2a) | 2b. 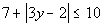
(answer/discussionto 2b) |
2c. 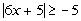
(answer/discussionto 2c) | 2d. 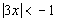
(answer/discussionto 2d) |
 Need Extra Help on These Topics? Need Extra Help on These Topics? |
The following are webpagesthat can assistyou in the topics that were covered on this page:
int_alg_tut10_linineq.htm
This website helps you with linear inequalities.
This website helps you with linear inequalities.
This website helps you with linear inequalities. |
forsomemore suggestions. |
All contents
June 22, 2003 |
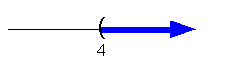
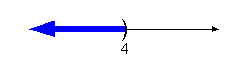

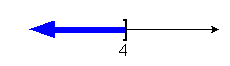

 Example1: Solve, write your answer in interval notation andgraphthe solution set:
Example1: Solve, write your answer in interval notation andgraphthe solution set: