Mathematics Content Expectations

Form A: Math Alignment Table
Alignment to Math High School Content Expectations
Math High School Content Expectations	Prealgebra Math 050 to Summer 2006	Prealgebra Math 050 to Fall 2006	Introductory Algebra Math 107 Summer and Fall 2006	Math 112	ACCUPLACER Tests
STANDARD S1: UNIVARIATE DATA – EXAMINING DISTRIBUTIONS Students plot and analyze univariate data by considering the shape of distributions and analyzing outliers; they find and interpret commonly -used measures of center and variation; and they explain and use properties of the normal distribution.
S1.1 Producing and Interpreting Plots
S1.1.1 Construct and interpret dot plots, histograms, relative frequency histograms, bar graphs, basic control charts, and box plots with appropriate labels and scales; determine which kinds of plots are appropriate for different types of data; compare data sets and interpret differences based on graphs and summary statistics.
S1.1.2 Given a distribution of a variable in a data set, describe its shape, including symmetry or skewness, and state how the shape is related to measures of center (mean and median) and measures of variation (range and standard deviation) with particular attention to the effects of outliers on these measures.
S1.2 Measures of Center and Variation
S1.2.1 Calculate and interpret measures of center including: mean, median, and mode; explain uses, advantages and disadvantages of each measure given a particular set of data and its context.
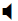S1.2.2 Estimate the position of the mean, median, and mode in both symmetrical and skewed distributions, and from a frequency distribution or histogram.
S1.2.3 Compute and interpret measures of variation, including percentiles, quartiles, interquartile range, variance, and standard deviation.
S1.3 The Normal Distribution
S1.3.1 Explain the concept of distribution and the relationship between summary statistics for a data set and parameters of a distribution.
S1.3.2 Describe characteristics of the normal distribution, including its shape and the relationships among its mean, median, and mode.
S1.3.3 Know and use the fact that about 68%, 95%, and 99.7% of the data lie within one, two, and three standard deviations of the mean, respectively in a normal distribution.
S1.3.4 Calculate z-scores, use z-scores to recognize outliers, and use z-scores to make informed decisions.
STANDARD S2: BIVARIATE DATA – EXAMINING RELATIONSHIPS Students plot and interpret bivariate data by constructing scatterplots, recognizing linear and nonlinear patterns, and interpreting correlation coefficients; they fit and interpret regression models, using technology as appropriate.
S2.1 Scatterplots and Correlation
S2.1.1 Construct a scatterplot for a bivariate data set with appropriate labels and scales.
S2.1.2 Given a scatterplot, identify patterns, clusters, and outliers; recognize no correlation, weak correlation, and strong correlation.
S2.1.3 Estimate and interpret Pearson’s correlation coefficient for a scatterplot of a bivariate data set; recognize that correlation measures the strength of linear association .
S2.1.4 Differentiate between correlation and causation; know that a strong correlation does not imply a cause-and-effect relationship; recognize the role of lurking variables in correlation.
S2.2 Linear Regression
S2.2.1 For bivariate data which appear to form a linear pattern, find the least squares regression line by estimating visually and by calculating the equation of the regression line; interpret the slope of the equation for a regression line.
S2.2.2 Use the equation of the least squares regression line to make appropriate predictions.
STANDARD S3: SAMPLES, SURVEYS, AND EXPERIMENTS Students understand and apply sampling and various sampling methods, examine surveys and experiments, identify bias in methods of conducting surveys, and learn strategies to minimize bias. They understand basic principles of good experimental design.
S3.1 Data Collection and Analysis
S3.1.1 Know the meanings of a sample from a population and a census of a population, and distinguish between sample statistics and population parameters.
S3.1.2 Identify possible sources of bias in data collection and sampling methods and simple experiments; describe how such bias can be reduced and controlled by random sampling; explain the impact of such bias on conclusions made from analysis of the data; and know the effect of replication on the precision of estimates .
S3.1.3 Distinguish between an observational study and an experimental study, and identify, in context, the conclusions that can be drawn from each .
STANDARD S4: PROBABILITY MODELS AND PROBABILITY CALCULATION Students understand probability and find probabilities in various situations, including those involving compound events , using diagrams, tables, geometric models and counting strategies; they apply the concepts of probability to make decisions.
S4.1 Probability
S4.1.1 Understand and construct sample spaces in simple situations (e.g., tossing two coins, rolling two number cubes and summing the results).
S4.1.2 Define mutually exclusive events, independent events, dependent events, compound events, complementary events and conditional probabilities; and use the to compute probabilities.
S4.2 Application and Representation
S4.2.1 Compute probabilities of events using tree diagrams, formulas for combinations and permutations, Venn diagrams, or other counting techniques.
S4.2.2 Apply probability concepts to practical situations, in such settings as finance, health, ecology, or epidemiology, to make informed decisions.
RECOMMENDED:
*S3.1.4 Design simple experiments or investigations to collect data to answer questions of interest; interpret and present results.
*S3.1.5 Understand methods of sampling, including random sampling, stratified sampling, and convenience samples, and be able to determine, in context, the advantages and disadvantages of each.
*S3.1.6 Explain the importance of randomization, double-blind protocols, replication, and the placebo effect in designing experiments and interpreting the results of studies.
*S3.2.1 Explain the basic ideas of statistical process control, including recording data from a process over time.
*S3.2.2 Read and interpret basic control charts; detect patterns and departures from patterns.
*S4.1.3 Design and carry out an appropriate simulation using random digits to estimate answers to questions about probability; estimate probabilities using results of a simulation; compare results of simulations to theoretical probabilities.
11/15/2006 bls BUSINESS and ED