Intermediate Algebra
Tutorial 30:
Solving By Factoring
 Learning Objectives
Learning Objectives
|
After completing this tutorial, you should be able to:
-
Write a polynomial equation in standard form.
-
Use the zero factor property.
-
Solve polynomial equations by factoring.
|
 Introduction
Introduction
|
| In this tutorial we will be putting our factoring skills to the test.
We will be looking at solving polynomial equations, which include quadratic
equations, by factoring. After
completing this tutorial, you will be a master at solving polynomial equations,
unlike the ones in this Close to Home comic. Solving equations
in general is a very essential part of Algebra. So I guess we better
get to it. |
 Tutorial
Tutorial
|
| A polynomial equation is one polynomial set equal to another polynomial.
The following is an example of a polynomial equation:
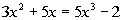
|
|
Standard Form of a Polynomial
Equation
|
| When a polynomial is set equal to 0, it is in standard form.
The following is an example of a polynomial equation in standard form:
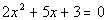
|
|
Quadratic Equation in Standard
Form
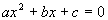
where a does not equal
0.
|
| The degree of a quadratic equation is 2. This is a common type
of equation. |
|
Zero Factor Property
If ab = 0, then a
= 0 or b = 0.
|
| 0 is our magic number because the only way a product can become
0 is if at least one of its factors is 0.
You can not guarantee what the factors would have to be if the product
was set equal to any other number. For example if ab
= 1, then a = 5 and b =
1/5 or a = 3 and b =
1/3, etc. But with the product set equal to 0, we can guarantee finding
the solution by setting each factor equal to 0. |
|
Solving a Polynomial Equation
by Factoring
|
| Step 1: Simplify if needed. |
| Clear any fractions or ( ) that are not in standard form
in the problem. |
| Step 2: Write in standard form if needed . |
| If needed, use inverse operations to get the polynomial set equal to
0. |
| Step 3: Factor the Polynomial. |
| Use the factoring
strategy shown in Tutorial 29: Factoring Special Products to factor
the polynomial completely. |
| Step 4: Use the Zero Factor Property. |
| Set every factor equal to zero. |
| Step 5: Solve for the equations set up in step 4. |
 Example
1: Solve the equation Example
1: Solve the equation 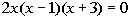 . . |
| Step 1: Simplify if needed. |
| In this problem, there is no simplifying that we can do. Even
though we have ( ), the problem is already in standard form, so we do not
need to multiply it out. |
| Step 2: Write in standard form if needed . |
| This problem is already written in standard form. |
| Step 3: Factor the Polynomial. |
| This problem is already factored. |
| Step 4: Use the Zero Factor Property
AND |
| Step 5: Solve for the equations set up in step 4. |
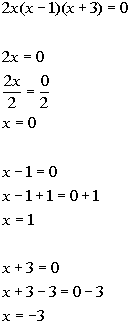 |
*Set 1st factor = 0
*Solve for
x
*Set 2nd factor = 0
*Solve for
x
*Set 3rd factor = 0
*Solve for
x |
| The solutions to this equation are 0, 1, and -3.
Note that if we plug any one of these three numbers into the original
equation, they would make the left side equal to the right side. |
 Example
2: Solve the equation Example
2: Solve the equation  . . |
| Step 1: Simplify if needed. |
| In this problem, there is no simplifying that we can do. Even
though we have ( ), the problem is already in standard form, so we do not
need to multiply it out. |
| Step 2: Write in standard form if needed. |
| This problem is already written in standard form. |
| Step 3: Factor the Polynomial. |
| This problem is already factored. |
| Step 4: Use the Zero Factor Property
AND |
| Step 5: Solve for the equations set up in step 4. |
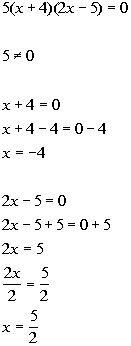 |
*5 can never equal 0
*Set 2nd factor = 0
*Solve for
x
*Set 3rd factor = 0
*Solve for
x
|
| Note that one of our factors is a constant 5. A non-zero constant
will never equal 0, so that factor does not give us a solution.
The solutions to this equation are - 4 and 5/2.
Note that if we plug either of these numbers into the original equation,
they would make the left side equal to the right side. |
 Example
3: Solve the equation Example
3: Solve the equation 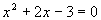 . . |
| Step 1: Simplify if needed. |
| In this problem, there is no simplifying that we can do. |
| Step 2: Write in standard form if needed. |
| This problem is already written in standard form. |
| Step 3: Factor the Polynomial. |
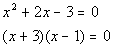 |
*Factor
the trinomial |
| Step 4: Use the Zero Factor Property
AND |
| Step 5: Solve for the equations set up in step 4. |
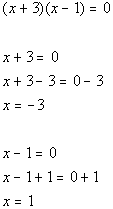 |
*Set 1st factor = 0
*Solve for
x
*Set 2nd factor = 0
*Solve for
x
|
| The solutions to this equation are -3 and 1.
Note that if we plug either of these numbers into the original equation,
they would make the left side equal to the right side. |
 Example
4: Solve the equation Example
4: Solve the equation 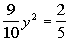 . . |
| Step 1: Simplify if needed. |
| We can simplify this problem by multiplying both sides of the equation
by the LCD. This will clear out the fractions and make it a nicer
problem to work with. |
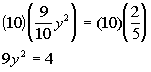 |
*Multiply both sides by LCD 10 |
| Step 2: Write in standard form if needed. |
| This problem is not written in standard form. We need to move
the 4 over to the other side of this equation so we have the polynomial
set equal to 0. |
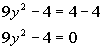 |
*Get polynomial = 0 |
| Step 3: Factor the Polynomial. |
 |
*Factor
the difference of squares |
| Step 4: Use the Zero Factor Property
AND |
| Step 5: Solve for the equations set up in step 4. |
 |
*Set 1st factor = 0
*Solve for
y
*Set 2nd factor = 0
*Solve for
y
|
| The solutions to this equation are -2/3 and 2/3.
Note that if we plug either of these numbers into the original equation,
they would make the left side equal to the right side. |
 Example
5: Solve the equation Example
5: Solve the equation 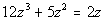 . . |
| Step 1: Simplify if needed. |
| In this problem, there is no simplifying to do. |
| Step 2: Write in standard form if needed. |
| This problem is not written in standard form. We need to move
the 2z over to the other side of this equation
so we have the polynomial set equal to 0. |
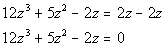 |
*Get polynomial = 0 |
| Step 3: Factor the Polynomial. |
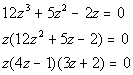 |
*Factor
a GCF out
*Factor
the trinomial |
| Step 4: Use the Zero Factor Property
AND |
| Step 5: Solve for the equations set up in step 4. |
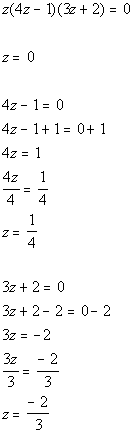 |
*Set 1st factor = 0
*Set 2nd factor = 0
*Solve for
z
*Set 3rd factor = 0
*Solve for
z
|
| The solutions to this equation are 1/4 and -2/3.
Note that if we plug any of these numbers into the original equation,
they would make the left side equal to the right side. |
 Example
6: Solve the equation Example
6: Solve the equation 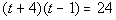 . . |
| Step 1: Simplify if needed. |
| On this problem, you need to be careful. Since it is set equal
to 24, it is NOT in standard form. So we need to multiply the left
side out and then get it into standard form. |
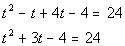 |
*Use the
FOIL method to multiply |
| Step 2: Write in standard form if needed. |
| This problem is not written in standard form. We need to move
the 24 over to the other side of this equation so we have the polynomial
set equal to 0. |
 |
*Get polynomial = 0 |
| Step 3: Factor the Polynomial. |
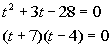 |
*Factor
the trinomial |
| Step 4: Use the Zero Factor Property
AND |
| Step 5: Solve for the equations set up in step 4. |
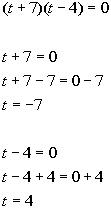 |
*Set 1st factor = 0
*Solve fort
*Set 2nd factor = 0
*Solve for
t
|
| The solutions to this equation are -7 and 4.
Note that if we plug either of these numbers into the original equation,
they would make the left side equal to the right side. |
 Practice Problems
Practice Problems
|
| These are practice problems to help bring you to the next level.
It will allow you to check and see if you have an understanding of these
types of problems. Math works just like anything
else, if you want to get good at it, then you need to practice it.
Even the best athletes and musicians had help along the way and lots of
practice, practice, practice, to get good at their sport or instrument.
In fact there is no such thing as too much practice.
To get the most out of these, you should work the problem out on
your own and then check your answer by clicking on the link for the answer/discussion
for that problem. At the link you will find the answer
as well as any steps that went into finding that answer. |
 Practice
Problems 1a - 1d: Practice
Problems 1a - 1d:
Solve each equation.
|
1a. 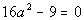
(answer/discussion
to 1a) |
1b. 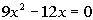
(answer/discussion
to 1b) |
1c. 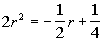
(answer/discussion
to 1c) |
1d. 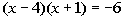
(answer/discussion
to 1d) |
 Need Extra Help on These Topics?
Need Extra Help on These Topics?
|
The following are webpages that can assist
you in the topics that were covered on this page:
sobyfactor.html
This webpage helps with solving quadratic equations by factoring.
This webpage helps with solving quadratic equations by factoring.
This webpage helps with solving quadratic equations by factoring.
This webpage helps with solving quadratic equations by factoring. |
for some
more suggestions. |
All contents
Jan. 8, 2002 |