College Algebra
Tutorial 46:
Logarithmic Equations
Learning Objectives
|
After completing this tutorial, you should be able to:
-
Solve logarithmic equations.
|
Introduction
|
| In this tutorial I will step you through how to solve equations that
have logarithmic expressions. In these equations, you will notice
that the variable that we are solving for is inside the log expressions.
We will rewrite the log equation exponentially using the definition of
logs to help us get the x outside of the log.
If you need a review on the definition of log functions, feel free to .
Ready, set, GO!!!!! |
Tutorial
|
|
Solving a Logarithmic Equation of the Form
|
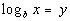
| Step 1: Write as
one log isolated on one side. |
| Get your log on one side everything outside of the log on the other
side of your equation using inverse operations.
Also use properties of logs to write it so that there is only one log.
If you need a review on properties of logs feel free to . |
| Step 2: Use
the definition of logarithms to write in exponential form. |
| Now that the variable is out of the log, solve for the variable using
inverse operations to complete the problem. |
 Example
1: Solve the logarithmic equation  .
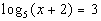Round your answer to two decimal places. |
| Step 1: Write
as one log isolated on one side. |
| This is already done for us in this problem. |
| Step 2: Use
the definition of logarithms to write in exponential form. |
 |
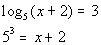*Rewrite in exponential form
*Base = 5 and exponent = 3 |
 |
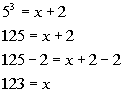*5 cubed is 125
*Solve for x |
Example
2: Solve the logarithmic equation  .
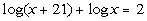Round your answer to two decimal places. |
| Step 1: Write
as one log isolated on one side. |
 |
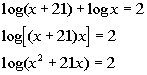*Use the product
rule to write as one log
*one log is isolated |
| Step 2: Use
the definition of logarithms to write in exponential form. |
 |
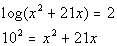*Rewrite in exponential form
*Base = 10 and exponent = 2 |
| Remember that when there is no base written on a log, that means it
is the common log, or log base 10. If you need a review on common
logs feel free to . |
 |
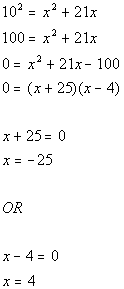*10 squared is 100
*Solve for x
*Factor
the trinomial
*Set 1st factor = 0
*Set 2nd factor = 0
|
Since -25 would create a negative number inside
both logs in this problem and we CANNOT take the log of a negative number,
we will have to throw out x = -25 as one of
our solutions.
Final answer: x = 4. |
Example
3: Solve the logarithmic equation  .
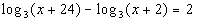Round your answer to two decimal places. |
| Step 1: Write
as one log isolated on one side. |
 |
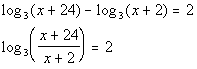*Use the quotient
rule to write as one log
*one log is isolated |
| Step 2: Use
the definition of logarithms to write in exponential form. |
 |
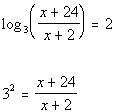*Rewrite in exponential form
*Base = 3 and exponent = 2 |
 |
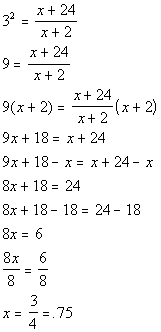*3 squared is 9
*Solve for x
*Multiply both sides by LCD of x
+ 2
|
| The equation that we had to solve in step 3 had a rational expression
in it. If you need a review on solving equations with rational expressions
feel free to |
Example
4: Solve the logarithmic equation  .
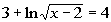Round your answer to two decimal places. |
| Step 1: Write
as one log isolated on one side. |
 |
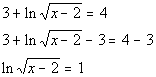*Inverse of add 3 is sub. 3
*one log is isolated |
| Step 2: Use
the definition of logarithms to write in exponential form. |
 |
*Rewrite in exponential form
*Base = e and
exponent = 1 |
| Remember that when you have ln, that means it is the natural log, or
log base e. If you need a review on natural
logs feel free to . |
 |
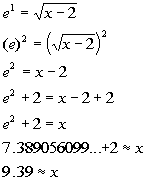*Square both sides to get rid of the radical
*Solve for x
*Use the calculator to find e
squared
|
| The equation that we had to solve in step 3 had a square root in it.
If you need a review on solving equations with radicals feel free to |
Practice Problems
|
| These are practice problems to help bring you to the next level.
It will allow you to check and see if you have an understanding of these
types of problems. Math works just like anything
else, if you want to get good at it, then you need to practice it.
Even the best athletes and musicians had help along the way and lots of
practice, practice, practice, to get good at their sport or instrument.
In fact there is no such thing as too much practice.
To get the most out of these, you should work the problem out on
your own and then check your answer by clicking on the link for the answer/discussion
for that problem. At the link you will find the answer
as well as any steps that went into finding that answer. |
 Practice
Problems 1a - 1d:
Solve the logarithmic equation. Round your
answer to two decimal places.
|
1a. 
(answer/discussion
to 1a) |
1b.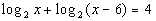 
(answer/discussion
to 1b) |
1c.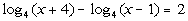 
(answer/discussion
to 1c) |
1d.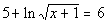 
(answer/discussion
to 1d) |
Need Extra Help on These Topics?
|
The following are webpages that can assist
you in the topics that were covered on this page:
This webpage will help you with solving logarithmic equations.
This webpage gives an example of solving a logarithmic equation. |
for some
more suggestions. |
All contents
November 5, 2002 |
0,
and is defined as 
.