College Algebra
Tutorial 4:
Radicals
 Learning Objectives Learning Objectives
|
After completing this tutorial, you should be able to:- Find the principal nth root of an expression.
- Find the nth root of an expression raised to the nth power.
- Simplify radical expressions.
- Multiply radicals that have the same index number.
- Divide radicals that have the same index number.
- Add and subtract like radicals.
- Rationalize one term denominators of rational expressions.
- Rationalize two term denominators of rational expressions.
|
 Introduction Introduction
|
| In this tutorial we will be looking at radicals (or roots). Basically,the root of an expression is the reverse of raising it to a power. For example, if you want the square root of an expression, then you wantanother expression, such that, when you square it, you get what is insidethe square root. This concept carries through to all roots. We willbe looking at rewriting, simplifying, adding, subtracting, multiplying,and rationalizing the denominator of radicals. You never knowwhen your radical simplifying skills may come in handy, so you want tobe prepared. |
 Tutorial Tutorial
|
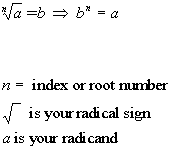 If n is even:
| If n is even, then aand b must be nonnegative for the root to bea real number. If n is even andaisnegative, then the root is not a real number. |
If n is odd:
| If n is odd, then a andbcan be any real number. |
|
Things to note about radicals in general:
When looking for the nth radical or nth root, you want the expressionthat, when you raise it to the nth power, you would get the radicand(what is inside the radical sign).
When there is no index number, n, itis understood to be a 2 or square root. For example: 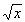 = principal squareroot of x. = principal squareroot of x.
Note that NOT EVERY RADICAL is a square root. If thereis an index number n other than the number2, then you have a root other than a square root. |
|
 Example1: Evaluate Example1: Evaluate  or indicate that the root is not a real number. or indicate that the root is not a real number. |
| The thought behind this is that we are looking for the square rootof 100. This means that we are looking for a number that when we squareit, we get 100. What do you think it is? Lets find out if you are right: 
Since 10 squared is 100, 10 is the square root of 100. Note that we are only interested in the principal root and since 100is positive and there is not a sign in front of the radical, our answeris positive 10. If there had been a negative in front of the radicalour answer would have been -10. |
 Example2: Evaluate Example2: Evaluate 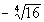 or indicate that the root is not a real number. or indicate that the root is not a real number. |
| Now we are looking for the negative of the fourth root of 16, whichmeans we are looking for a number that when we raise it to the fourth powerwe get 16 (then we will take its negative). What do you think it is? Lets find out if you are right: 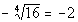
Since 2 raised to the fourth power is 16 and we are negating that, ouranswer is going to be -2. Note that the negative was on the outside of our even radical. If the negative had been on the inside of an even radical, then the answerwould be no real number. |
 Example3: Evaluate Example3: Evaluate 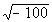 or indicate that the root is not a real number. or indicate that the root is not a real number. |
| Now we are looking for the square root of -100, which means we arelooking for a number that when we square it we get -100. What do you think it is? Lets find out if you are right: Since there is no such real number that when we square it we get -100,theanswer is not a real number. |
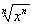 rule ruleIf n is an even positive integer, then 
If n is an odd positive integer, then 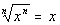 |
| If a problem does not indicate that a variable is positive, thenyou need to assume that we are dealing with both positive and negativereal numbers and use this rule. |
 Example4: Simplify Example4: Simplify 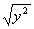 . . |
Since it didnt say that y is positive,we have to assume that it can be either positive or negative. Andsince the root number and exponent are equal, then we can use the 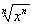 rule. rule.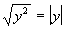
Since the root number and the exponent inside are equal and are theeven number 2, we need to put an absolute value around yfor our answer. The reason for the absolute value is that we do not know if yis positive or negative. So if we put yas our answer and it was negative, it would not be a true statement. For example if y was -5, then -5 squaredwould be 25 and the square root of 25 is 5, which is not the same as -5. The only time that you do not need the absolute value on a problem likethis is if it stated that the variable is positive. |
 Example5: Simplify Example5: Simplify  . . |
Since it didnt say that y is positive,we have to assume that it can be either positive or negative. Andsince the root number and exponent are equal, then we can use the 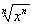 rule. rule.
This time our root number and exponent were both the odd number 3. When an odd numbered root and exponent match then the answer is the basewhether it is negative or positive. |
| Simplifying a Radical Expression |
| When you simplify a radical, you want to take out as much as possible. We can use the product rule of radicals (foundbelow) in reverse to help us simplify the nth root of a number that wecannot take the nth root of as is, but has a factor that we can take thenth root of. If there is such a factor, we write the radicand asthe product of that factor times the appropriate number and proceed. We can also use the quotient rule of radicals(found below) to simplify a fraction that we have under the radical. Note that the phrase "perfect square" meansthat you can take the square root of it. Just as "perfectcube" means we can take the cube root of the number, and so forth. I will be using that phrase in some of the following examples. |
A Product of Two Radicals
With the Same Index Number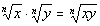
|
| In other words, when you are multiplying two radicals that havethe same index number, you can write the product under the same radicalwith the common index number. Note that if you have different index numbers, you CANNOT multiply themtogether. Also, note that you can use this rule in eitherdirection depending on what your problem is asking you to do. |
 Example6: Use the product rule to simplify Example6: Use the product rule to simplify 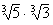 . . |
 | *Use the prod. rule of radicalsto rewrite |
| Note that both radicals have an index number of 3, so we were ableto put their product together under one radical keeping the 3 as its indexnumber. Since we cannot take the cube root of 15 and 15 does not have any factorswe can take the cube root of, this is as simplified as it gets. |
 Example7: Use the product rule to simplify Example7: Use the product rule to simplify 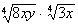 . . |
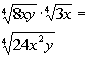 | *Use the prod. rule of radicalsto rewrite |
| Note that both radicals have an index number of 4, so we were ableto put their product together under one radical keeping the 4 as its indexnumber. Since we cannot take the fourth root of what is inside the radical signand 24 does not have any factors we can take the fourth root of, this isas simplified as it gets. |
 Example8: Use the product rule to simplify Example8: Use the product rule to simplify 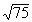 . . |
| Even though 75 is not a perfect square, it does have a factor thatwe can take the square root of. Check it out: |
 |
*Rewrite 75 as (25)(3)*Use the prod. rule of radicalsto rewrite
*The square root of 25 is 5 |
| In this example, we are using the product rule of radicals in reverseto help us simplify the square root of 75. When you simplify a radical,you want to take out as much as possible. The factor of 75 that wecan take the square root of is 25. We can write 75 as (25)(3) andthen use the product rule of radicals to separate the two numbers. We can take the square root of the 25 which is 5, but we will have to leavethe 3 under the square root. |
 Example9: Use the product rule to simplify Example9: Use the product rule to simplify 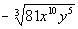 . . |
Even though 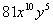 is not a perfect cube, it does have a factor that we can take the cuberoot of. is not a perfect cube, it does have a factor that we can take the cuberoot of.Check it out: |
In this example, we are using the product rule of radicals in reverseto help us simplify the cube root of 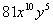 . When you simplify a radical, you want to take out as much as possible. The factor of . When you simplify a radical, you want to take out as much as possible. The factor of 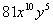 that we can take the cube root of is that we can take the cube root of is 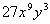 . We can write . We can write 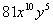 as as 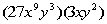 and then use the product rule of radicals to separate the two numbers. We can take the cube root of and then use the product rule of radicals to separate the two numbers. We can take the cube root of 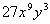 ,which is ,which is 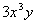 , butwe will have to leave the rest of it under the cube root. , butwe will have to leave the rest of it under the cube root. |
A Quotient of Two Radicals
With the Same Index NumberIf n is even, x and y representany nonnegative real number and y does not equal 0. If n is odd, x and y representany real number and y does not equal 0.

|
| This works in the same fashion as the rule for a product of two radicals. This rule can also work in either direction. |
 Example10: Use the quotient rule to simplify Example10: Use the quotient rule to simplify 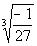 . . |
 | *Use the quotient ruleof radicals to rewrite
*The cube root of -1 is -1 and the cube rootof 27 is 3
|
 Example11: Use the quotient rule to simplify Example11: Use the quotient rule to simplify 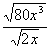 . . |
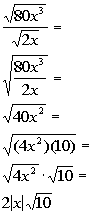 |
*Use the quotient ruleof radicals to rewrite *Simplify the fraction *Use the prod. rule of radicalsto rewrite *The square root of 4 x squared is 2|x|
|
| Since we cannot take the square root of 10 and 10 does not have anyfactors that we can take the square root of, this is as simplified as itgets. |
| Like radicals are radicals that have the same root number AND radicand(expression under the root). The following are two examples of two different pairs of like radicals: 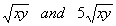
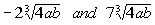
|
Adding and Subtracting
Radical Expressions |
| Step 1: Simplifythe radicals. |
Step 2: Combine likeradicals.
| You can only add or subtract radicals together if they are likeradicals. You add or subtract them in the same fashion that you do like terms. Combine the numbers that are in front of the like radicals and write thatnumber in front of the like radical part. |
|
 Example12: Add Example12: Add 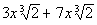 . . |
| Step 1: Simplifythe radicals. |
| Both radicals are as simplified as it gets. |
| Step 2: Combine likeradicals. |
| Note how both radicals are the cube root of 2. These two radicalsare like radicals. |
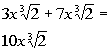 | *Combine like radicals: 3x+ 7x = 10x |
 Example13: Subtract Example13: Subtract  . . |
| Step 1: Simplifythe radicals. |
| The 75 in the second radical has a factor that we can take the squareroot of. Can you think of what that factor is? Lets see what we get when we simplify the second radical: |
 |
*Rewrite 75 as (25)(3)
*Use Prod. Rule of Radicals*Square root of 25 is 5 |
| The first radical is already in its simplest form. |
| Step 2: Combine likeradicals. |
 | *Combine like radicals: 4 - 30 = -26 |
Rationalizing the Denominator
(with one term) |
| When a radical contains an expression that is not a perfect root,for example, the square root of 3 or cube root of 5, it is calledan irrational number. So, in order to rationalize the denominator,we need to get rid of all radicals that are in the denominator. |
| Step 1: Multiply numeratorand denominator by a radical that will get rid of the radical in the denominator. |
| If the radical in the denominator is a square root, then you multiplyby a square root that will give you a perfect square under the radicalwhen multiplied by the denominator. If the radical in the denominatoris a cube root, then you multiply by a cube root that will give you a perfectcube under the radical when multiplied by the denominator and so forth... Note that the phrase "perfect square" meansthat you can take the square root of it. Just as "perfectcube" means we can take the cube root of the number, and soforth. Keep in mind that as long as you multiply the numerator and denominatorby the exact same thing, the fractions will be equivalent. |
| Step 2: Simplifythe radicals. |
| Step 3: Simplify thefraction if needed. |
| Be careful. You cannot cancel out a factor that is on the outsideof a radical with one that is on the inside of the radical. In orderto cancel out common factors, they have to be both inside the same radicalor be both outside the radical. |
 Example14: Rationalize the denominator Example14: Rationalize the denominator 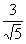 . . |
| Step 1: Multiply numeratorand denominator by a radical that will get rid of the radical in the denominator. |
| Since we have a square root in the denominator, then we need tomultiply by the square root of an expression that will give us a perfectsquare under the radical in the denominator. Square roots are nice to work with in this type of problem because ifthe radicand is not a perfect square to begin with, we just have to multiplyit by itself and then we have a perfect square. So in this case we can accomplish this by multiplying top and bottomby the square root of 5: |
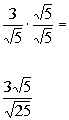 |
*Mult. num. and den. by sq. root of 5
*Den. now has a perfect square under sq. root
|
| Step 2: Simplifythe radicals. AND |
| Step 3: Simplify thefraction if needed. |
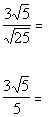 | *Sq. root of 25 is 5
|
| Be careful when you reduce a fraction like this. It isreal tempting to cancel the 5 which is on the outside of the radical withthe 5 which is inside the radical on the last fraction. You cannotdo that unless they are both inside the same radical or both outside theradical. |
 Example15: Rationalize the denominator Example15: Rationalize the denominator 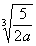 . . |
| Step 1: Multiply numeratorand denominator by a radical that will get rid of the radical in the denominator. |
Since we have a cube root in the denominator, we need to multiplyby the cube root of an expression that will give us a perfect cube underthe radical in the denominator.
So in this case, we can accomplish this by multiplying top and bottomby the cube root of 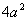 : : |
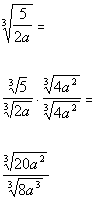 |
*Mult. num. and den. by cube root of 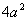
*Den. now has a perfect cube under cube root |
| Step 2: Simplifythe radicals. AND |
| Step 3: Simplify thefraction if needed. |
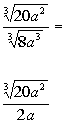 |
*Cube root of 8 acube is 2a |
| As discussed in example 14, we would not be able to cancel out the2 with the 20 in our final fraction because the 2 is on the outside ofthe radical and the 20 is on the inside of the radical. Also, we cannot take the cube root of anything under the radical. So, the answer we have is as simplified as we can get it. |
Rationalizing the Denominator
(with two terms) |
| Above we talked about rationalizing the denominator with one term. Again, rationalizing the denominator means to get rid of any radicals inthe denominator. Because we now have two terms, we are going to have to approach it differentlythan when we had one term, but the goal is still the same. |
| Step 1: Find the conjugateof the denominator. |
| You find the conjugate of a binomial by changing the sign that is betweenthe two terms, but keep the same order of the terms. a + band a - b are conjugatesof each other. |
| Step 2: Multiply thenumerator and the denominator of the fraction by the conjugate found inStep 1. |
| Keep in mind that as long as you multiply the numerator and denominatorby the exact same thing, the fractions will be equivalent. When you multiply conjugates together you get: 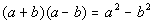
|
| Step 3: Simplifythe radicals. |
| Step 4: Simplify thefraction if needed. |
| Be careful. You cannot cancel out a factor that is on the outsideof a radical with one that is on the inside of the radical. In orderto cancel out common factors, they have to be both inside the same radicalor be both outside the radical. |
 Example16: Rationalize the denominator Example16: Rationalize the denominator 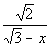 . . |
| Step 1: Find the conjugateof the denominator. |
| In general the conjugate of a + bisa- b and vice versa. So what would the conjugate of our denominator be? It looks like the conjugate is 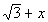 . . |
| Step 2: Multiply thenumerator and the denominator of the fraction by the conjugate found inStep 1. |
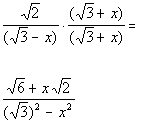 |
*Mult. num. and den. by conjugate of den.*Use distributive prop. to multiply the numerators
*In general, product of conjugates is 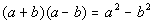 |
| Step 3: Simplifythe radicals. AND |
| Step 4: Simplify thefraction if needed. |
 |
*Square root of 3 squared is 3
|
 Example17: Rationalize the denominator Example17: Rationalize the denominator 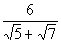 . . |
| Step 1: Find the conjugateof the denominator. |
| In general the conjugate of a + bisa- b and vice versa. So what would the conjugate of our denominator be? It looks like the conjugate is 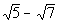 . . |
| Step 2: Multiply thenumerator and the denominator of the fraction by the conjugate found inStep 1. |
 |
*Mult. num. and den. by conjugate of den.*Use distributive prop. to multiply the numerators
*In general, product of conjugates is 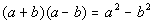 |
| Step 3: Simplifythe radicals. AND |
| Step 4: Simplify thefraction if needed. |
 | *Square root of 5 squared is 5
*Square root of 7 squared is 7
*Divide BOTH terms of num. by -2
|
 Practice Problems Practice Problems
|
| These are practice problems to help bring you to the next level. It will allow you to check and see if you have an understanding of thesetypes of problems. Math works just like anythingelse, if you want to get good at it, then you need to practice it. Even the best athletes and musicians had help along the way and lots ofpractice, practice, practice, to get good at their sport or instrument. In fact there is no such thing as too much practice. To get the most out of these, you should work the problem out onyour own and then check your answer by clicking on the link for the answer/discussionfor that problem. At the link you will find the answeras well as any steps that went into finding that answer. |
 PracticeProblems 1a - 1d: PracticeProblems 1a - 1d:Evaluate or indicate that the root is not a real number. |
1a. 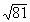
(answer/discussionto 1a) | 1b. 
(answer/discussionto 1b) |
1c. 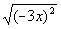
(answer/discussionto 1c) | 1d. 
(answer/discussionto 1d) |
 PracticeProblems 2a - 2b: PracticeProblems 2a - 2b:Use the product rule to simplify the expression. |
2a. 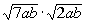
(answer/discussionto 2a) | 2b. 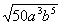
(answer/discussionto 2b) |
 PracticeProblems 3a - 3b: PracticeProblems 3a - 3b:Use the quotient rule to simplify the expression. |
3a. 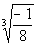
(answer/discussionto 3a) | 3b.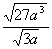
(answer/discussionto 3b) |
 PracticeProblems 4a - 4b: PracticeProblems 4a - 4b:Add or subtract. |
4a. 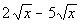
(answer/discussionto 4a) | 4b. 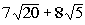
(answer/discussionto 4b) |
 PracticeProblems 5a - 5b: PracticeProblems 5a - 5b:Rationalize the denominator. |
5a. 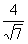
(answer/discussionto 5a) | 5b. 
(answer/discussionto 5b) |
 Need Extra Help on These Topics? Need Extra Help on These Topics?
|
The following are webpages that can assistyou in the topics that were covered on this page:
int_alg_tut37_radical.htm
This webpage goes through how to find the nth root of an expression.
int_alg_tut39_simrad.htm
This webpage covers how to multiply, divide, and simplify radical expressions.
radicals.htm
This webpage takes a look at the product and quotient rules for radicals.
int_alg_tut40_addrad.htm
This webpage helps you with adding and subtracting like radicals.
int_alg_tut41_rationalize.htm
This webpage explains how to rationalize denominators.
|
for somemore suggestions. |
All contents
June 16, 2002 |